The line AA' is on a charged infinite conducting plane which is perpendicular to the plane of the paper. The plane has surface density of charge σ and B is a ball of mass m with a like charge of magnitude q. B is connected by a string from a point on the line AA'. The tangent of the angle (θ) formed between the line AA' and the string is (provided, the charge q does not affect the distribution of charge on conducting plate)
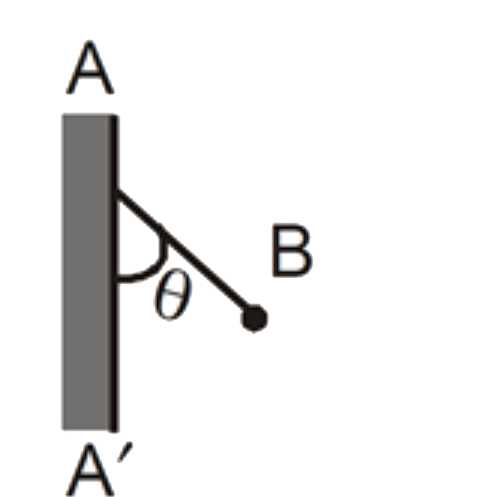
qσ2ε0mg
qσ4πε0mg
qσ2πε0mg
qσε0mg
The correct Answer is:D
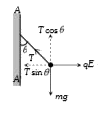
Tsinθ=qE
Tcosθ=mg
tanθ=qEmg=q.σε0mg [As for conductor E=σε0 ]
tanθ=qσε0mg
Topper's Solved these Questions
- BOOK - ERRORLESSCHAPTER - ELECTROSTATICSEXERCISE - NCERT BASED QUESTIONS (Electric Field and Potential)129videos
ELECTROSTATICS
- BOOK - ERRORLESSCHAPTER - ELECTROSTATICSEXERCISE - NCERT BASED QUESTIONS (Electric Dipole)21videos
ELECTROSTATICS
- BOOK - ERRORLESSCHAPTER - ELECTRONICSEXERCISE - Assertion & Reason26videos
ELECTRONICS
- BOOK - ERRORLESSCHAPTER - MAGNETIC EFFECTS OF CURRENT EXERCISE - ASSERTION & REASON18videos
MAGNETIC EFFECTS OF CURRENT
Similar Questions
Knowledge Check
- Question 1 - Select One
The line A A' is on charged infinite conducting plane which is perpendicular to the plane of the paper. The plane has a surface density of charge of magnitude q. B is connected by string from a point on the line A A'. The tangent of angle (θ) formed between the line A A' and the string is
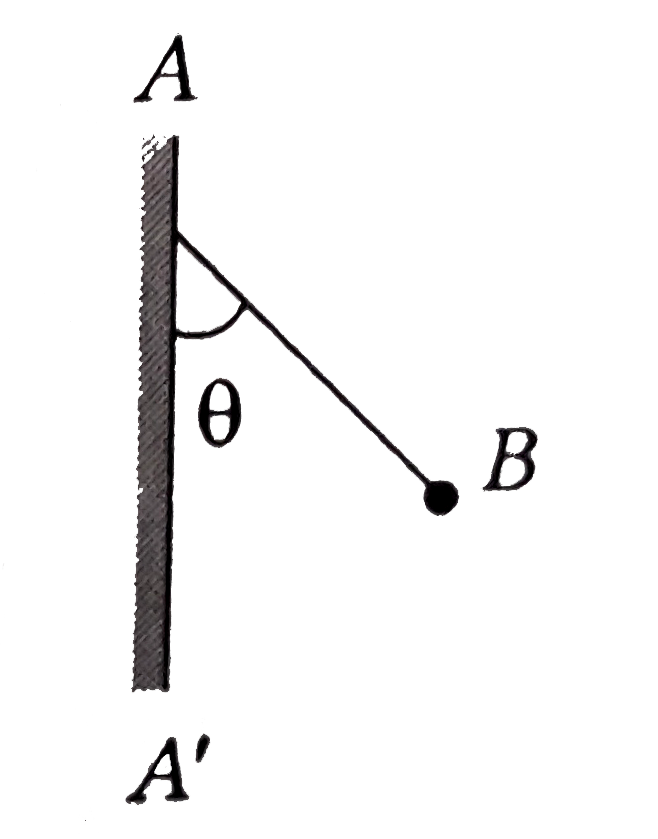 Aqσ2ε0mgBqσ4πε0mgCqσ2πε0mgDqσε0mg
Aqσ2ε0mgBqσ4πε0mgCqσ2πε0mgDqσε0mg - Question 2 - Select One
An infinite plane sheet of positive charge has surface charge density σ. A metallic ball of mass m and charge +Q is attached to a thred and is tied to a point A on the sheet PQ. The angle θ made by the string with plane sheet is in equilibrium is
AzeroBtan−1(Qσ4ε0mg)Ctan−1(Qσ2ε0mg)Dtan−1(Qσε0mg) - Question 3 - Select One
The line of greatest slope on an inclined plane P1 is the line in the plane P1 which is perpendicular to the line of intersection of the plane P1 and a horizontal planeP2.
Q. The coordinate of a point on the plane 2x+y−5z=0,2√11 unit away from the line of intersection of 2x+y−5z=0and4x−3y+7z=0 areAx3=y1=z−1Bx3=y−1=z1Cx−3=y1=z1Dx1=y3=z−1
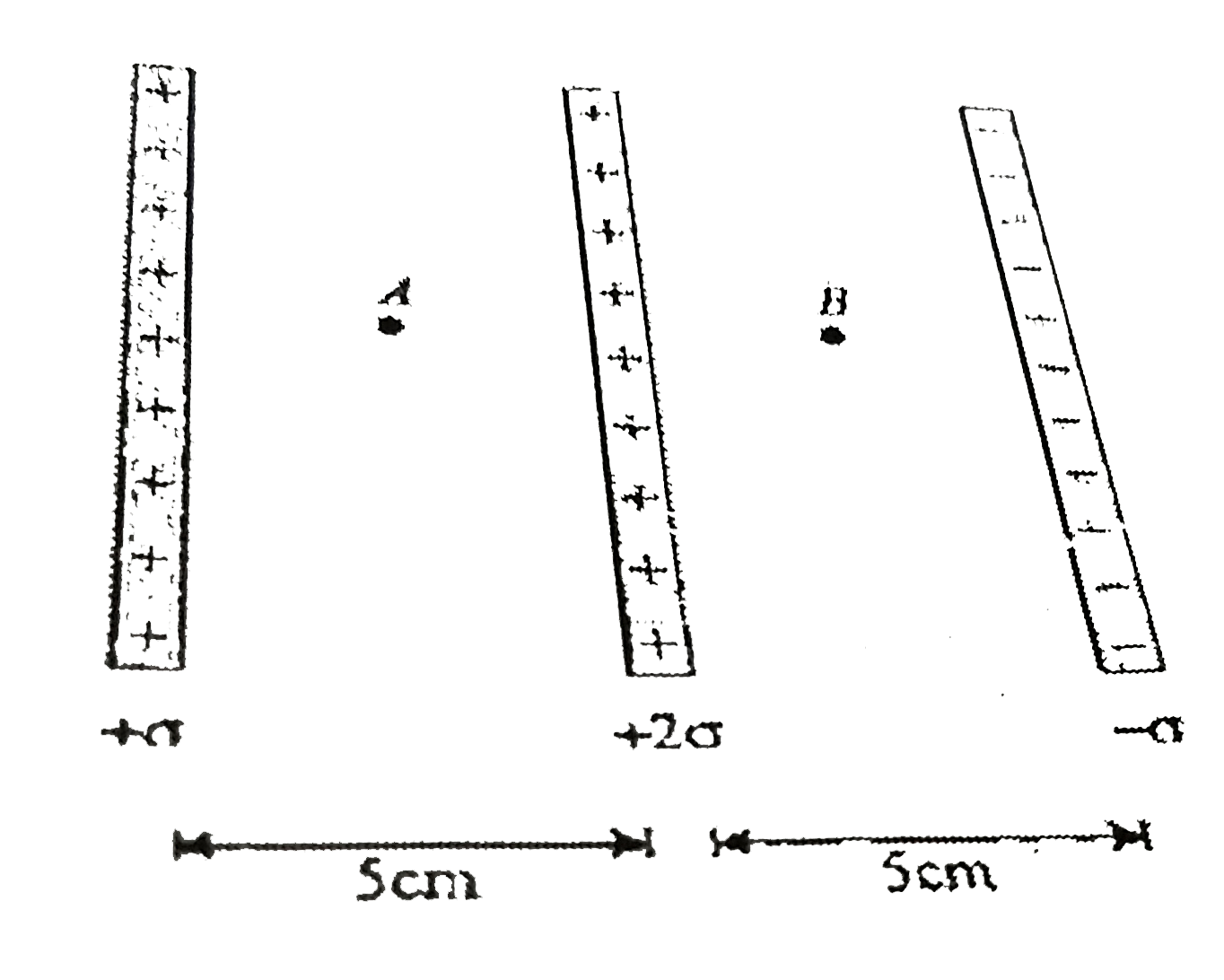
The figure shows three infinite non-conducting plates of charge perpendicular to the plane of the paper with charge per unit area +σ,+2σ and −σ. Find the ratio of the net electric field at that point a to that at point B.
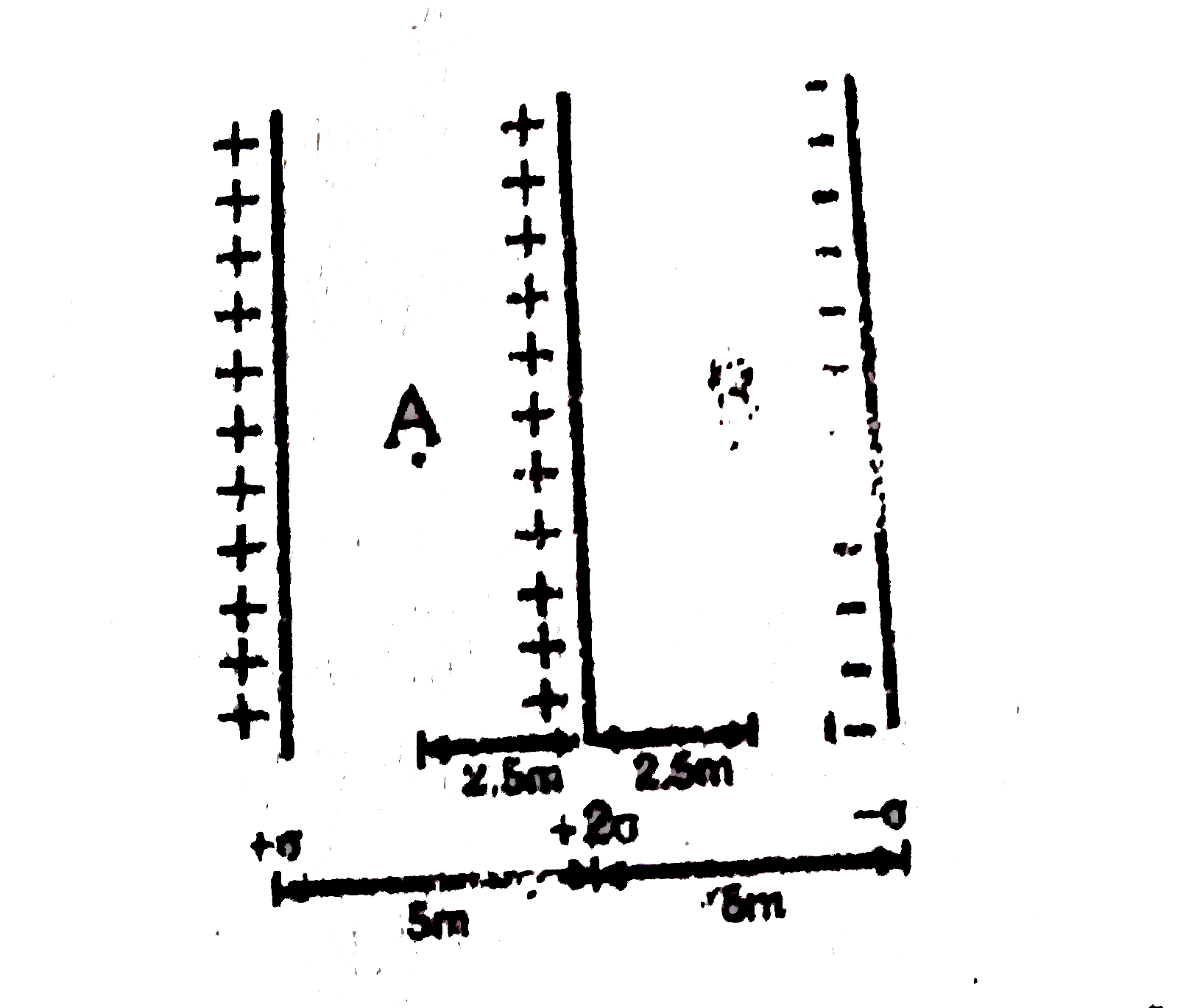 View Solution
View SolutionThe figure shows thrednfinite non-conducting plates of charge perpendicular to the plane of the paper with charge per unit area +σ,+2σ and −σ . Find the ratio of the net electric field atthat point A to that at point B. The points A and Bare located midway between the plates :
 View Solution
View SolutionA point charge q is located at a distance l from an infinite tonducting plane. Find the interaction energy of that charge with chose induced on the plane.
View SolutionA point charge +Q is placed in the vicinity of a conducting surface. Draw the eletric field lines between the surface and the charge
View SolutionElectric field at a point near a uniformaly charged infinite plane sheet having surface density of charge σ is given as E= ________________
View Solution
