A large number of particles are placed around the origin, each at a distance R from the origin. The distance of the center of mass of the system from the origin is
= R
≥R
>R
≤R
The correct Answer is:D
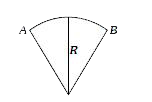
If arc AB → 0 centre of mass is at a distance R from the origin as the Arc length AB increases, centre of mass starts moving down.
Topper's Solved these Questions
- ROTATIONAL MOTIONBOOK - ERRORLESSCHAPTER - ROTATIONAL MOTIONEXERCISE - NCERT BASED QUESTIONS (Angular Displacement, Velocity and Acceleration)
34 Videos
- ROTATIONAL MOTIONBOOK - ERRORLESSCHAPTER - ROTATIONAL MOTIONEXERCISE - NCERT BASED QUESTIONS (Moment of Inertia)
139 Videos
- NEWTON'S LAWS OF MOTIONBOOK - ERRORLESSCHAPTER - NEWTON'S LAWS OF MOTIONEXERCISE - ASSERTION & REASON
18 Videos
- SIMPLE HARMONIC MOTIONBOOK - ERRORLESSCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Assertion & Reason
15 Videos
Similar Questions
All the particles of body are situated at a distance R from the origin. The distance of the centre of mass of the body from the origin is also R. Is this statement true or false?
View SolutionFor a group of particles of different masses,if all the masses are placed at different points at equal distance R from the origin, then the distance of the centre of mass from the origin will be
View Solution
Knowledge Check
All the particles of a body are situated at a distance R from the origin. The distance of the centre of mass of the body from the origin is
A=RB≤RC>RD≥RAll the particles of a body situated at distance d from the origin. The distance of the center of mass of the body from the origin is
A=dB≤dC>dD≥dThe distance of the point p(3,4) from the origin is
A3B4C7D5
All the particles are situated at a distance R from the origin. The distance of centre of mass of the body from the origin will be
(1) More than R
(2) Less than R
(3) Equal to R
(4) At the origin
View SolutionThe distance from the origin to the point (8,15)
View SolutionThe distance of the point P(2,4) from the origin is :
View SolutionThe distance of the points (8,6) from the origin is
View SolutionThe distance of the B(6,6) from the origin is:
View Solution
