The pressure at depth h below the surface of a liquid of density ρ open to the atmosphere is
Greater than the atmospheric pressure by ρgh
Less than the atmospheric pressure by ρgh
Equal to the atmospheric pressure
Decreases exponentially with depth
The correct Answer is:A
P=P0+ρgh
Topper's Solved these Questions
- SURFACE TENSIONBOOK - ERRORLESSCHAPTER - SURFACE TENSIONEXERCISE - NCERT BASED QUESTIONS (Capillarity)
18 Videos
- SURFACE TENSIONBOOK - ERRORLESSCHAPTER - SURFACE TENSIONEXERCISE - PAST YEARS QUESTIONS
12 Videos
- SURFACE TENSIONBOOK - ERRORLESSCHAPTER - SURFACE TENSIONEXERCISE - NCERT BASED QUESTIONS (Angle of Contact)
11 Videos
- SIMPLE HARMONIC MOTIONBOOK - ERRORLESSCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Assertion & Reason
15 Videos
- THERMODYNAMICSBOOK - ERRORLESSCHAPTER - THERMODYNAMICSEXERCISE - ASSERTION AND REASON
20 Videos
Similar Questions
Find the total pressure inside a sperical air bubble of radius 0.2 mm. The bubble is at a depth 5 cm below the surface of a liquid of density 2×103 kg m−3 and surface tension 0.082 N m−1. (Given : atmospheric pressure = 1.01 ×105Nm−2)
Hint : Pi=Po+2SR
Po = atmospheric pressure + gauge pressure of liquid column.
View SolutionAt what depth below the surface of water total pressure would be equal to 1.5 atmosphere ?
View Solution
Knowledge Check
If the atompspheric pressure is Pa then the jpressure P at depth a below the surface of a liquid of density ρ open to the atmosphere is
APa−ρgh2BPa−ρghCPa+ρghDP1+ρgh2The absolute pressure at a depth h below the surface of a liquid of density ρ is [Given Pa = atmospheric pressure, g = acceleration due to gravity]
AρghBPa+ρghCPa−ρghDPa2ρghThe pressure at a depth h in a liquid of density ρ is plotted on the Y-axis and the value of h on the X-axis the graph is a strainght line. The slope of the straight line is (g= acceleration due to gravity)
AρgB1ρgCρgDgρ
What will be the total pressure inside a spherical air bubble of radius 0.2 mm at a depth of 2m below the surface of a liquid of density 1.1g/cm3 and surface tension 50dyne/cm. Atmospheric pressure is 1.01×105Nm−2.
View SolutionA solid cube of side a and density ρ0 floats on the surface of a liquid of density ρ. If the cube is slightly pushed downward, then it oscillates simple harmonically with a period of
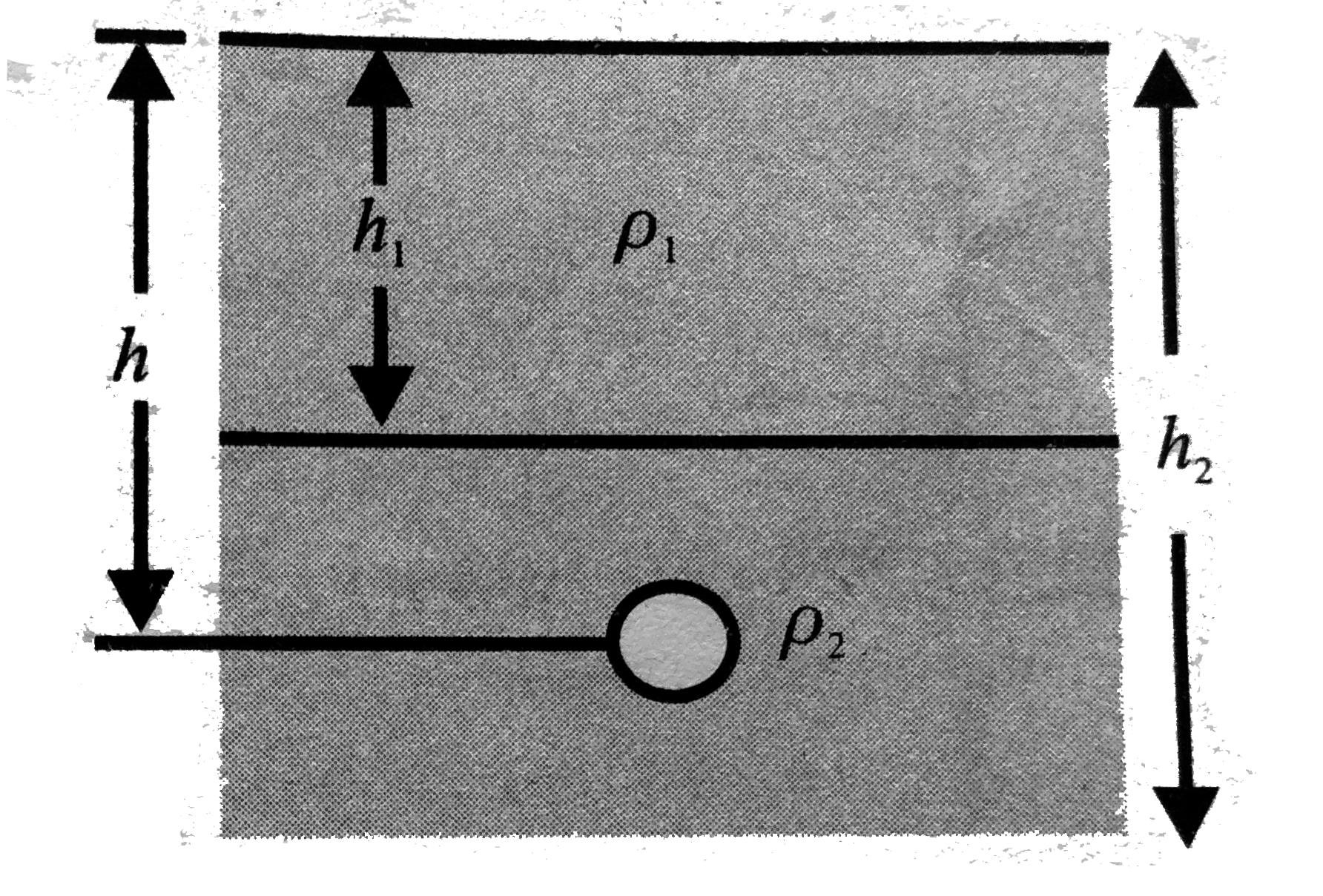
View SolutionCalculate the pressure inside a small air bubble of radus r situated at a depth h below the free surface of liquids of densities ρ1 and ρ2 and surface tennsions T1 and T2. The thickness of the first and second liquids are h1 and h2 respectively. Take atmosphere pressure =P0.
View SolutionThe pressure in a liquid at a given depth below the surface :
View SolutionA liquid of density ρ is filled in a vessel up to height H and a hole of cross section area A is made at a depth h below the free surface of liquid. The speed of liquid coming out of the hole is independent of
View Solution
