A particle moves in a circular orbit under the action of a central attractive force inversely proportional to the distance r. The speed of the particle is
Proportional to r2
Independent of r
Proportional to r
Proportional to 1/r
The correct Answer is:B
Let .F. be the force.
Given F∝α1r
=F=kr, where k is a constant.
Since, particle experiences centripetal force.
mv2r=kr
=v=√km (independent of r)
Topper's Solved these Questions
- MOTION IN TWO DIMENSIONBOOK - ERRORLESSCHAPTER - MOTION IN TWO DIMENSIONEXERCISE - NCERT BASED QUESTIONS (NON-UNIFORM CIRCULAR MOTION)
36 Videos
- MOTION IN TWO DIMENSIONBOOK - ERRORLESSCHAPTER - MOTION IN TWO DIMENSIONEXERCISE - NCERT BASED QUESTIONS (HORIZONTAL PROJECTILE MOTION)
11 Videos
- MOTION IN ONE DIMENSIONBOOK - ERRORLESSCHAPTER - MOTION IN ONE DIMENSIONEXERCISE - ASSERTION AND REASON
24 Videos
- NEWTON'S LAWS OF MOTIONBOOK - ERRORLESSCHAPTER - NEWTON'S LAWS OF MOTIONEXERCISE - ASSERTION & REASON
18 Videos
Similar Questions
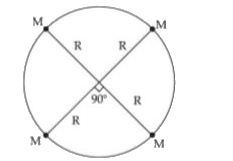
Four particles each of mass M, move along a circle of radius R under the action of their mutual gravitational attraction as shown in figure. The speed of each particle is :
View SolutionA particle revolves round a circular path. The acceleration of the particle is inversely proportional to-
View Solution
Knowledge Check
A particle moves in a circular orbit under the action of a central attractive force which is inversely proportional to the distance 'r' . The speed of the particle is
Aproportional to r2BIndependent of rCproportional to rDproportional to 1/rA particle moves in a circular orbit under the action of a central attractive force inversely proportional to the distance r. The speed of the particle is
AProportional to r2BIndependent of rCProportional to rDProportional to 1/rA particle executes circular motion under a central attractive force inversely proportional to distance R. The speed of the particle is
ADependent on RBDependent on R2CIndependent of RDDependent on 1/R
A particle is moving with a uniform speed in a circular orbit of radius R in a central force inversely proportional to the nth power of R. If the period of rotation of the particle is T, then :
View SolutionA particle revolves round a circular path. The acceleration of the particle is inversely proportional
View SolutionA particle revolves around a circular path. The centripetal acceleration of the particle is inversely proportional to
View SolutionA particle is moving in a circular path of radius r under the action of a force F. If at an instant velocity of particle is v, and speed of particle is increasing, then
View SolutionA particle is moving with uniform speed along the circumference of a circle of radius R under the action of a central fictitious force F which is inversely proportional to R3. its time period of revolution will be given by :
View Solution
