For a particle undergoing SHM, displacement from mean position is given according to the law : x(t)=(10cm)sin(2πt45), where t is in seconds. The mean position of particle is located at x=2cm. The ratio of KE and PE of particle when it is at x cm =−3cm is (Assume PE at mean position is zero)
2
0.33
0.5
3
The correct Answer is:D
Step by step video & text solution for For a particle undergoing SHM, displacement from mean position is given according to the law : x(t)=(10cm)sin((2pit)/45), where t is in seconds. The mean position of particle is located at x=2cm. The ratio of KE and PE of particle when it is at x cm =−3cm is (Assume PE at mean position is zero) by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Similar Questions
A particle executes SHM according to equation x=10(cm)cos[2πt+π2], where t is in seconds. The magnitude of the velocity of the particle at t=16s will be :-
View SolutionThe displacement of a particle from its mean position (in m) varies with time according to the relation y = 0.2sin(10πt+1.5π)cos(10πt+1.5π) . The motion of the particle is
View Solution
Knowledge Check
The velocity of a particle in simple harmonic motion at displacement y from mean position is
Aω√(a2+y2)Bω√(a2−y2)CwyDω2√(a2+y2)A particle is performing SHM according to the equation x=(3cm)sin(2π18+π6), where t is in seconds. The distance travelled by the particle in 39 s is
A24cmB1.5cmC25.5cmDnone of theseA particle is oscillating according to the equation x=10cos2πt, where 't' is in seconds. The particle moves from the position of maximum displacement to equilibrium in time.
A2 sec.B4 sec.C0.25 sec.D0.5 sec.
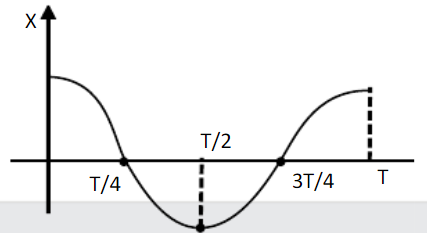
Displacement time graph of particle performing SHM is as shown in figure. Assume that mean position is at x=0
View SolutionThe equation of a wave is y=4sin{π2(2t+x8)} where y,x are in cm and time in seconds . The acceleration of particle located at x=8cm and t=1sec is
View SolutionThe position of a particle is given by
x=2(t−t2)
where t is expressed in seconds and x is in metre.
The acceleration of the particle is
View SolutionA particle is oscillating according to the equation x=8cosπt, where 't' is in second. The particle moves from the position of equilibrium to maximum displacement in time
View SolutionThe displacement of a particle from its mean position (in metre) is given by
y=0.2sin(10πt+1.5π)cos(10πt+0.5π).
The motion of particle is
View Solution