Two light aluminium rods of same length are suspended from a ceiling. First rod has load W hanging from it, while second rod has load 2W hanging from it. If the ratio of energy stored per unit volume of first rod to second rod is 1:64, then the ratio of diameter of first rod to second rod will be :
The correct Answer is:B
To solve the problem step by step, we will analyze the given situation involving two aluminum rods and the loads they carry.
Step 1: Understand the Problem
We have two aluminum rods of the same length. The first rod has a load W and the second rod has a load 2W. We need to find the ratio of the diameters of the two rods given that the ratio of energy stored per unit volume in the first rod to the second rod is 1:64.
Step 2: Define the Energy Stored per Unit Volume
The energy stored per unit volume U can be expressed as:
U=12×Stress×Strain
Step 3: Relate Stress and Strain
Stress (σ) is defined as the force per unit area:
σ=FA
For a circular cross-section, the area A is given by:
A=πD24
Thus, the stress for each rod can be written as:
σ1=WπD214=4WπD21
σ2=2WπD224=8WπD22
Step 4: Substitute Stress into Energy Equation
Now substituting stress into the energy stored per unit volume equation:
U1=12σ1ϵ1=12(4WπD21)ϵ1
U2=12σ2ϵ2=12(8WπD22)ϵ2
Step 5: Use the Given Ratio of Energy Stored
We know:
U1U2=164
Substituting the expressions for U1 and U2:
12(4WπD21)ϵ112(8WπD22)ϵ2=164
This simplifies to:
4Wϵ1D228Wϵ2D21=164
Canceling W and simplifying gives:
D22ϵ12D21ϵ2=164
Step 6: Relate Strain to Stress
Using the relationship γ=σϵ, we can express ϵ in terms of stress:
ϵ1=σ1γ1,ϵ2=σ2γ2
Assuming the material properties are the same for both rods, we can say γ1=γ2. Thus:
ϵ1=4WπD21γ,ϵ2=8WπD22γ
Step 7: Substitute Back into the Ratio
Substituting these into the ratio gives:
D22(4WπD21γ)2D21(8WπD22γ)=164
This simplifies to:
D42D41=132
Step 8: Solve for the Diameter Ratio
Taking the fourth root gives:
D2D1=12⟹D1D2=2
Final Answer
The ratio of the diameter of the first rod to the second rod is:
D1D2=2:1
We have two aluminum rods of the same length. The first rod has a load W and the second rod has a load 2W. We need to find the ratio of the diameters of the two rods given that the ratio of energy stored per unit volume in the first rod to the second rod is 1:64.
The energy stored per unit volume U can be expressed as:
U=12×Stress×Strain
Stress (σ) is defined as the force per unit area:
σ=FA
For a circular cross-section, the area A is given by:
A=πD24
Thus, the stress for each rod can be written as:
σ1=WπD214=4WπD21
σ2=2WπD224=8WπD22
Now substituting stress into the energy stored per unit volume equation:
U1=12σ1ϵ1=12(4WπD21)ϵ1
U2=12σ2ϵ2=12(8WπD22)ϵ2
We know:
U1U2=164
Substituting the expressions for U1 and U2:
12(4WπD21)ϵ112(8WπD22)ϵ2=164
This simplifies to:
4Wϵ1D228Wϵ2D21=164
Canceling W and simplifying gives:
D22ϵ12D21ϵ2=164
Using the relationship γ=σϵ, we can express ϵ in terms of stress:
ϵ1=σ1γ1,ϵ2=σ2γ2
Assuming the material properties are the same for both rods, we can say γ1=γ2. Thus:
ϵ1=4WπD21γ,ϵ2=8WπD22γ
Substituting these into the ratio gives:
D22(4WπD21γ)2D21(8WπD22γ)=164
This simplifies to:
D42D41=132
Taking the fourth root gives:
D2D1=12⟹D1D2=2
The ratio of the diameter of the first rod to the second rod is:
D1D2=2:1
Similar Questions
Two steel wires having same length are suspended from a ceiling under the same load. If the ratio of their energy stored per unit volume is 1:4 the ratio of their diameters is
View SolutionA uniform rod of length l is suspended from a point P. and the rod is made to undergo small oxcillations. Match the following
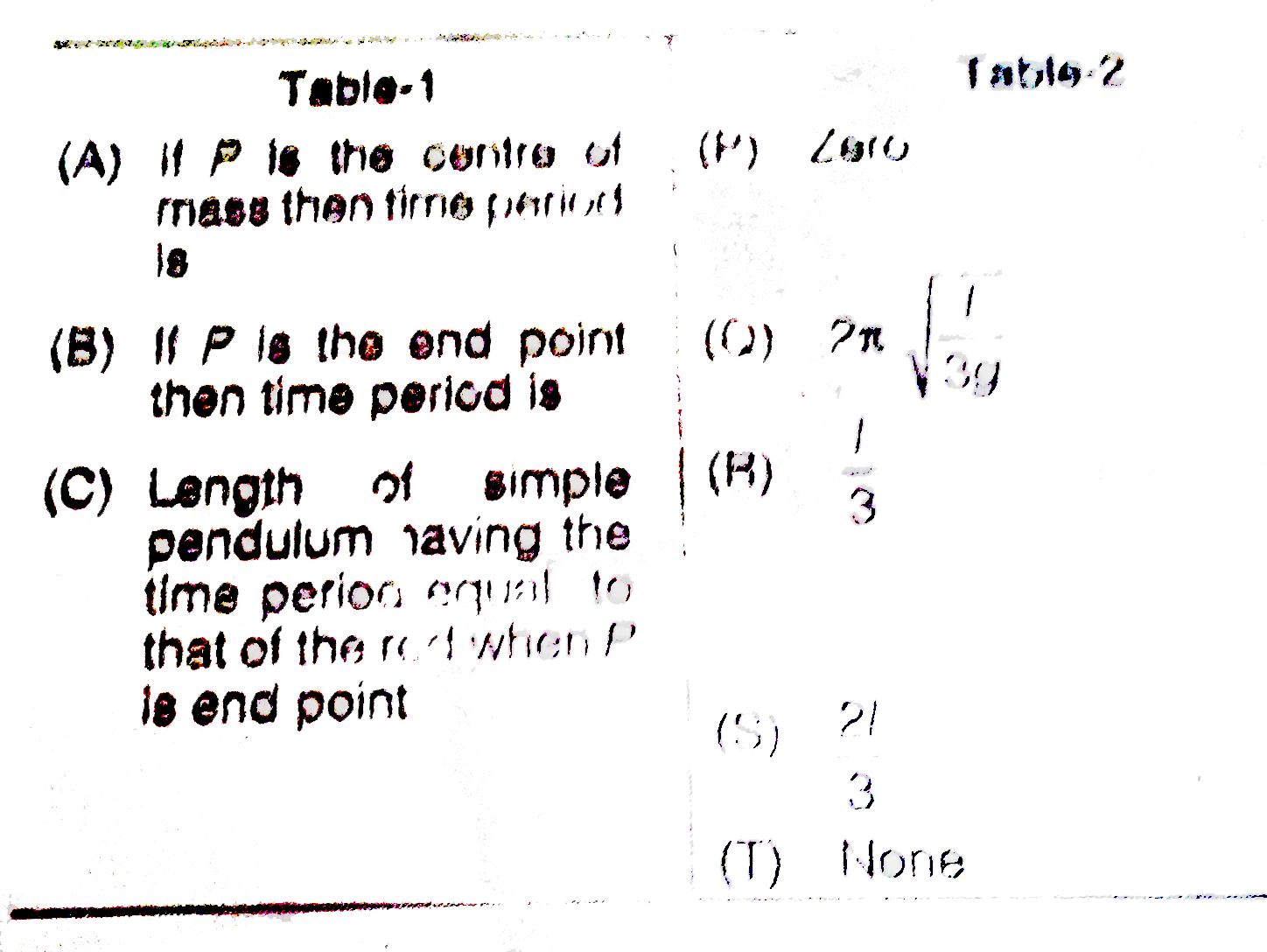
View Solution
Knowledge Check
A uniform thin rod of length l is suspended from one of its ends and is rotated at f rotations per second. The rotational kinetic energy of the rod will be
A23π2f2ml2B43f2ml2C4π2f2ml2DzeroA uniform thin rod of length l is suspended from one of its ends and is rotated at f rotations per second. The rotational kinetic energy of the rod will be
A23π2f2ml2B43f2ml2C4π2f2ml2DZeroRatio of energy density of two steel rods is 1:4 when same mass is suspended from the rods. If length of both rods is same then ratio of diameter of rods will be.
A√2:1B1:√2C1:2D2:1
A uniform rod is kept at smooth horizontal surface, a constant force is applied on the rod in horizontal direction at end 'A'. Find the ratio of energy stored per unit volume at end A to the energy stored per unit volume in the middle of rod.
View SolutionA steel cylindrical rod of length l and radius r is suspended by its end from the ceiling.
(a) Find the elastic deformation energy U of the rod.
(b) Define U in terms of tensile strain Δl/l of the rod.
View SolutionA copper rod of length l is suspended from the ceiling by one of its ends. Find:
(a) the elongation Δl of the rod due to its own weight ,
(b) the relative increment of its volume ΔV/V.
View SolutionA metallic rod of length 'L' and cross-section 'A' has Young's modulus 'Y' and coefficent of linear expansion 'α'. If the rod is heated to a temperature. 'T' the energy stored per unit volume is:
View SolutionTwo rods of same length and cross section are joined along the length. Thermal conductivities of first and second rod are K1 and K2. The temperature of the free ends of the first and seconds rods are maintained at θ1 and θ2 respectively. The temperature of the common junction is
View Solution