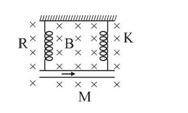
A metal rod of mass 10 gm and length 25cm is suspended on two springs as shown in the figure. The springs are extended by 4cm. When a 20 ampere current passes through the rod it rises by 1cm. The magnetic field is x ×10−2T(g=10m/s2) Find the value of 2x.
The correct Answer is:1
Step by step video, text & image solution for A metal rod of mass 10 gm and length 25cm is suspended on two springs as shown in the figure. The springs are extended by 4cm. When a 20 ampere current passes through the rod it rises by 1cm. The magnetic field is "x " xx 10^(-2)T (g= 10m//s^2) Find the value of 2x. by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Similar Questions
A metal rod of mass 10 gm and length 25 cm is suspended on two springs as shown in Fig. 1.131. The springs are extended by 4 cm. When a 20 A current passes through the rod, it rises by 1 cm. Determine the magnetic field assuming acceleration due to gravity to be 10ms−1.

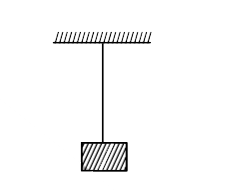
View SolutionA 'block' of mass 10 kg is suspended with string as shown in figure. Find tension in the string. (g=10m/s2)
View Solution
Knowledge Check
A metal rod of uniform thickness and of length 1m is suspended at its 25cm division with help of a string. The rod remains horizontally straight when a block of mass 2kg is suspended to the rod at its 10cm division. The mass of rod is
A0.4kgB0.8kgC1.2kgD1.6kgA conducting rod of 1 m length and 1 kg mass is suspended by two verticle wires through its ends. An external magnetic field of 2T is applied normal to the rod. Now the current to be passed through the rod so as to make the tension in the wire zero is
[Take g=10ms−2]A15 AB5AC1.5AD2.5AA load of mass 100 gm increases the length of wire by 10 cm. If the system is kept in oscillation, its time period is (g=10m/s2)
A0.314sB3.14sC0.628sD6.28s
An iron rod of mass 50 g and length 50 cm is suspended by master wires as shown in the figure. Calculate the magnitude and direction of magnetic field to be set in the region such that the tension is the wires is zero. The current in the rod is 4 A.

View SolutionA conducting rod of 1 m length and 1 kg mass is suspended by two vertical wires through its ends. An external magnetic field of 2T is applied normal to the rod. Now, the current to be passed through the rod so as to make the tension in the wires zero is : (Take g=10ms−2) :
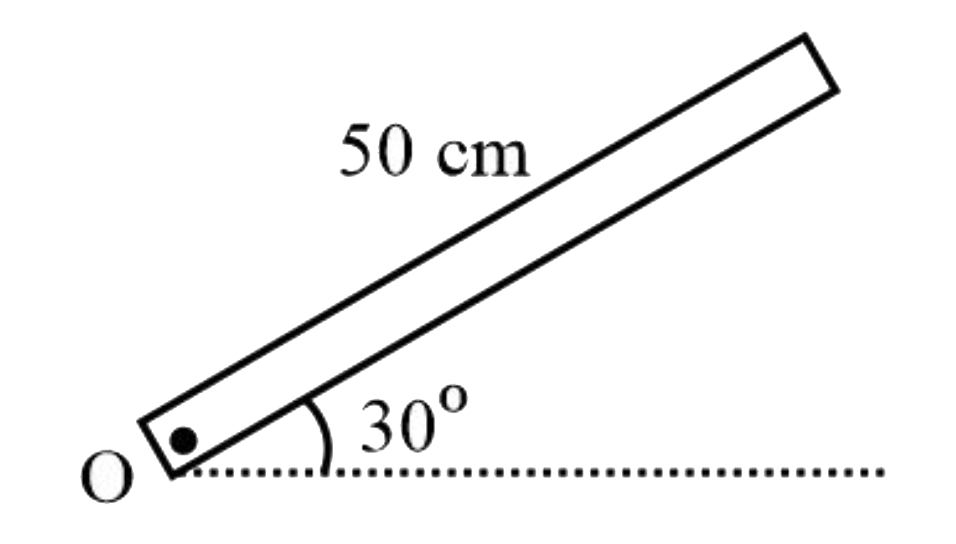
View SolutionA uniform rod of length 50 cm is released in the vertical plane from the position shown in the figure. The rod is hinged smoothly at O. The angular speed of rod when it becomes horizontal is ( take g=10ms−2 )
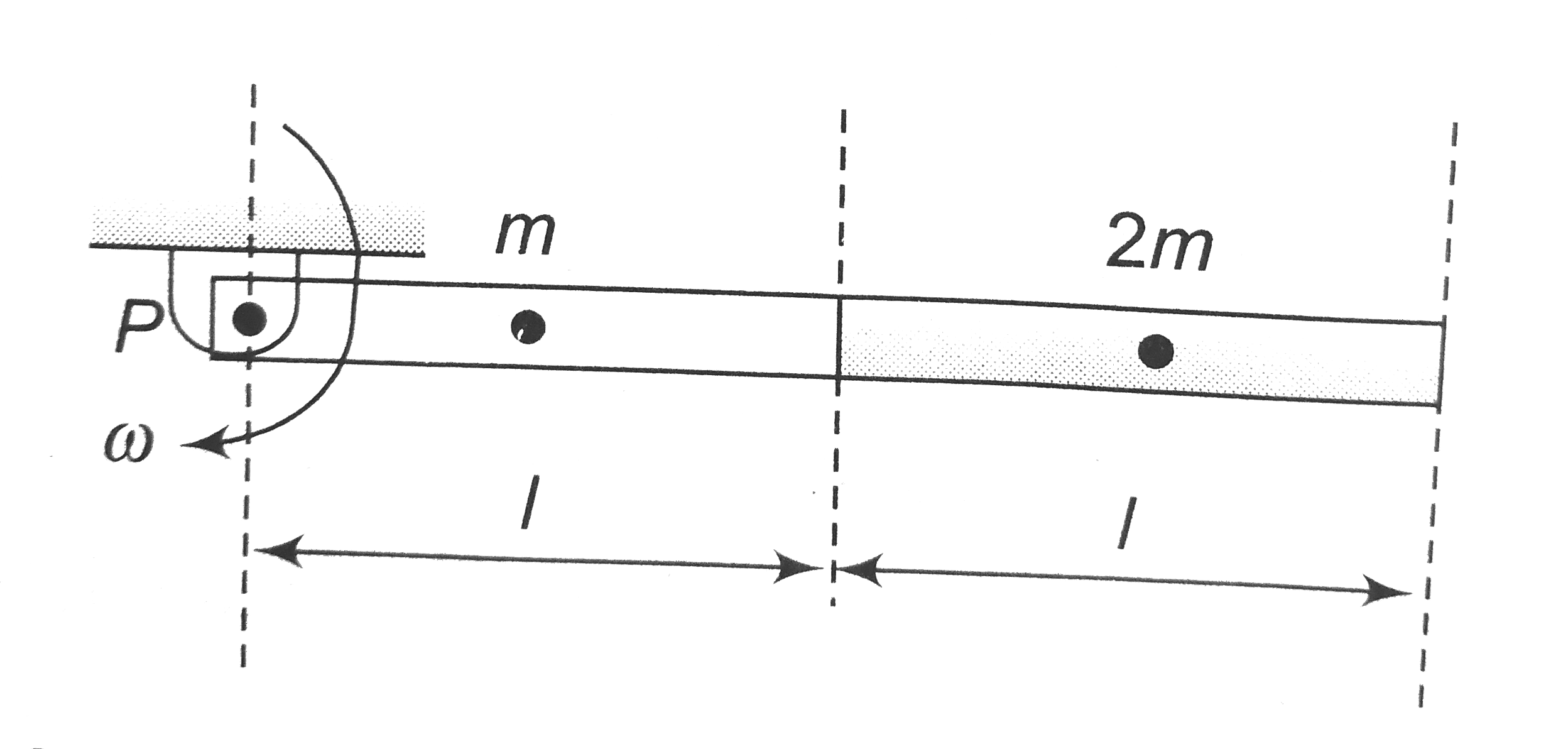
View SolutionA composite rod comprising two rods of mass m and 2m and each of length l=1m as shown in the figure. Assume ω=10rad/s and m=1kg, the KE of the rotating composite rod is.
 .
.
View SolutionIn the figure shown a uniform conducing rod of mass m and length l is suspended invertical plane by two conducing springs of spring constant k each. Upper end of springs are connected to each other by a capacitor of capacitance C. A uniform horizontal magnetic field (B0) perpendicular to plane of springs in space initially rod is in equillibrium. If the rod is pulled down and released, it performs SHM. (Assume resistance of springs and rod are negligible)
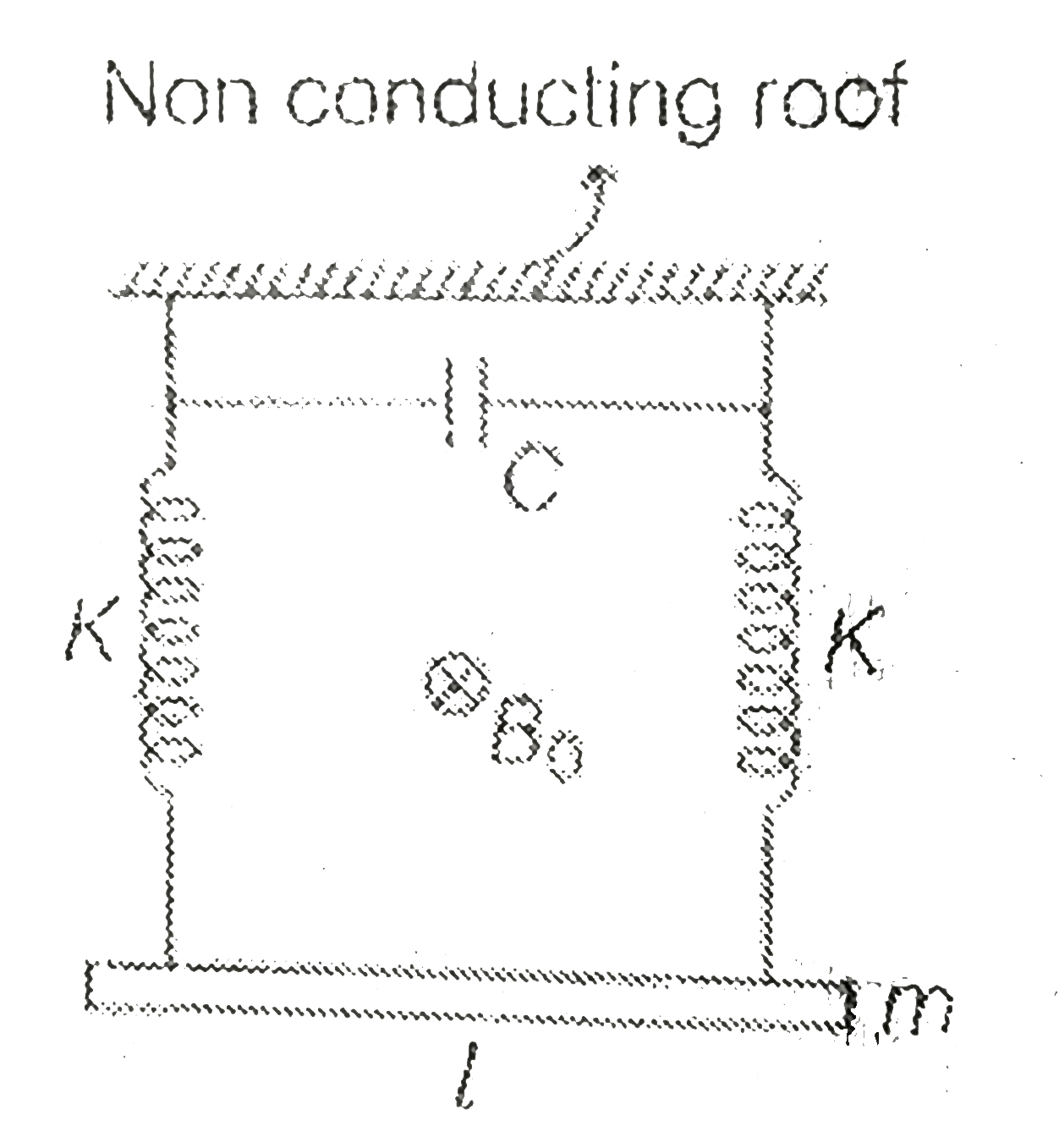
Find the period of oscillation of rod.
View Solution
