Which of the following number lines correctly represents 'p' if,
p−(2p+5)−5(1−2p)=2(3+4p)−3(p−4)
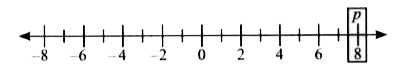
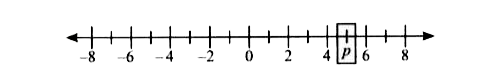


The correct Answer is:D
To solve the equation p−(2p+5)−5(1−2p)=2(3+4p)−3(p−4), we will follow these steps:
Step 1: Expand and simplify both sides of the equation
Left Side:
1. Distribute the negative sign and the multiplication:
p−2p−5−5+10p
This simplifies to:
p−2p+10p−5−5=9p−10
Right Side:
2. Distribute the multiplication:
2⋅3+2⋅4p−3(p−4)
This simplifies to:
6+8p−3p+12=5p+18
Now, we have the simplified equation:
9p−10=5p+18
Step 2: Move all terms involving p to one side and constant terms to the other side
3. Subtract 5p from both sides:
9p−5p−10=18
This simplifies to:
4p−10=18
4. Add 10 to both sides:
4p=28
Step 3: Solve for p
5. Divide both sides by 4:
p=284=7
Conclusion
The value of p is 7.
1. Distribute the negative sign and the multiplication:
p−2p−5−5+10p
This simplifies to:
p−2p+10p−5−5=9p−10
2. Distribute the multiplication:
2⋅3+2⋅4p−3(p−4)
This simplifies to:
6+8p−3p+12=5p+18
9p−10=5p+18
9p−5p−10=18
This simplifies to:
4p−10=18
4p=28
p=284=7
The value of p is 7.
Topper's Solved these Questions
- IMO QUESTION PAPER 2018-19 SET - BBook:SCIENCE OLYMPIAD FOUNDATION Chapter:IMO QUESTION PAPER 2018-19 SET - BExercise:EVERYDAY MATHEMATICS
Explore 10 Videos
- IMO QUESTION PAPER 2018-19 SET - BBook:SCIENCE OLYMPIAD FOUNDATION Chapter:IMO QUESTION PAPER 2018-19 SET - BExercise:ACHIEVERS SECTION
Explore 5 Videos
- IMO QUESTION PAPER 2017-18 SET ABook:SCIENCE OLYMPIAD FOUNDATION Chapter:IMO QUESTION PAPER 2017-18 SET AExercise:ACHIEVERS SECTION
Explore 5 Videos
- IMO QUESTION PAPER 2018-19 SET ABook:SCIENCE OLYMPIAD FOUNDATION Chapter:IMO QUESTION PAPER 2018-19 SET AExercise:Logical Reasoning
Explore 20 Videos
Similar Questions
Knowledge Check
The sum of four prime numbers of two digits is 204. The sum of the first and last i.e, (p1+p4) is same as the sum of second number p2 and third number p3 (i.e., p2+p3). Again p3−p2=2(p2−p1)=2(p4−p3). The average number of all the four numbers is not a prime number, but a product of two prime numbers one of these prime numbers (out of four) is :
Aa. 23Bb. 89Cc. 71Dd. can't be determinedThe sum of four prime numbers of two digits is 204. The sum of the first and last i.e, (p1+p4) is same as the sum of second number p2 and third number p3 (i.e., p2+p3). Again p3−p2=2(p2−p1)=2(p4−p3). The average number of all the four numbers is not a prime number, but a product of two prime numbers one of these prime numbers (out of four) is :
A23B89C71Dcan't be determinedSolve for p:2p(p−3)+5(p−2)=0
A2,212B212,−2C−212,2D−212,−2
Add the following expressions:
58p4+2p2+58,18−17p+98p2andp5−p3+7
View Solution2p−(7−5p)9p−(3+4p)=76
View SolutionSolve the following:
4 (3p + 2) – 5(6p –1) = 2(p – 8) – 6(7p – 4)
View SolutionThe number of cubic polynomials p(x) satisfying p(1)=2, p(2)=4, p(3)=6, p(4)=8 is
View Solutionp+37−2p−53=3p−55−25
View Solution