The total number of students in three classes of an Engineering Institute is 885. The strength of the students in the first two classes are in the ratio of 4: 9. The ratio of the number of students in the second and the third classes is 6: 11. How many students are there in the class that has the maximum number of students?
594
495
954
459
The correct Answer is:B
To solve the problem step by step, we will first define the variables based on the information given in the question.
Step 1: Define Variables
Let the number of students in the first class be 4x and in the second class be 9x (since the ratio of the first two classes is 4:9).
Let the number of students in the third class be y.
Step 2: Set Up the Equation
According to the problem, the total number of students in all three classes is 885. Therefore, we can write the equation:
4x+9x+y=885
This simplifies to:
13x+y=885(1)
Step 3: Use the Second Ratio
We are also given that the ratio of the number of students in the second and third classes is 6:11. This can be expressed as:
9xy=611
Cross-multiplying gives us:
11⋅9x=6y
This simplifies to:
99x=6y(2)
Step 4: Solve for y
From equation (2), we can express y in terms of x:
y=99x6=16.5x
Step 5: Substitute y in Equation (1)
Now we substitute y in equation (1):
13x+16.5x=885
This simplifies to:
29.5x=885
Step 6: Solve for x
Now we can solve for x:
x=88529.5=30
Step 7: Find the Number of Students in Each Class
Now that we have x, we can find the number of students in each class:
- First class: 4x=4×30=120
- Second class: 9x=9×30=270
- Third class: y=16.5x=16.5×30=495
Step 8: Identify the Class with Maximum Students
Now we compare the number of students in each class:
- First class: 120 students
- Second class: 270 students
- Third class: 495 students
The class with the maximum number of students is the third class with 495 students.
Final Answer
The number of students in the class that has the maximum number of students is 495.
---
Let the number of students in the first class be 4x and in the second class be 9x (since the ratio of the first two classes is 4:9).
According to the problem, the total number of students in all three classes is 885. Therefore, we can write the equation:
4x+9x+y=885
This simplifies to:
13x+y=885(1)
We are also given that the ratio of the number of students in the second and third classes is 6:11. This can be expressed as:
9xy=611
Cross-multiplying gives us:
11⋅9x=6y
This simplifies to:
99x=6y(2)
From equation (2), we can express y in terms of x:
y=99x6=16.5x
Now we substitute y in equation (1):
13x+16.5x=885
This simplifies to:
29.5x=885
Now we can solve for x:
x=88529.5=30
Now that we have x, we can find the number of students in each class:
- First class: 4x=4×30=120
- Second class: 9x=9×30=270
- Third class: y=16.5x=16.5×30=495
Now we compare the number of students in each class:
- First class: 120 students
- Second class: 270 students
- Third class: 495 students
The number of students in the class that has the maximum number of students is 495.
Similar Questions
Knowledge Check
In a class the girls are 60 % of the total number of students and the boys are 18 in number . How many students are there in the class ?
A35B25C45D55The class, in which the marks of maximum number of students lies, is
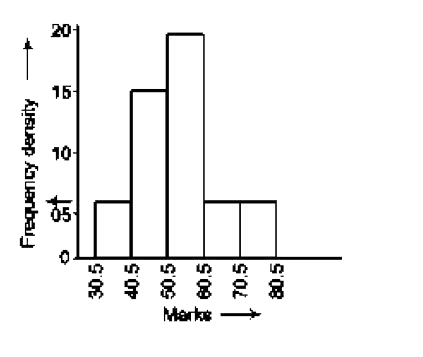 A40.5 to 50.5B50.5 to 60.5C60.5 to 70.5D70.5 to 80.5
A40.5 to 50.5B50.5 to 60.5C60.5 to 70.5D70.5 to 80.5The students in three classes are in the ratio 2:3:5. If 20 students are increased in each class, the ratio changes to 4:5:7. Originally the total number of students was :
A120B150C200D100
The following bar graph shows the number of students in different classes of a school.
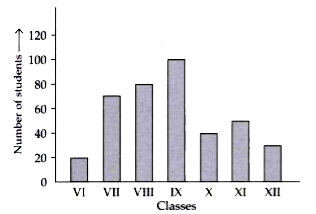
How many more students are there in Class IX than in Class VIII ?
View SolutionOut of 30 students in a class, 20 like cricket and 10 like Hockey. The ratio of the number of students liking Hockey to the total number of students is
View Solution42% of the total number of student passed in a class test. How many students failed, if the total number of students in the class is 1450 ?
View SolutionThe number of students in three classes are in the ratio 2 : 3:5. If 40 students are increased in each class, the ratio changes to 4:5:7. Originally, the total number of students was :
View SolutionIf the students of a class can be grouped exactly into 6 or 8 or 10, then the minimum number of students in the class must be
View Solution

