The magnetic field perpendicular to the plane of a loop of area 7m2 is 13T. Find the magnetic flux of the loop?
12 Wb
12 Wb
91 Wb
554 Wb
The correct Answer is:C
To find the magnetic flux through a loop when the magnetic field is perpendicular to the plane of the loop, we can use the formula for magnetic flux (Φ):
Φ=B⋅A⋅cos(θ)
Where:
- Φ is the magnetic flux,
- B is the magnetic field strength,
- A is the area of the loop,
- θ is the angle between the magnetic field and the normal (perpendicular) to the surface of the loop.
1. Identify the Given Values:
- Area of the loop, A=7m2
- Magnetic field strength, B=13T
- Since the magnetic field is perpendicular to the plane of the loop, the angle θ=0∘.
2. Calculate Cosine of the Angle:
- cos(0∘)=1
3. Substitute the Values into the Formula:
- Now, we can substitute the values into the magnetic flux formula:
Φ=B⋅A⋅cos(θ)=13T⋅7m2⋅1
4. Perform the Multiplication:
- Calculate 13⋅7:
13⋅7=91
5. Final Result:
- Therefore, the magnetic flux Φ=91Wb (Weber).
Conclusion:
The magnetic flux through the loop is 91Wb.
---
- Φ is the magnetic flux,
- B is the magnetic field strength,
- A is the area of the loop,
- θ is the angle between the magnetic field and the normal (perpendicular) to the surface of the loop.
- Area of the loop, A=7m2
- Magnetic field strength, B=13T
- Since the magnetic field is perpendicular to the plane of the loop, the angle θ=0∘.
- cos(0∘)=1
- Now, we can substitute the values into the magnetic flux formula:
Φ=B⋅A⋅cos(θ)=13T⋅7m2⋅1
- Calculate 13⋅7:
13⋅7=91
- Therefore, the magnetic flux Φ=91Wb (Weber).
The magnetic flux through the loop is 91Wb.
Similar Questions
The magnetic field perpendicular to the plane of a loop of area 0.1m2 is 0.2 T. Calculate the magnetic flux through the loop (in weber)
View SolutionA circular loop of area 1cm2 , carrying a current of 10 A , is placed in a magnetic field of 0.1 T perpendicular to the plane of the loop. The torque on the loop due to the magnetic field is
View Solution
Knowledge Check
A conducting circular loop of radius r carries a constant current i. It is placed in a uniform magnetic field B such that B is perpendicular to the plane of loop. What is the magnetic force acting on the loop?
AI r BB2πrIBCzeroDπrIB
Assertion A conducting loop is rotated in a uniform magnetic field with constant angular velocity ω as shown in figure. At time t = 0, plane of the loop is perpendicular to the magnetic field. Induced emf produced in the loop is maximum when plane of loop is parallel to magnetic field.
Reason When plane of loop is parallel to magnetic field, then magnetic flux passing through the loop is zero.
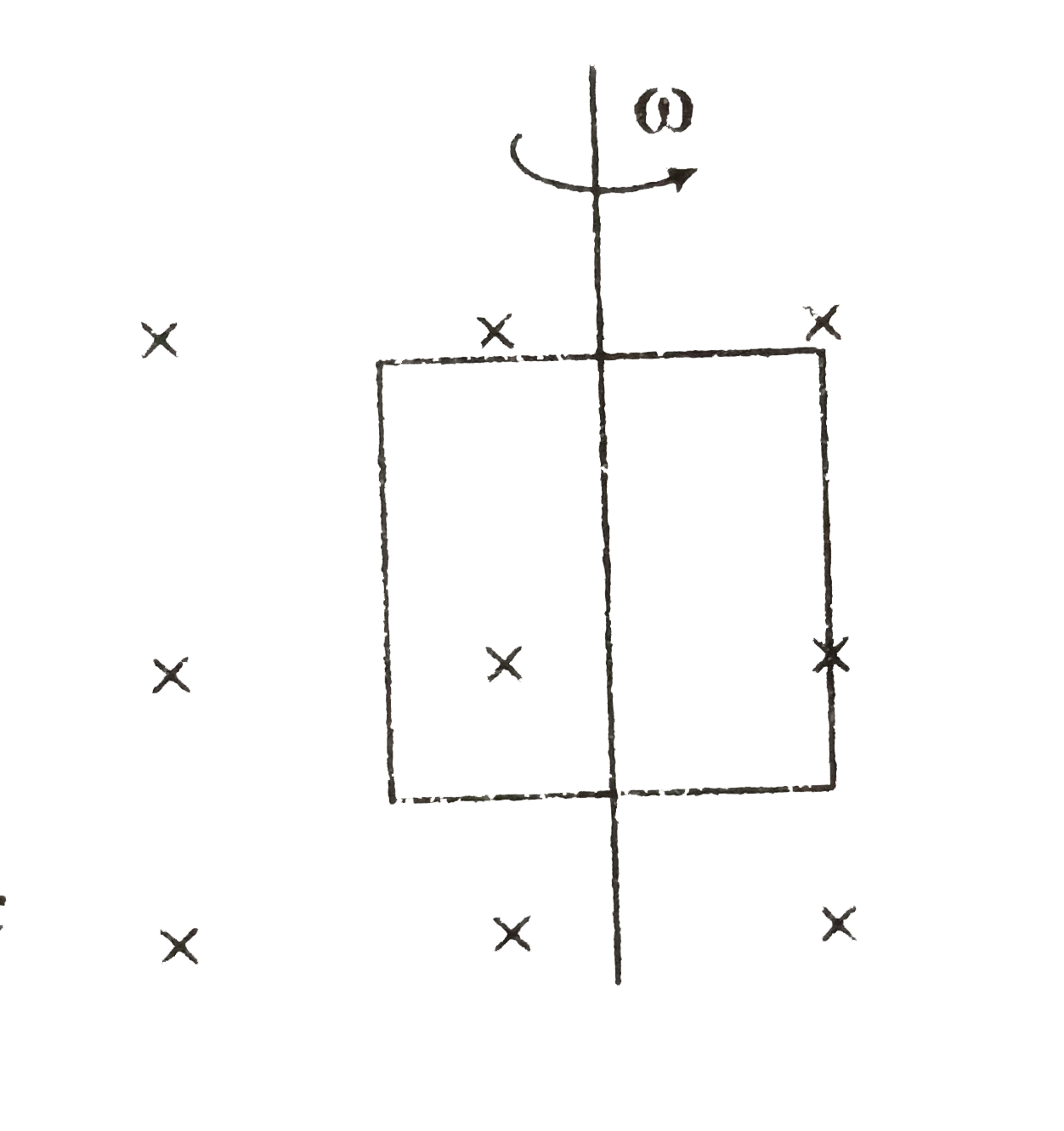
View SolutionA square metallic wire loop of side 0.1 m and resistance of 1Ω is moved with a constant velocity in a magnetic field of 2wb/m2 as shown in figure. The magnetic field field is perpendicular to the plane of the loop, loop is coonected to a network of resistances. what should be the velocity of loop so as to have a steady current of 1mA in loop?
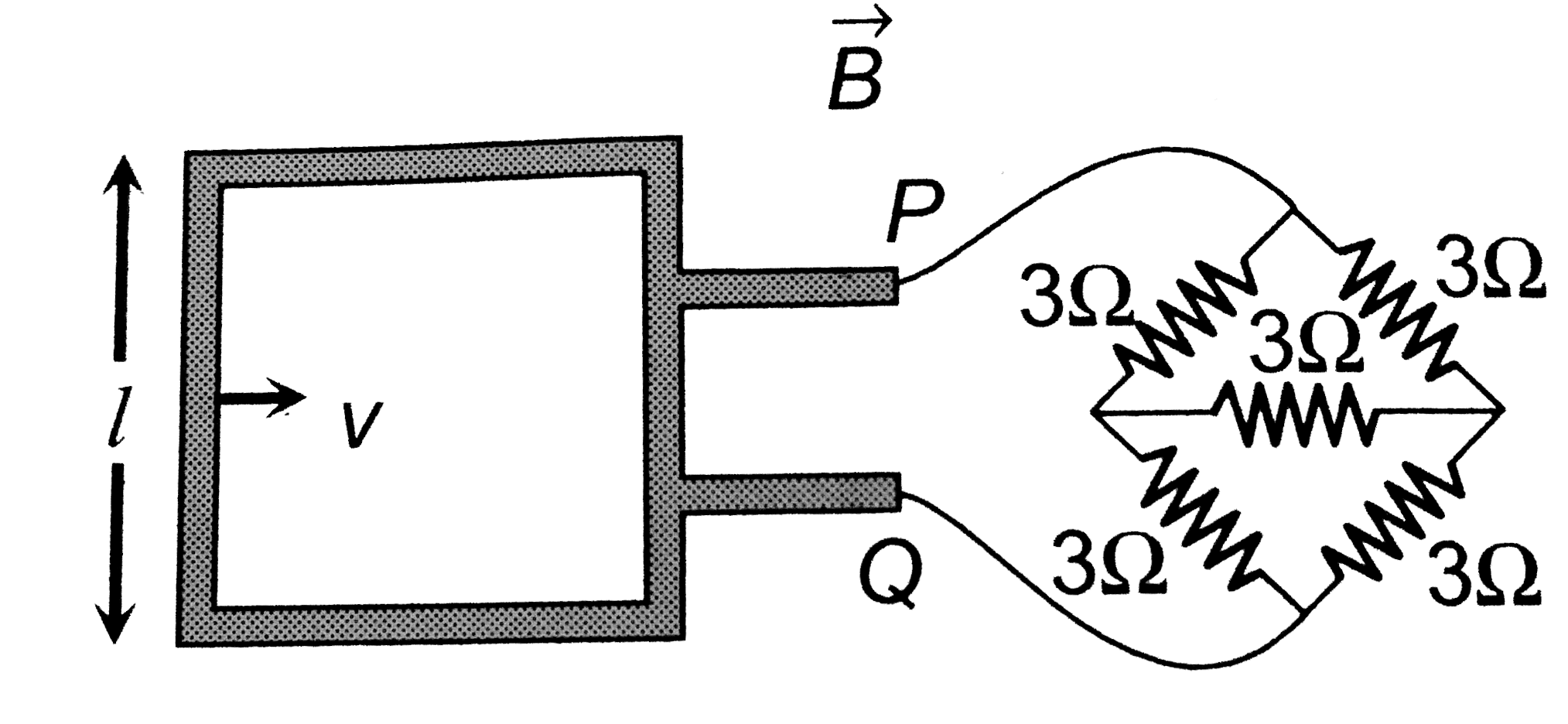
View SolutionA square metal wire loop of side 10cm and resistances 1Ω is moved with constant velocity v0 in a uniform magnetic field of induction B=2Wbm−2, as shown in Fig. 3.218. The magnetic field lines are perpendicular to the plane of loop network of resistances, each of value 3Ω. The speed of the loop so as to have a steady current of 1mA in the loop is

View SolutionA square metal wire loop of side 20 cm and resistance 2Ω is moved with a constant velcoity v0 in a uniform magnetic field of induction B = 1 Wb/m2 as shown in the figure. The magnetic field lines are perpendicular to the plane of the loop. The loop is connected to a network of resistance each of value 5Ω. The resistances of the lead wires BF and AE are negligible. What should be the speed of the loop, so as to have a steady current of 2 mA in the loop? Give the direction of current in the loop.

View SolutionA circular loop of radius R is kept in a uniform magnetic field pointing perpendicular into the plane of paper. When a current l flows in the loop, the tension produced in the loop is

View SolutionIn Fig. there is a frame consisting of two square loops having resistors and inductors as shown. This frame is placed in a uniform but time-varying magnetic field in such a way that one of the loops is placed in crossed magentic field and the other is placed in dot magnetic field. Both magnetic fields are perpendicular to the planes of the loops.
If the magnetic field is given by B=(20+10t)Wbm−2 in both regions [l=20cm,b=10cm, and R=10Ω, L=10H),
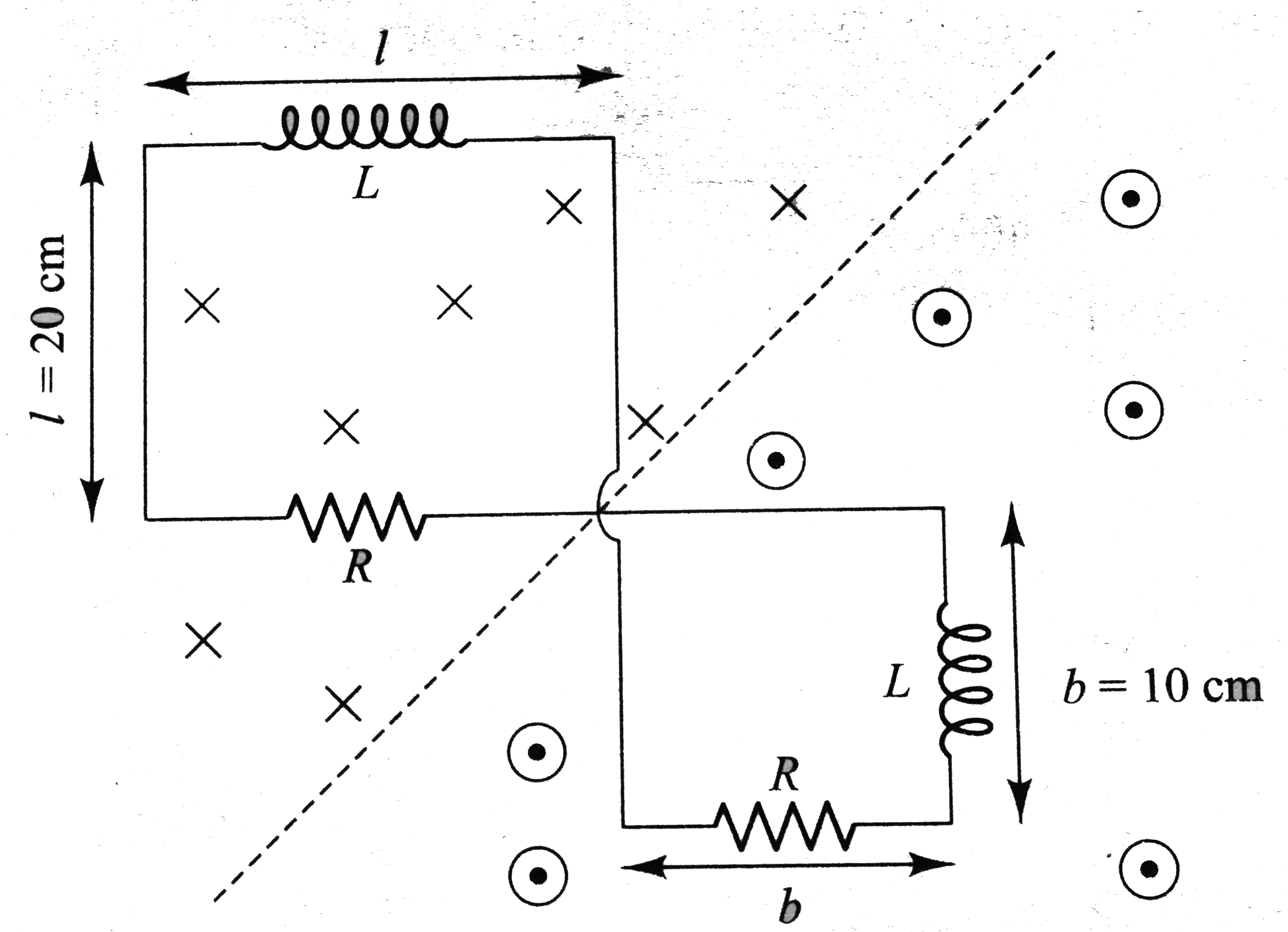
The direction in induced current in the bigger loop will be
View SolutionIn Fig. there is a frame consisting of two square loops having resistors and inductors as shown. This frame is placed in a uniform but time-varying magnetic field in such a way that one of the loops is placed in crossed magentic field and the other is placed in dot magnetic field. Both magnetic fields are perpendicular to the planes of the loops.
If the magnetic field is given by B=(20+10t)Wbm−2 in both regions [l=20cm,b=10cm, and R=10Ω, L=10H),
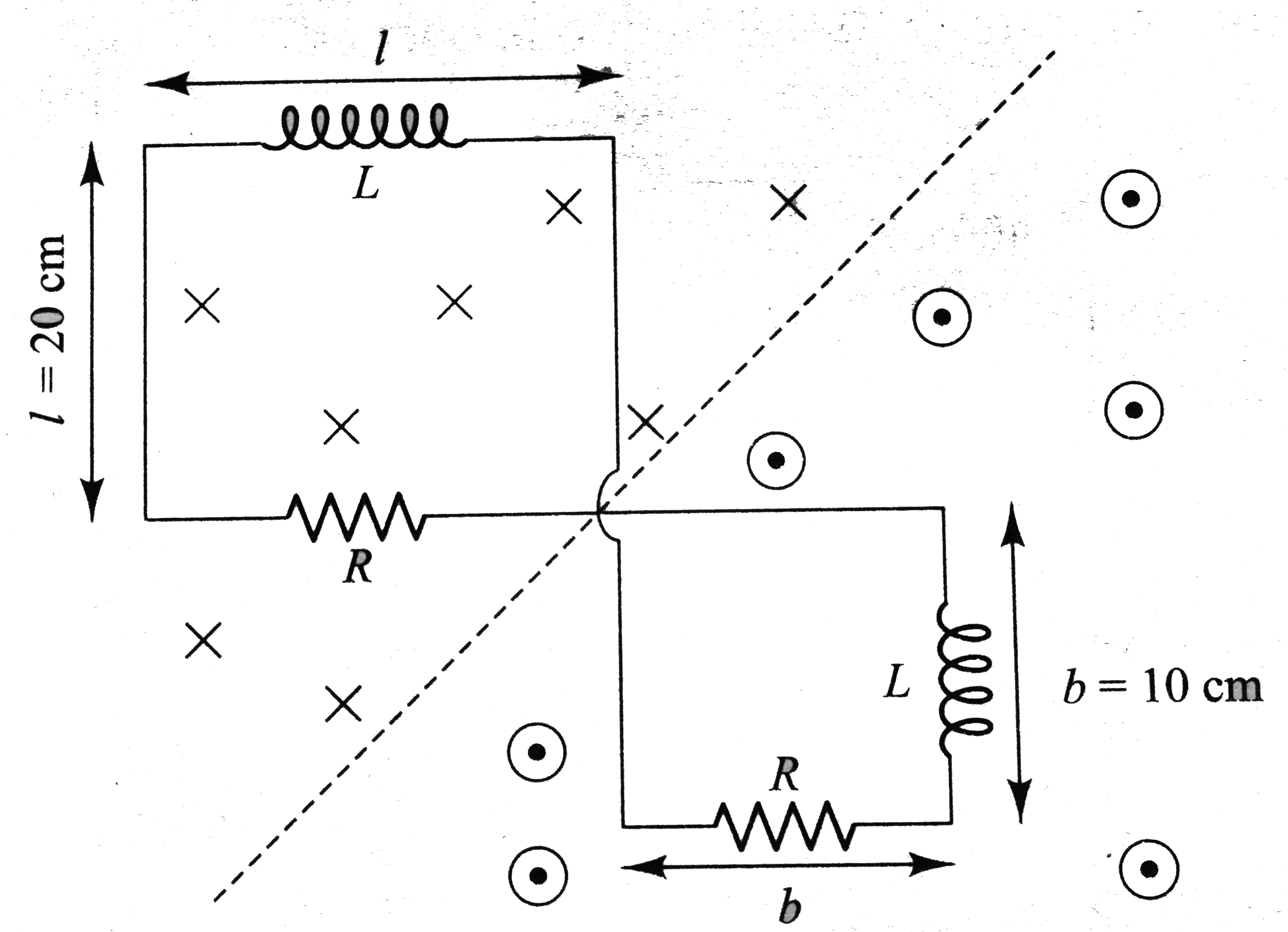
The current in the frame as a funtion of time will be
View Solution
