If degree, then the value of is ?
Infinite
Between Zero and 1
One
The correct Answer is:B
To solve the problem, we need to find the value of the induced electromotive force (emf), denoted as e, when the angle θ is given as 270 degrees.
1. Identify the formula for induced emf:
The induced emf (e) is given by the formula:
\(
e = e_0 \sin(\theta)
\)
where \( e_0 \) is the maximum induced emf and \( \theta \) is the angle.
2. Substitute the given value of theta:
We are given that \( \theta = 270^\circ \). We can substitute this value into the formula:
\(
e = e_0 \sin(270^\circ)
\)
3. Calculate sin(270 degrees):
The sine of 270 degrees is a known value:
\(
\sin(270^\circ) = -1
\)
4. Substitute the value of sin(270 degrees):
Now we can substitute this value back into the equation for e:
\(
e = e_0 \cdot (-1)
\)
This simplifies to:
\(
e = -e_0
\)
5. Conclusion:
Therefore, the value of \( e \) when \( \theta = 270^\circ \) is:
\(
e = -e_0
\)
Final Answer:
The value of e is \(-e_0\).
The induced emf (e) is given by the formula:
\(
e = e_0 \sin(\theta)
\)
where \( e_0 \) is the maximum induced emf and \( \theta \) is the angle.
We are given that \( \theta = 270^\circ \). We can substitute this value into the formula:
\(
e = e_0 \sin(270^\circ)
\)
The sine of 270 degrees is a known value:
\(
\sin(270^\circ) = -1
\)
Now we can substitute this value back into the equation for e:
\(
e = e_0 \cdot (-1)
\)
This simplifies to:
\(
e = -e_0
\)
Therefore, the value of \( e \) when \( \theta = 270^\circ \) is:
\(
e = -e_0
\)
The value of e is \(-e_0\).
Similar Questions
If sin2θ=14 , then the value of θ is
View SolutionIf sinθ=√3cosθ,−π<θ<0, then the value of θ is
View SolutionWhat is the value of θ ?
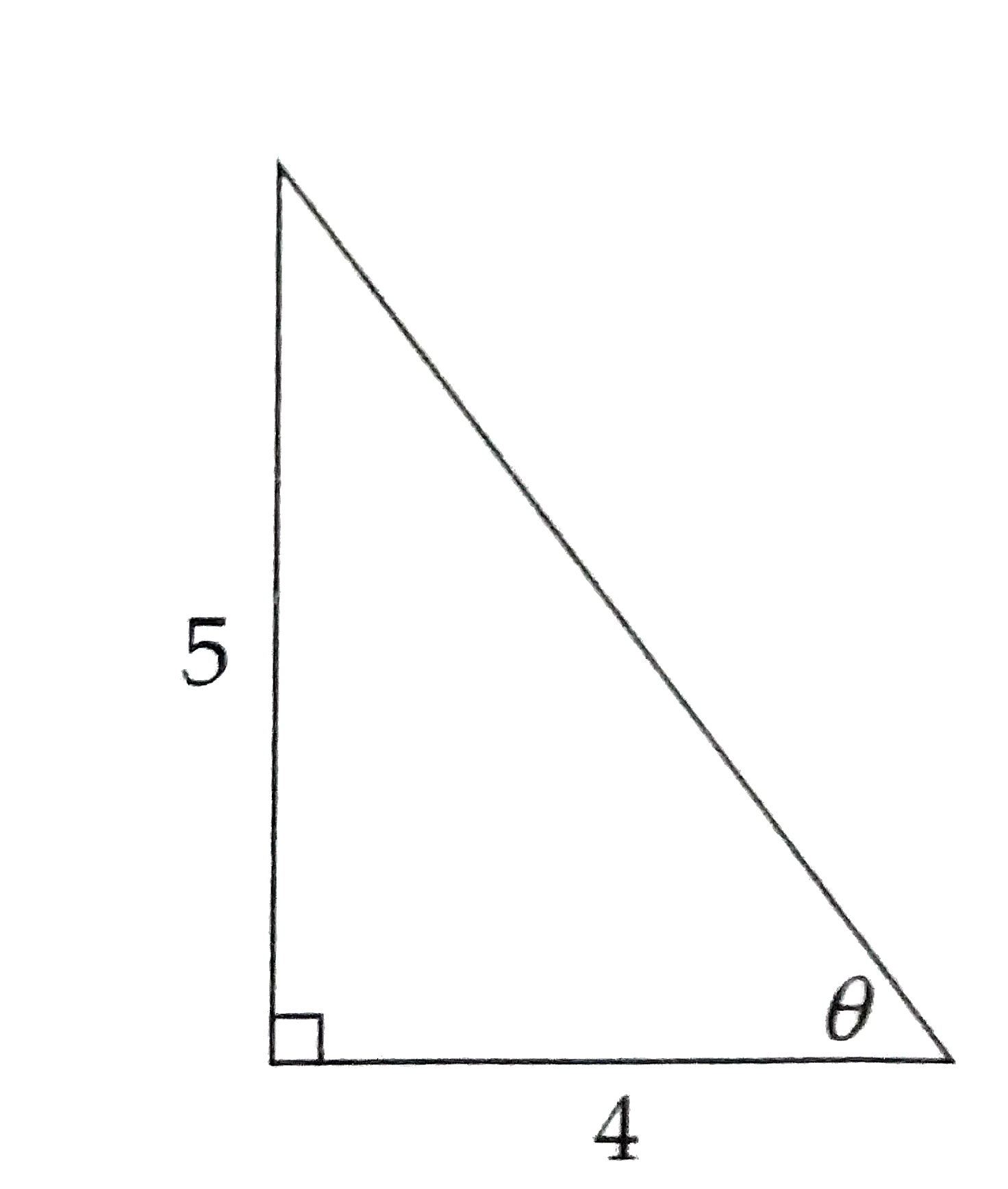
View SolutionIf tan2θ= 1−e2 then the value of secθ+tan3θcosecθ
View SolutionIf sinθ+cosecθ=2, then find the value of sin20θ+cosec20θ.
View SolutionIf sinθ+cosecθ=2, then the value of sin8θ+cosec8θ is equal to
View SolutionIf tan4θ+tan2θ=2, then the value of cos4θ+cos2θ is-
View SolutionIf 3sinθ+4cosθ=5, then find the value of 4sinθ−3cosθ.
View SolutionIf 5 cot θ=12 , find the value of : cosecθ+secθ
View SolutionIf tan3θ=cotθ, then general value of θ is :
View Solution