Boat A travel downstream from point X to point Y in 3 hours less than time taken by boat B to travel upstream point Y to Z distance between X and Y is 20 km which is half of the distance between Y and Z ,the speed of boat B in still water is 10 kmph and speed of boat A in still water is equal to the speed of boat B upstream, what is speed of A in still water consider the speed of current to be same
10km/hr
16km/hr
12km/hr
8km/hr
The correct Answer is:D
To solve the problem step by step, we can follow the reasoning provided in the video transcript. Here’s the structured solution:
Step 1: Identify the distances
- The distance from point X to point Y is given as 20 km.
- The distance from point Y to point Z is double that of X to Y, so it is 40 km.
Step 2: Define the speeds
- Let the speed of the current (stream) be denoted as c km/h.
- The speed of boat B in still water is given as 10 km/h.
- The speed of boat A in still water is equal to the speed of boat B upstream. Therefore, the speed of boat A in still water is also 10−c km/h (since upstream speed is reduced by the speed of the current).
Step 3: Calculate the time taken by both boats
- Time taken by Boat A (downstream from X to Y):
- Speed downstream = Speed of A + Speed of current = (10−c)+c=10 km/h.
- Time taken = Distance / Speed = 20/10=2 hours.
- Time taken by Boat B (upstream from Y to Z):
- Speed upstream = Speed of B - Speed of current = 10−c km/h.
- Time taken = Distance / Speed = 40/(10−c).
Step 4: Set up the equation based on the time difference
According to the problem, Boat A takes 3 hours less than Boat B:
2=4010−c−3
Step 5: Solve the equation
1. Rearranging the equation:
4010−c=5
2. Cross-multiplying gives:
40=5(10−c)
3. Expanding and simplifying:
40=50−5c
5c=50−40
5c=10
c=2 km/h
Step 6: Find the speed of Boat A in still water
- The speed of Boat A in still water is:
10−c=10−2=8 km/h
Final Answer
The speed of Boat A in still water is 8 km/h.
---
- The distance from point X to point Y is given as 20 km.
- The distance from point Y to point Z is double that of X to Y, so it is 40 km.
- Let the speed of the current (stream) be denoted as c km/h.
- The speed of boat B in still water is given as 10 km/h.
- The speed of boat A in still water is equal to the speed of boat B upstream. Therefore, the speed of boat A in still water is also 10−c km/h (since upstream speed is reduced by the speed of the current).
- Time taken by Boat A (downstream from X to Y):
- Speed downstream = Speed of A + Speed of current = (10−c)+c=10 km/h.
- Time taken = Distance / Speed = 20/10=2 hours.
- Speed upstream = Speed of B - Speed of current = 10−c km/h.
- Time taken = Distance / Speed = 40/(10−c).
According to the problem, Boat A takes 3 hours less than Boat B:
2=4010−c−3
1. Rearranging the equation:
4010−c=5
2. Cross-multiplying gives:
40=5(10−c)
3. Expanding and simplifying:
40=50−5c
5c=50−40
5c=10
c=2 km/h
- The speed of Boat A in still water is:
10−c=10−2=8 km/h
The speed of Boat A in still water is 8 km/h.
Similar Questions
Boat A travels downstream from Point X to Point Y in 3 hours less than the time taken by Boat B travel upstream from Point Y to Point Z. The distance between X and Y is 20 km, which is half of the distance between Y and Z. The speed of Boat B in still water is 10 km/h and the speed of Boat A in still water is equal to the speed of Boat B upstream What is the speed of Boat A in Still water? (Consider the speed of the current to be the same.) a. 10 km/h b. 16 km/h c. 12 km/h d. 8 km/h
View SolutionSpeed of boat in still water when speed of downstream and upstream are given.
View Solution
Knowledge Check
A boat takes 5 hours more while going back in upstream than in downstream if the distance between two places is 24 km and the speed of boat in still water be 5.5 km/h what must be the speed of boat in still water so that it can row downstream 24 km in 4 hours?
A20 km/hB10 km /hC12 km /hD14 km/hThe speed of a boat in still water is 12 kmph . If the boat covers 36 km upstream in 4 hours , what is the speed of stream ?
A3 km/hrB4 km/hrC5 km/hrD2 km/hrIf a boat goes 7 km upstream in 42 minutes and the speed of the stream is 3 kmph, the speed of the boat in still water is :
A9 km/hrB13 km/hrC8 km/hrD21 km/hr
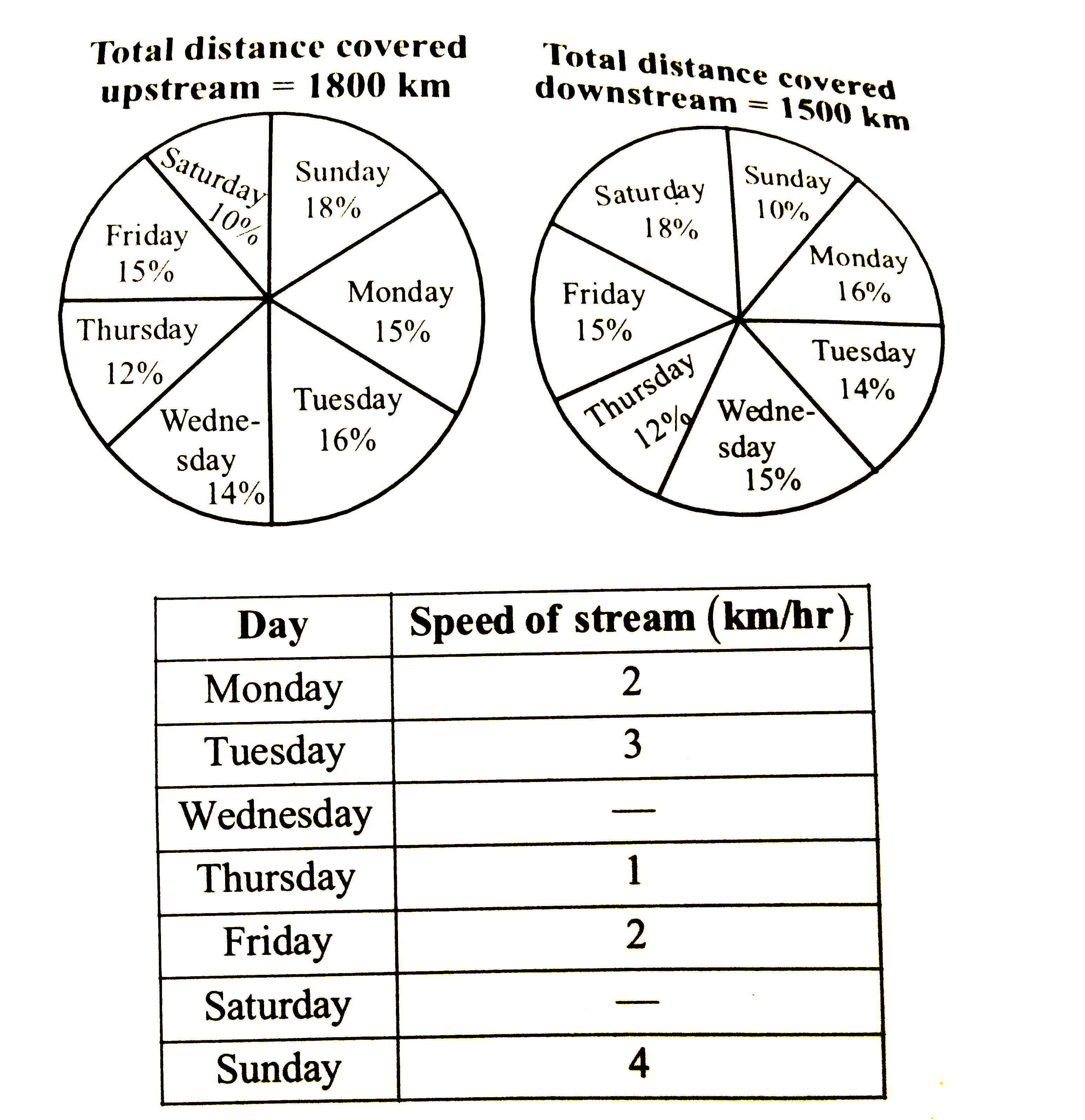
If the time taken by boat to travel upstream on Thursday is equla to the time taken by it to travel downstrem on Monday and the speed of boat in still water on Monday is 16 kmph then the speed in still water on Thursday?
View SolutionDownstream speed of boat is 50 km/h and upstream speed of boat is 30 km/h. Find the speed of boat in still water and speed of stream?
View SolutionA boat goes 20 km downstream in 1 h and the same distance upstream in 2h. The speed of the boat in still water is-
View Solution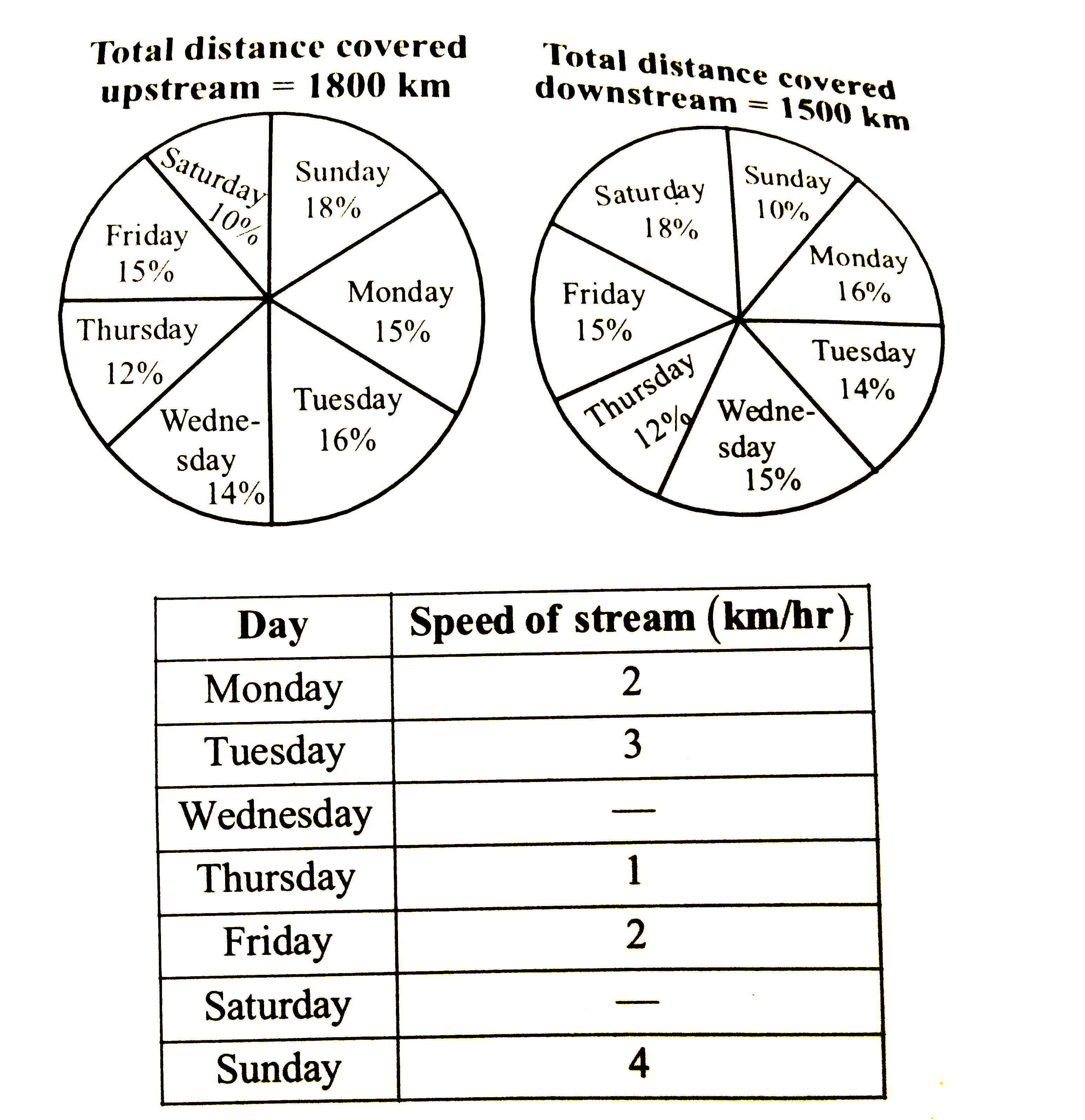
If the taken by boat to travel upstream on Monday is 4511 hrs. more than the time taken by it to travel downstream on the day, then find the speed of boat in still water on Monday ?
View SolutionA man can row downstream at 12 km/h and upstream at 8 kn/h what is the speed of man in still water?
View Solution
