Study the information carefully to answer the questions that follow:
A school consisting of 1560 students has boys and girls in the ratio of 7:5. All the students are enrolled for different countries’ tours, viz Russia, Switzerland and Japan. One-fifth of the boys are enrolled for only Switzerland tour. Twenty per cent of the girls are enrolled for only Japan tour. Ten, per cent of the boys are enrolled for only Russia tour. Twenty four per cent of the girls are enrolled for both Russia and Switzerland tour together but not for Japan.. The number of the girls enrolled for only Russia tour is two hundred per cent of the boys enrolled for the same tour. One-thirteenth of the boys are enrolled for all the three tours together. The ratio of the boys enrolled for Switzerland and Japan tour together but not for Russia to the,girls enrolled for the same tour is 2 : 1. Ten per cent of the girls are enrolled for only Switzerland tour whereas eight per cent of the girls are enrolled for both Switzerland and Japan tour together but not for Russia tour. The remaining girls are enrolled for all the three tours together. The number of boys enrolled for Russia and Switzerland tour together but not for Japan tour is fifty per cent of the number of girls enrolled for the same. The remaining boys are enrolled for only Japan tour.
What is the ratio ofthe number of girls enrolled for only Japan tour and the number of boys' enrolled for only Switzerland tour together to the number of boys enrolled for only Japan tour and the number of girls enrolled for only Switzerland tour together?
The correct Answer is:C
To solve the problem step by step, we need to extract the relevant information and calculate accordingly.
Step 1: Determine the number of boys and girls in the school.
- The total number of students is 1560.
- The ratio of boys to girls is 7:5.
Let the number of boys be 7x and the number of girls be 5x.
Setting up the equation:
7x+5x=156012x=1560x=130
Calculating the number of boys and girls:
Boys=7x=7×130=910Girls=5x=5×130=650
Step 2: Calculate the enrollment for specific tours.
1. Boys enrolled for only Switzerland tour:
Boys for Switzerland=15×910=182
2. Girls enrolled for only Japan tour:
Girls for Japan=20%×650=0.2×650=130
3. Boys enrolled for only Russia tour:
Boys for Russia=10%×910=0.1×910=91
4. Girls enrolled for both Russia and Switzerland tour (not Japan):
Girls for Russia and Switzerland=24%×650=0.24×650=156
5. Girls enrolled for only Russia tour:
Girls for only Russia=200%×91=2×91=182
6. Boys enrolled for all three tours:
Boys for all three=113×910=70
Step 3: Calculate remaining enrollments.
1. Girls for only Switzerland tour:
Girls for only Switzerland=10%×650=65
2. Girls for both Switzerland and Japan (not Russia):
Girls for Switzerland and Japan=8%×650=52
3. Remaining girls for all three tours:
Remaining girls=650−(65+130+182+156+52)=65
Step 4: Calculate boys and girls for tours.
1. Boys for Russia and Switzerland (not Japan):
Boys for Russia and Switzerland=50%×156=78
2. Remaining boys for only Japan tour:
Remaining boys=910−(182+91+70+78)=489
Step 5: Calculate the required ratio.
- Girls enrolled for only Japan tour: 130
- Boys enrolled for only Switzerland tour: 182
- Boys enrolled for only Japan tour: 489
- Girls enrolled for only Switzerland tour: 65
Now, we need to find the ratio:
Ratio=130+182489+65=312554
Step 6: Simplify the ratio.
To simplify 312554:
1. Find the GCD of 312 and 554.
2. Divide both the numerator and denominator by their GCD.
The GCD is 2:
312÷2554÷2=156277
Final Answer:
The ratio of the number of girls enrolled for only Japan tour and the number of boys enrolled for only Switzerland tour together to the number of boys enrolled for only Japan tour and the number of girls enrolled for only Switzerland tour together is 156:277.
- The total number of students is 1560.
- The ratio of boys to girls is 7:5.
7x+5x=156012x=1560x=130
Boys=7x=7×130=910Girls=5x=5×130=650
1. Boys enrolled for only Switzerland tour:
Boys for Switzerland=15×910=182
Girls for Japan=20%×650=0.2×650=130
Boys for Russia=10%×910=0.1×910=91
Girls for Russia and Switzerland=24%×650=0.24×650=156
Girls for only Russia=200%×91=2×91=182
Boys for all three=113×910=70
1. Girls for only Switzerland tour:
Girls for only Switzerland=10%×650=65
Girls for Switzerland and Japan=8%×650=52
Remaining girls=650−(65+130+182+156+52)=65
1. Boys for Russia and Switzerland (not Japan):
Boys for Russia and Switzerland=50%×156=78
Remaining boys=910−(182+91+70+78)=489
- Girls enrolled for only Japan tour: 130
- Boys enrolled for only Switzerland tour: 182
- Boys enrolled for only Japan tour: 489
- Girls enrolled for only Switzerland tour: 65
Ratio=130+182489+65=312554
To simplify 312554:
1. Find the GCD of 312 and 554.
2. Divide both the numerator and denominator by their GCD.
312÷2554÷2=156277
The ratio of the number of girls enrolled for only Japan tour and the number of boys enrolled for only Switzerland tour together to the number of boys enrolled for only Japan tour and the number of girls enrolled for only Switzerland tour together is 156:277.
Similar Questions
What is the interest rate per annum, if a sum of money invested at compound interest amount to Rs. 2400 in 3 years and in 4 years to Rs. 2,520?
View Solution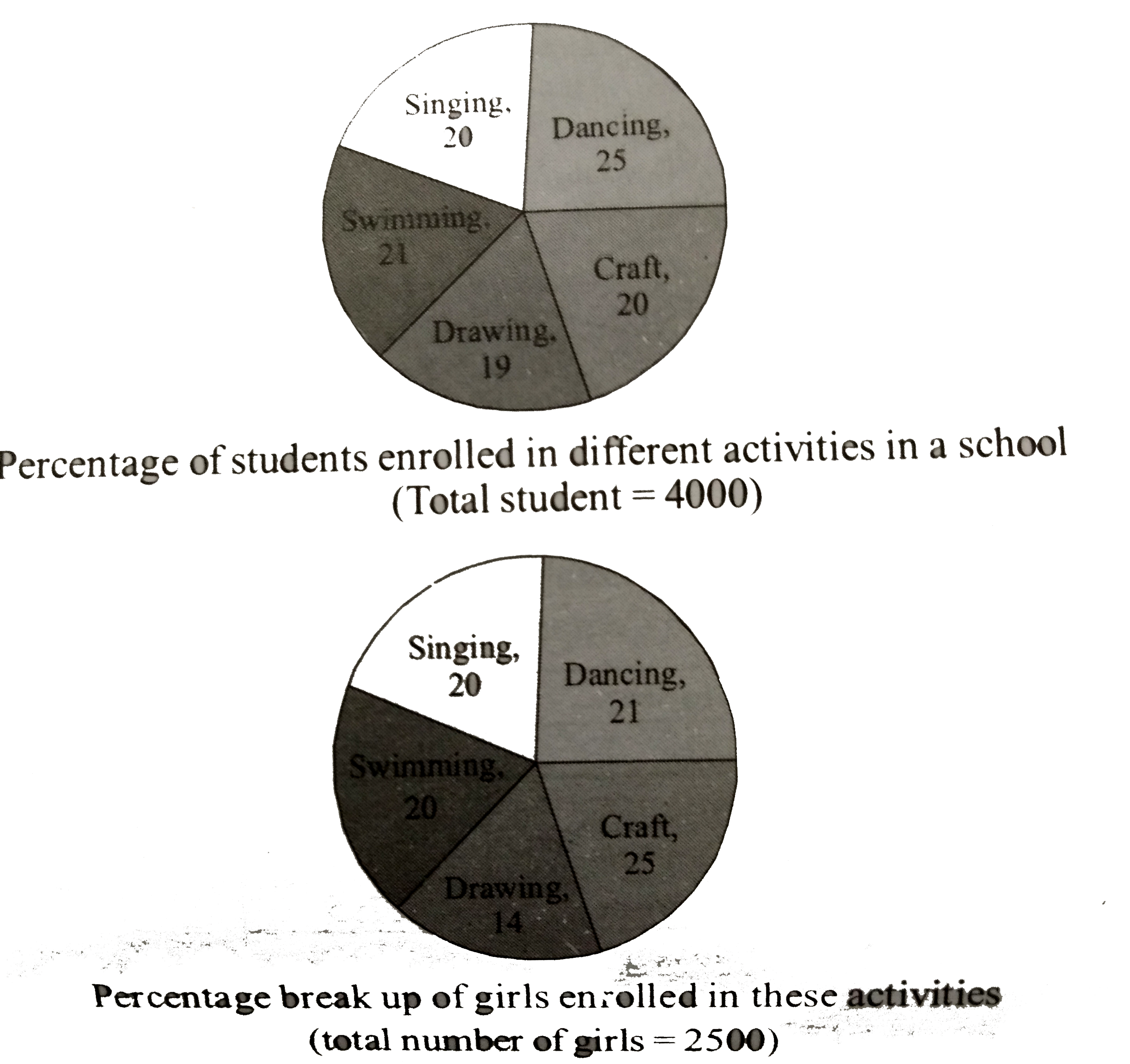
Number of girls enrolled in Dancing is what per cent of total number of students in the school (rounded off to two digits after decimal)
View Solution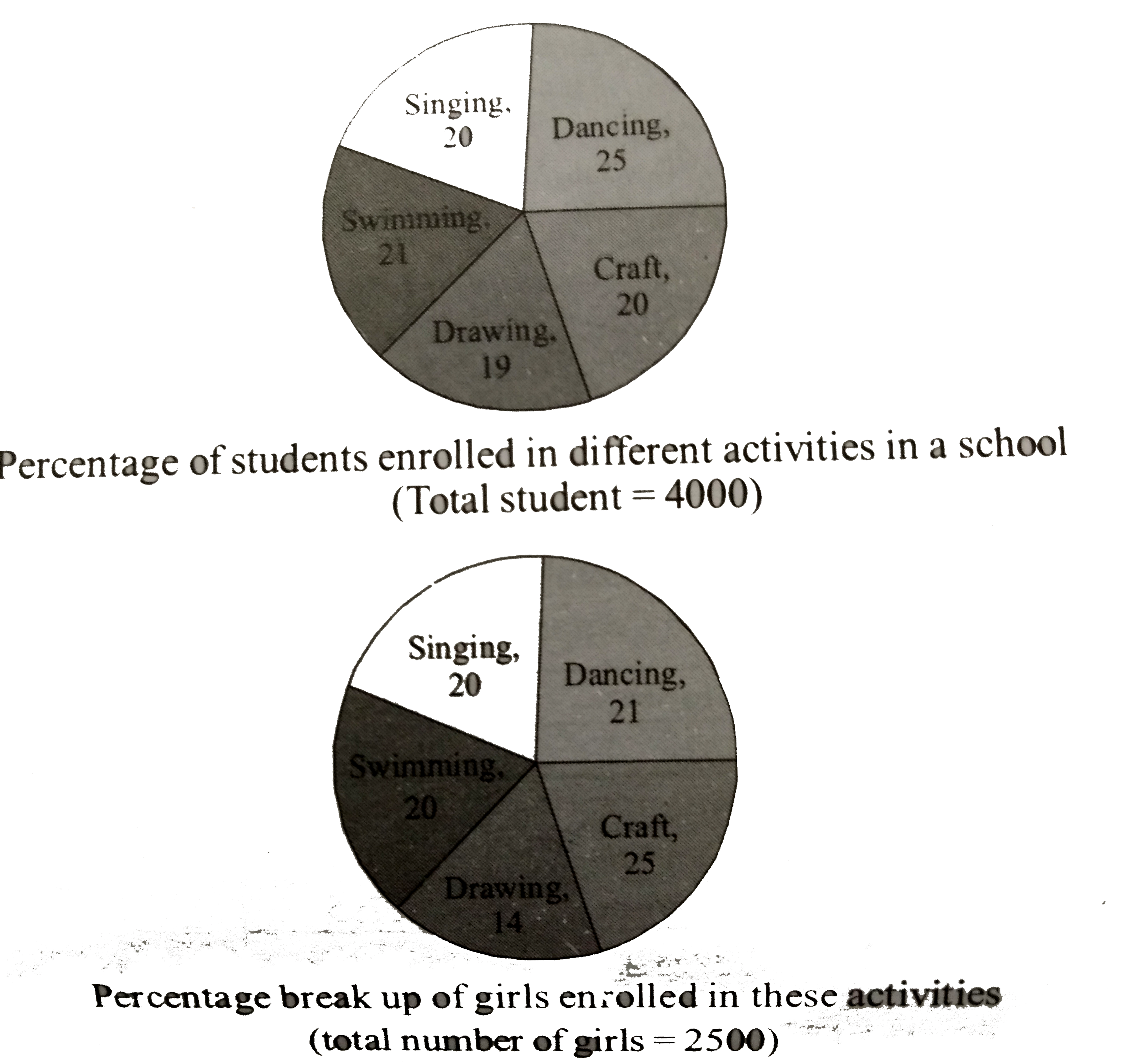
What is the respective ratio of number of girls enrolled in Swimming to the number of boys enrolled in Swimming ?
View Solution432 विद्यार्थियो वाले एक स्कूल में लड़कों और लड़कियों की संख्या का अनुपात 5:4 है। जब स्कूल में कुछ नये लड़के-लड़कियां दाखिल हो जाते हैं तो लड़कों की संख्या 12 और बढ़ जाती है तथा लड़को और लड़कियों से अनुपात बदलकर 7:6 हो जाता है। बताइए कितनी नई लड़कियों ने दाखिला लिया है ?
View SolutionRatio of number of boys and girls in a school with 432 students 5:4 It is when some new boys and girls are enrolled in the school, then the number of boys increases by 12 and the ratio of boys to girls changes 7:6 Lets say, how many new girls have you entered?
View SolutionIn a school with 727 students,600 students are enrolled for
mathematics and 173 students are enrolled in both
mathematics and physics.How many students are enrolled
in Physics only.
View SolutionThe pie-chart given below shows the number of students enrolled in a school in different activities. Total number of students in the school is 1200. Study the chart and answer the questions.

The number of students enrolled in Eco-club is what per cent of those enrolled in Redcross activities ?
View SolutionThe pie-chart given below shows the number of students enrolled in a school in different activities. Total number of students in the school is 1200. Study the chart and answer the questions.

Which two clubs have the enrolment in the ratio of 2:1 ?
View Solution