In each of the following questions, two quantities I and II are given. Compare both the quantities and choose the correct option and give answer accordingly.
Quantity I Value of
Quantity II Value of
Quantity I = Quantity II or No relation can be established
The correct Answer is:A
To solve the problem, we need to compare the two quantities given:
Quantity I: (230−229)/2
Quantity II: 228
Let's break down the calculations step by step.
Step 1: Simplify Quantity I
We start with the expression for Quantity I:
Quantity I=230−2292
Step 2: Factor out common terms
Notice that both terms in the numerator have a common factor of 229:
230−229=229(2−1)=229⋅1=229
Step 3: Substitute back into Quantity I
Now we substitute back into the expression for Quantity I:
Quantity I=2292
Step 4: Simplify further
Dividing 229 by 2 gives us:
Quantity I=229−1=228
Step 5: Compare with Quantity II
Now we can compare Quantity I with Quantity II:
- Quantity I: 228
- Quantity II: 228
Conclusion
Since both quantities are equal:
Quantity I=Quantity II
Thus, the correct option is that Quantity I is equal to Quantity II.
Final Answer
Option 1: Quantity I is equal to Quantity II.
---
Quantity II: 228
- Quantity II: 228
Similar Questions
In the following question, two equations I and II are given. Solve both the equations carefully & answer the questions given below:
I. (x−12)2=0
II. y2=144View SolutionIn the following question, two equations numbered I and II are given. You have to solve both the equations and give the answer:
I. x2−x−12=0
II. y2+5y+6=0View Solution
Knowledge Check
- Question 1 - Select One
In the following questions two equation numbered I and II are given You have to solve both equations and give answer
(i) x2=729
(ii) y=√729Aif x > yBif x ≥ yCif x < yDif x ≤ y - Question 2 - Select One
In the following questions two equations numbered I and II are given. You have to solve both the equations and Give answer
I x2−1=0 II. y2+4y+3=0AIf x>yBIf x≥yCIf x<yDIf x≤y - Question 3 - Select One
In the following question two Quantities i.e., Quantity I and Quantity II are given. You have to determine the relation between Quantity I and Quantity II.
‘a’, ‘b’ and ‘c’ are positive integers.
Quantity I: Value of a in (a+b)2−(a−b)28ab(a+b)2=1
Quantity II: Value of c in (c+b)3−(c−b)3(b2+3c2)2=18bAQuantity I > Quantity IIBQuantity I < Quantity IICQuantity I ≥ Quantity IIDQuantity I ≤ Quantity II
In the following question, two equations numbered I and II are given. You have to solve both the equations and give the answer:
x2–28+3x=0
8y2−y−9=0View SolutionDirections: Given below are two quantities named I and II. Based on the given information, you have to determine the relation between the two quantities. You should use the given data and your knowledge of Mathematics to choose among the possible answers. In the given question, two equations numbered l and II are given. Solve both the equations and mark the appropriate answer.
Quantity I: x - 39x + 378 = 0
Quantity II: y - 24y + 108 = 0View SolutionIn the following questions two quantities are given. You are required to calculate the value in the quantity and compare them. According to the comparison kindly mark your answer.
Quantity I: 5 balls are drawn at random from a bag which contains 10 white, 7 blue and 13 red balls. What is the probability if two balls are red?
Quantity II: 7 marbles are drawn at random from a jar which contains 8 brown marbles, 6 blue marbles and 7 grey marbles. Find the probability if at least 4 marbles are brown.View SolutionMatch the quantities given in List-I to the respective ratios given in List-II.
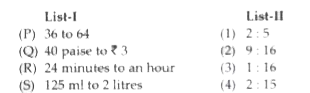 View Solution
View SolutionMatch the quantities of column I with their values in column II and choose the correct option from the codes given below
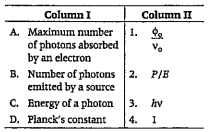 View Solution
View Solution
