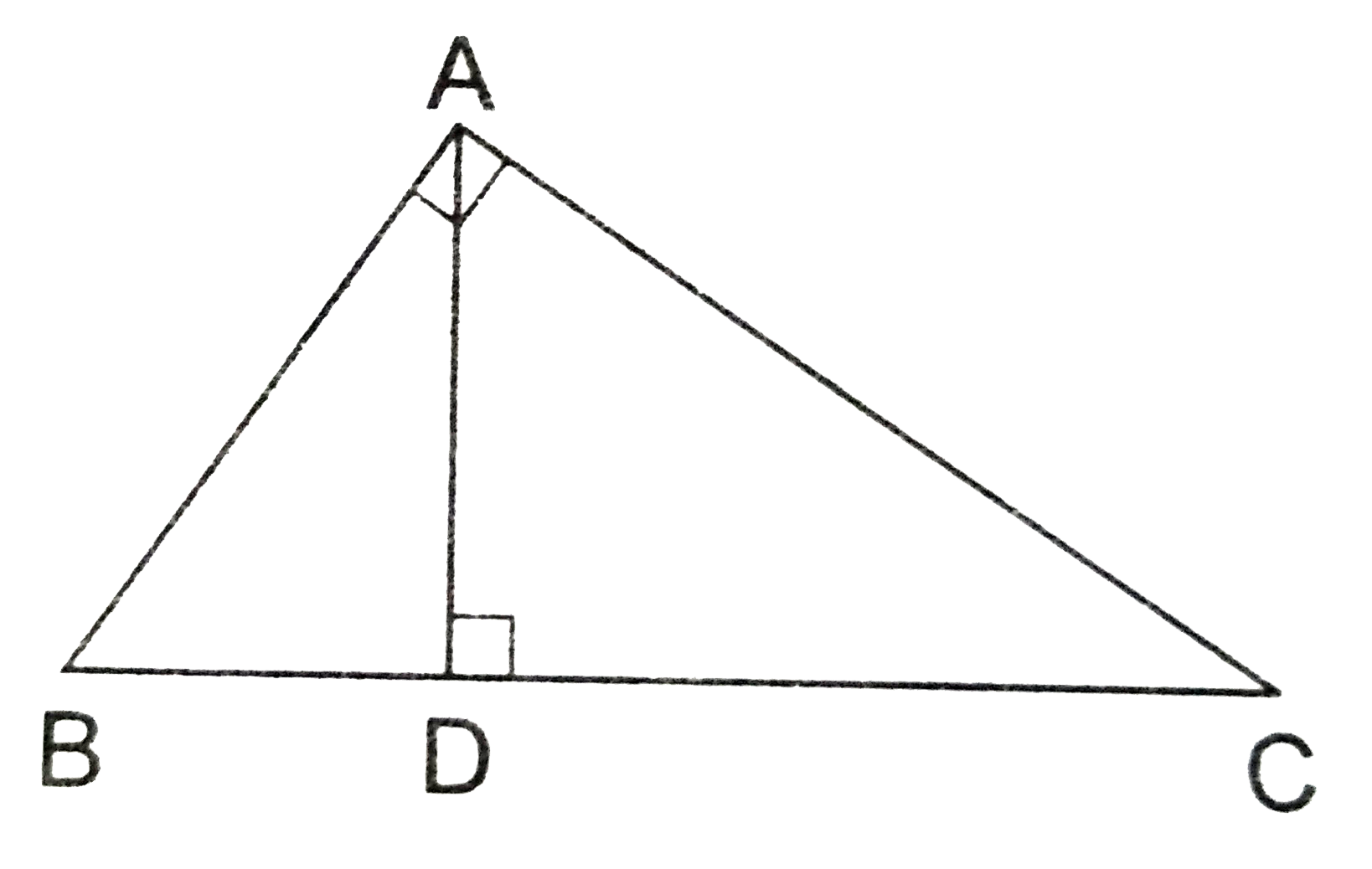
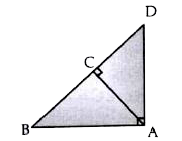
In the figure given below ∠BAC=90∘ and AD ⊥ BC. Then
A
BD.CD=BC2
B
AB.AC=BC2
C
BD.CD=AD2
D
AB.AC=AD2
Video Solution
Text Solution
The correct Answer is:C
|
Answer
Step by step video & text solution for In the figure given below angleBAC=90^(@) and AD bot BC. Then by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
- SAMPLE PAPER 7BOOK - OSWAL PUBLICATIONCHAPTER - SAMPLE PAPER 7EXERCISE - Section - A
7 Videos
- SAMPLE PAPER 7BOOK - OSWAL PUBLICATIONCHAPTER - SAMPLE PAPER 7EXERCISE - Section - B
4 Videos
- SAMPLE PAPER 6BOOK - OSWAL PUBLICATIONCHAPTER - SAMPLE PAPER 6EXERCISE - Section -C
7 Videos
- SAMPLE QUESTION PAPERBOOK - OSWAL PUBLICATIONCHAPTER - SAMPLE QUESTION PAPEREXERCISE - Section -C
5 Videos
Similar Questions
Knowledge Check
In figure, if ∠BAC=90∘ and AD⊥BC. Then,
 ABD.CD=BC2BAB⋅AC=BC2CBD⋅CD=AD2DAB⋅AC=AD2
ABD.CD=BC2BAB⋅AC=BC2CBD⋅CD=AD2DAB⋅AC=AD2In the figure, if ∠BAC=90∘ and AD⊥BC, then:
 ABD.CD=BC2BAB.AC=BC2CBD.CD=AD2DAB.AC=AD2
ABD.CD=BC2BAB.AC=BC2CBD.CD=AD2DAB.AC=AD2
In the given figure ∠ABC=90∘andCD⊥AB. Prove that BC2AC2=BDAD
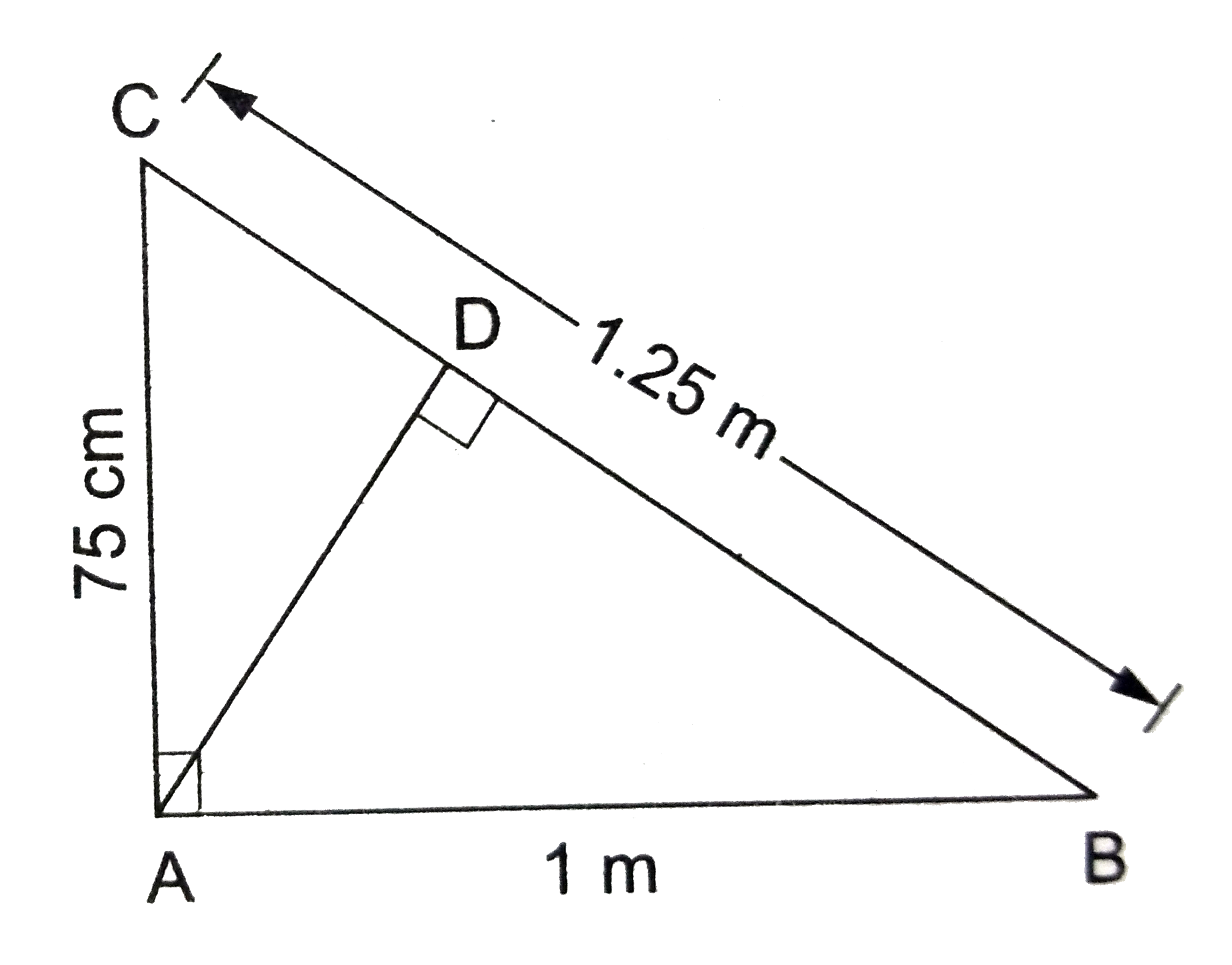
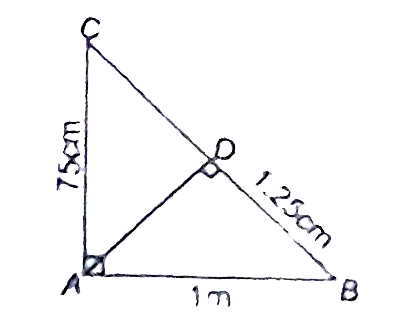
View Solutionin the given figure ∠CAB=90∘andAD⊥BC. if AC= 75 cm , AB= 1m and BD= 1.25 m . Find AD.
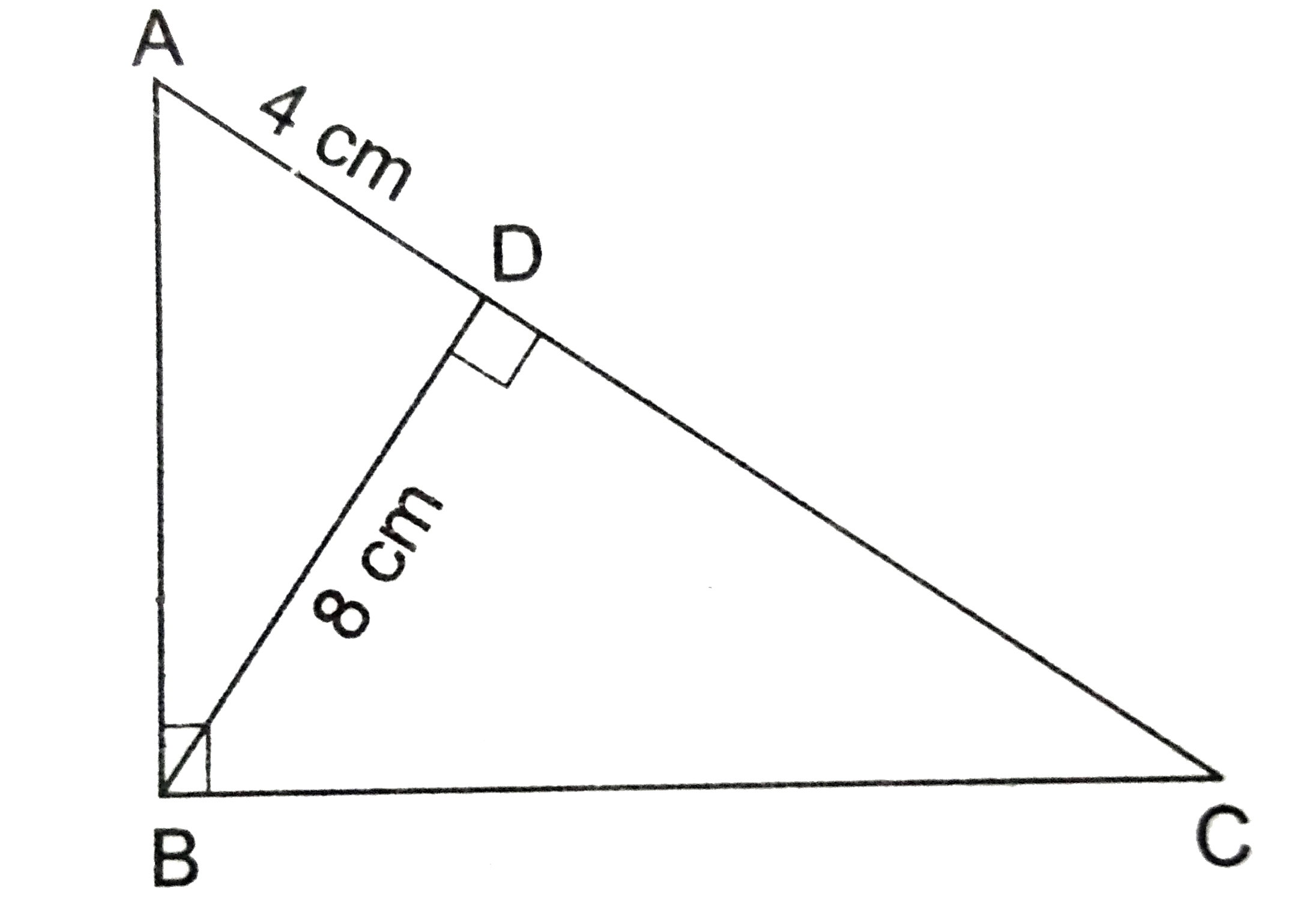
View SolutionIn the given figure, ∠ABC=90∘andBD⊥AC. If BD=8cm,AD=4cm find CD.
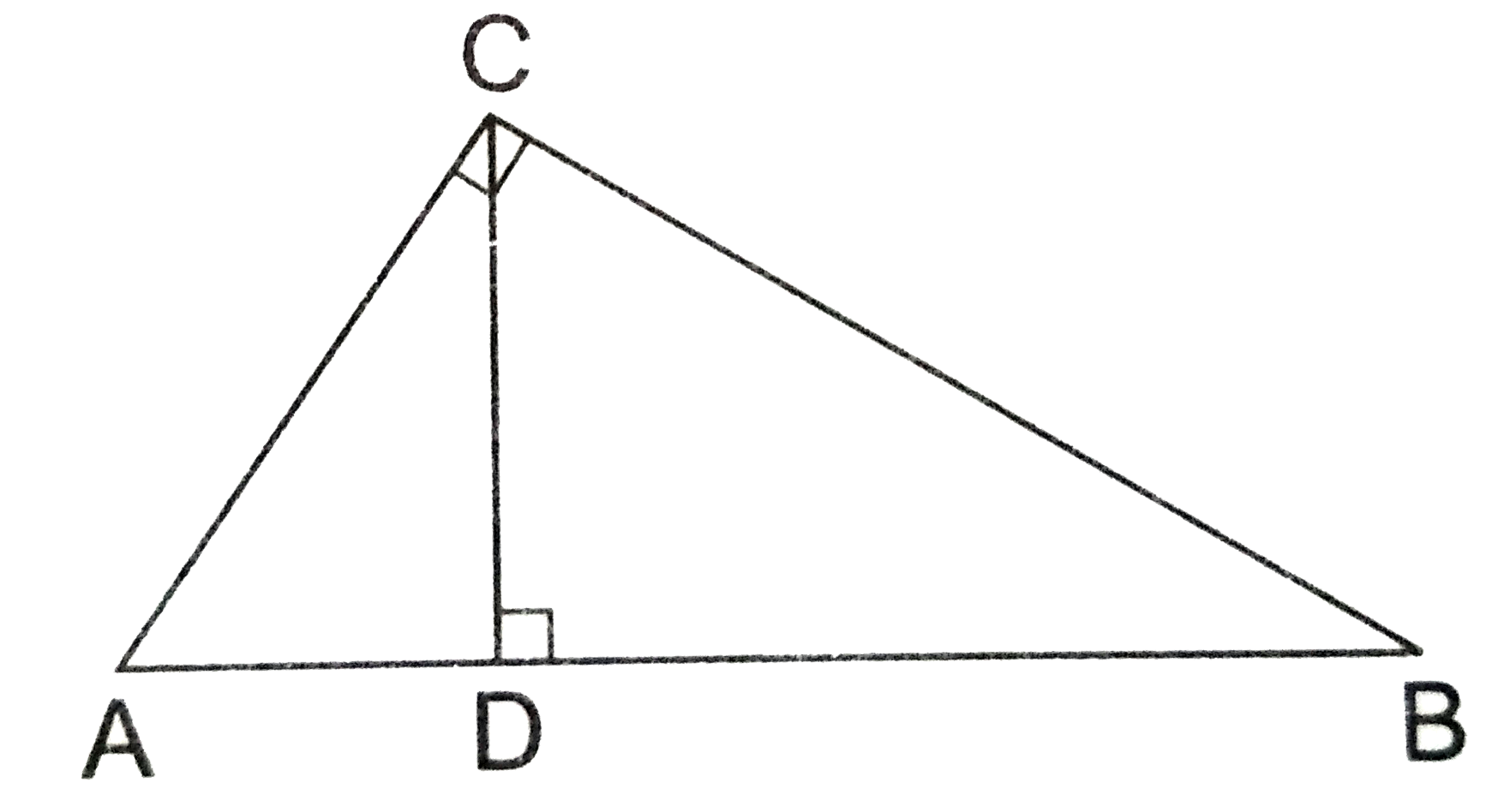
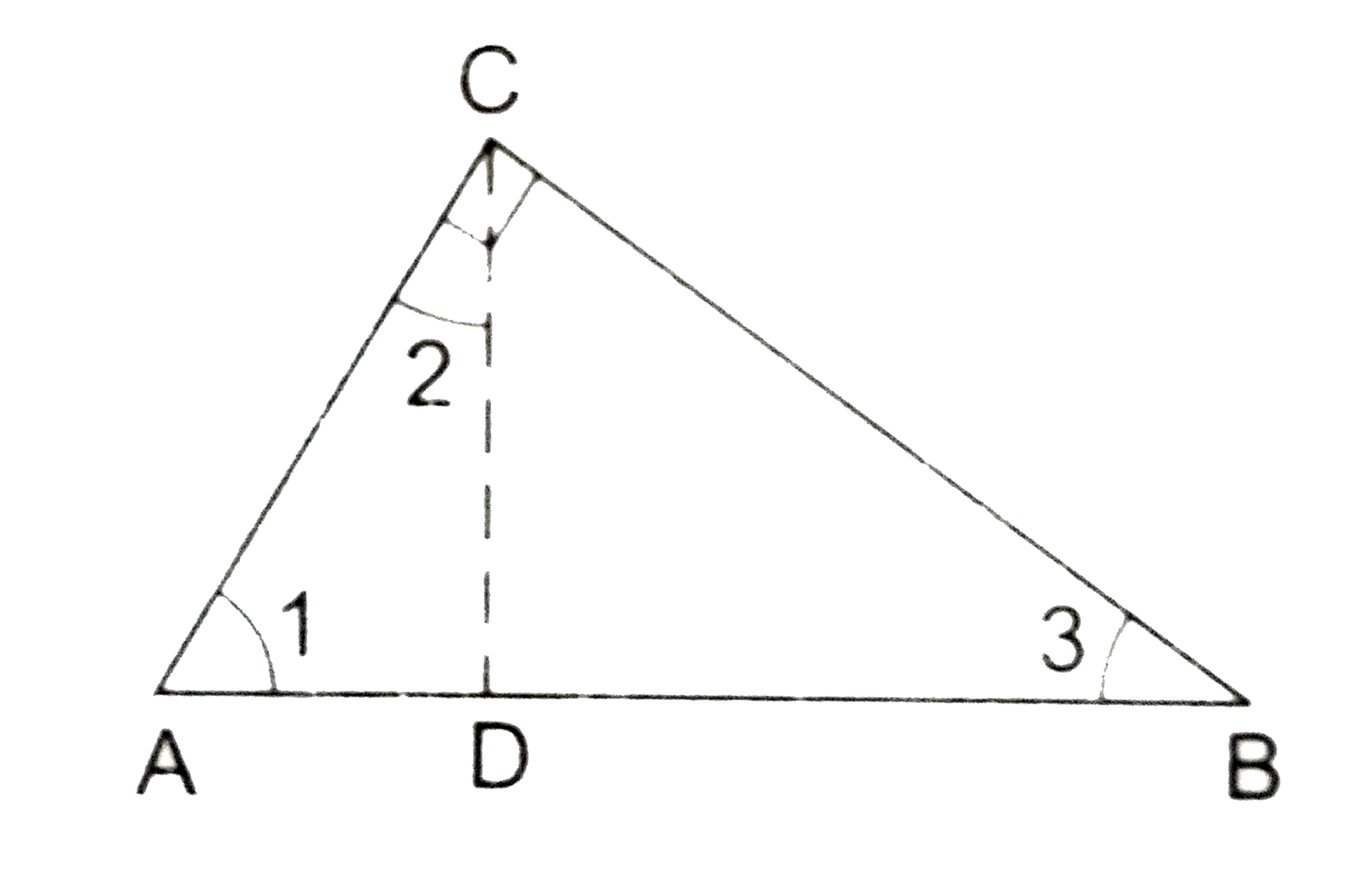
View SolutionIn the given figure, ∠ACB=90∘andCD⊥AB. Prove that CD2=BD⋅AD
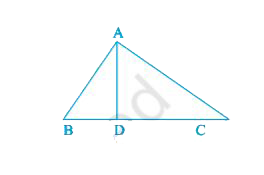
View SolutionIn Fig. 2.12 ∠BAC=90∘ and AD⊥BC.
The number of right triangles in the figure is
View SolutionIn the figure given below ABC is a triangle right angled at A and AC ⊥ CD . Show that
AC2=BC×DC
View Solution