A 20 m long vertical pole casts a shadow 10 m long.At the same time tower makes shadow 50 m long, the tower is _____ long.
75 m
100 m
105 m
120 m
The correct Answer is:B
To find the length of the tower based on the information given about the vertical pole and its shadow, we can use the concept of similar triangles. Here’s a step-by-step solution:
Step 1: Understand the Problem
We have a vertical pole that is 20 m tall and casts a shadow of 10 m. At the same time, a tower casts a shadow of 50 m. We need to find the height of the tower.
Step 2: Set Up the Similar Triangles
The pole and its shadow form a right triangle, and the tower and its shadow form another right triangle. Since the angles of elevation from the tip of the shadow to the top of the pole and the tower are the same (because they are cast at the same time), the two triangles are similar.
Step 3: Write the Proportions
For similar triangles, the ratios of the corresponding sides are equal. Therefore, we can set up the following proportion:
Height of PoleLength of Pole's Shadow=Height of TowerLength of Tower's Shadow
Substituting the known values:
20 m10 m=h50 m
Where h is the height of the tower.
Step 4: Solve for the Height of the Tower
Cross-multiply to solve for h:
20×50=10×h
This simplifies to:
1000=10h
Now, divide both sides by 10:
h=100010=100 m
Conclusion
The height of the tower is 100 m.
---
We have a vertical pole that is 20 m tall and casts a shadow of 10 m. At the same time, a tower casts a shadow of 50 m. We need to find the height of the tower.
The pole and its shadow form a right triangle, and the tower and its shadow form another right triangle. Since the angles of elevation from the tip of the shadow to the top of the pole and the tower are the same (because they are cast at the same time), the two triangles are similar.
For similar triangles, the ratios of the corresponding sides are equal. Therefore, we can set up the following proportion:
Cross-multiply to solve for h:
The height of the tower is 100 m.
Topper's Solved these Questions
- SAMPLE PAPER 5BOOK - OSWAL PUBLICATIONCHAPTER - SAMPLE PAPER 5EXERCISE - Section-A
7 Videos
- SAMPLE PAPER 5BOOK - OSWAL PUBLICATIONCHAPTER - SAMPLE PAPER 5EXERCISE - Section- B
3 Videos
- SAMPLE PAPER 4BOOK - OSWAL PUBLICATIONCHAPTER - SAMPLE PAPER 4EXERCISE - Section -C
8 Videos
- SAMPLE PAPER 6BOOK - OSWAL PUBLICATIONCHAPTER - SAMPLE PAPER 6EXERCISE - Section -C
7 Videos
Similar Questions
A vertical stick 20 m long casts a shadow 10m long on the ground. At the same time, a tower casts a shadow 50m long on the ground. The height of the tower is (a) 100m (b) 120m (c) 25m (d) 200m
View SolutionA tree 6 m tall casts a shadow 4m long. At the same time if a tower casts the shadow 10m long then find the height of the tower.
View Solution
Knowledge Check
A vertical pole 40 m long casts a shadow 20 m long on the ground. At the same time a……….. Tower casts a shadow 50 m long on the ground.
A100mB50mC25 mD150 mA vertical stick 12 m long casts a shadow 8 m long on the ground. At the same time, a tower casts a shadow of 40 m long on the ground. The height of the tower is
A60 mB65 mC70 mD72 m
A vertical stick 10 cm long casts a shadow 8 cm long. At the same time a tower casts a shadow 30m long. Determine the height of the tower.
View SolutionA vertical pole of length 8 m casts a shadow of 15 m long on the ground. At the same time, a tower casts a shadow 45 m long. Find the height of the tower.
View SolutionA vertical stick 12 m long casts a shadow 5 m . Long on the ground. At the same time, a tower casts a shadow 40 m. Long on the ground. What is the height of the tower ?
View SolutionA vertical stick 12m long casts a shadow 8m long on the ground. At the same time a tower casts the shadow 40m long onthe ground. Determine the height of the tower.
View Solution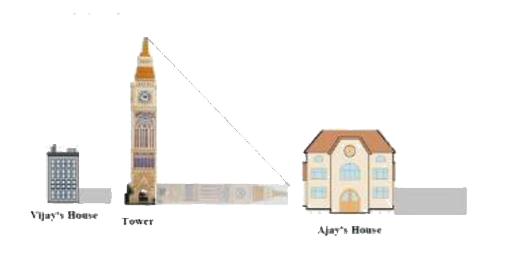
Vijay is trying to find the average height of a tower near his house. He is using the properties of similar triangles.The height of Vijay’s house if 20m when Vijay’s house casts a shadow 10m long on the ground. At the same time, the tower casts a shadow 50m long on the ground and the house of Ajay casts 20m shadow on the ground.
When the tower casts a shadow of 40m, same time what will be the length of the shadow of Ajay’s house?
View Solution
Vijay is trying to find the average height of a tower near his house. He is using the properties of similar triangles.The height of Vijay’s house if 20m when Vijay’s house casts a shadow 10m long on the ground. At the same time, the tower casts a shadow 50m long on the ground and the house of Ajay casts 20m shadow on the ground.
When the tower casts a shadow of 40m, same time what will be the length of the shadow of Vijay’s house?
View Solution
