A missile is fired from the ground level rises x metres vertically upwards in t second, where x=100t−252t2. The maximum height reached is
200m
125 m
160 m
190 m
The correct Answer is:A
Step by step video & text solution for A missile is fired from the ground level rises x metres vertically upwards in t second, where x=100 t-25/2t^(2). The maximum height reached is by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Similar Questions
A stone thrown vertically upwards rises S ft in t seconds where S=112t−16t2. The maximum height reached by the stone is
View SolutionA particle when thrown obliquely from ground, can have a maximum horizontal range 'X'. When it is thrown vertically upwards with the same speed, the maximum height reached by the particle, will be 2 Х 2x √2
View Solution
Knowledge Check
A stone is thrown up vertically and the height x ft it reached in t seconds is x=(80)t−(16)t2. It reached the maximum height in time t=
A2 secondsB3 secondsC2.5 secondsD1.5 secondsA stone thrown down with a seed u takes a time t1 to reach the ground , while another stone, thrown upwards from the same point with the same speed, takes time t2. The maximum height the second stone reaches from the ground is
A.1/2gt1t2Bg/8(t1+t2)2Cg/8(t1−t2)2D.1/2gt22A stone is projected vertically upwards. Its height h at time t sec is h=(80)t−(16)t2. The velocity with which it hits the ground is
A80 units/ secB60 units /secC16 units /secD32 units / sec
A stone thrown vertically upwards satisfies the equations s = 80t–16t2 . The time required to reach the maximum height is
View SolutionA stone thrown upwards from ground level, has its equation of height h=490t−4.9t2where'h' is in metres and t is in seconds respectively. What is the maximum height reached by it ?
View SolutionThe maximum height is reached is 5s by a stone thrown vertically upwards and moving under the equation 10s=10ut−49t2, where s is in metre and t is in second. The value of u is
View SolutionA stone, vertically thrown upward is moving in a line. Its equation of motion is s=294t−49t2, then the maximum height that the stone reaches is
View SolutionSoumya throws a ball upwards, from a rooftop, 80 m above. It will reach a maximum height and then fall back to the ground. The height of the ball from the ground at time 't' is 'h', which is given by h=−16t2+64t+80
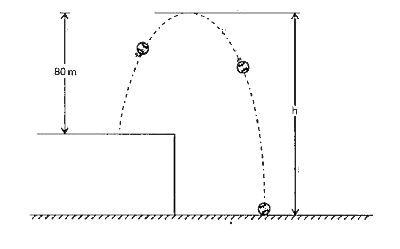
What is the maximum height reached by the ball ?
View Solution
