The specific resistance of manganin is 50×10−8Ω m.
The specific resistance of the combination of two cubes of length 50 m in series will be:
10−6Ωm
2.5×10−5Ωm
2×10−6Ωm
50×10−8Ωm
The correct Answer is:D
To solve the problem, we need to understand the concept of specific resistance (or resistivity) and how it applies to the combination of two cubes of manganin in series.
1. Understanding Specific Resistance:
- The specific resistance (or resistivity) of a material is a property that indicates how strongly the material opposes the flow of electric current. It is denoted by the symbol ρ (rho) and is measured in ohm-meters (Ω·m).
- In this case, the specific resistance of manganin is given as 50×10−8Ωm.
2. Identifying the Configuration:
- The question states that we have two cubes of manganin, each with a length of 50 m, connected in series.
- When resistors are connected in series, the total resistance is the sum of the individual resistances.
3. Calculating the Resistance of Each Cube:
- The resistance R of a cube can be calculated using the formula:
R=ρLA
where:
- R is the resistance,
- ρ is the specific resistance,
- L is the length of the cube,
- A is the cross-sectional area.
- Since we are dealing with cubes, the length L is given as 50 m. However, we need to know the cross-sectional area A to calculate the resistance.
4. Assuming the Cross-Sectional Area:
- For the sake of this problem, let's assume the cross-sectional area A is constant and does not affect the specific resistance given (as it is a property of the material).
- Therefore, the resistance of each cube can be expressed as:
R1=R2=ρ50A
- Since both cubes are identical, they will have the same resistance.
5. Calculating Total Resistance in Series:
- The total resistance Rtotal for two resistors in series is given by:
Rtotal=R1+R2=2R
- Substituting the expression for R:
Rtotal=2(ρ50A)
6. Conclusion:
- However, since the specific resistance of the material does not change regardless of the configuration, the specific resistance of the combination remains the same as that of a single cube:
Specific Resistance of the combination=50×10−8Ωm
Final Answer:
The specific resistance of the combination of two cubes of manganin in series is 50×10−8Ωm.
- The specific resistance (or resistivity) of a material is a property that indicates how strongly the material opposes the flow of electric current. It is denoted by the symbol ρ (rho) and is measured in ohm-meters (Ω·m).
- In this case, the specific resistance of manganin is given as 50×10−8Ωm.
- The question states that we have two cubes of manganin, each with a length of 50 m, connected in series.
- When resistors are connected in series, the total resistance is the sum of the individual resistances.
- The resistance R of a cube can be calculated using the formula:
R=ρLA
where:
- R is the resistance,
- ρ is the specific resistance,
- L is the length of the cube,
- A is the cross-sectional area.
- Since we are dealing with cubes, the length L is given as 50 m. However, we need to know the cross-sectional area A to calculate the resistance.
- For the sake of this problem, let's assume the cross-sectional area A is constant and does not affect the specific resistance given (as it is a property of the material).
- Therefore, the resistance of each cube can be expressed as:
R1=R2=ρ50A
- Since both cubes are identical, they will have the same resistance.
- The total resistance Rtotal for two resistors in series is given by:
Rtotal=R1+R2=2R
- Substituting the expression for R:
Rtotal=2(ρ50A)
- However, since the specific resistance of the material does not change regardless of the configuration, the specific resistance of the combination remains the same as that of a single cube:
Specific Resistance of the combination=50×10−8Ωm
The specific resistance of the combination of two cubes of manganin in series is 50×10−8Ωm.
Similar Questions
If a wire is stretched to four times its length, then the specific resistance of the wire will
View SolutionThe resistance of a heater coil is 110Ω. A resistance R is connected in parallel with it and the combination is joined in series with a resistance of 11Ω to a 220V main line. The heater operates with a power of 110W. The value of R in Ω is
View Solution
Knowledge Check
In the series combination of two or more than two resistances
Athe current through each resistance is sameBthe voltage through each resistance is sameCneither current nor voltage through each resistance is sameDboth current and voltage through each resistance are sameThe circuit shown in the figure contains two diodes each with a forward resistance of 30 Ω and with infinite backward resistance. If the battery is 3 V, the current through the 50 Ω resistance (in ampere) is
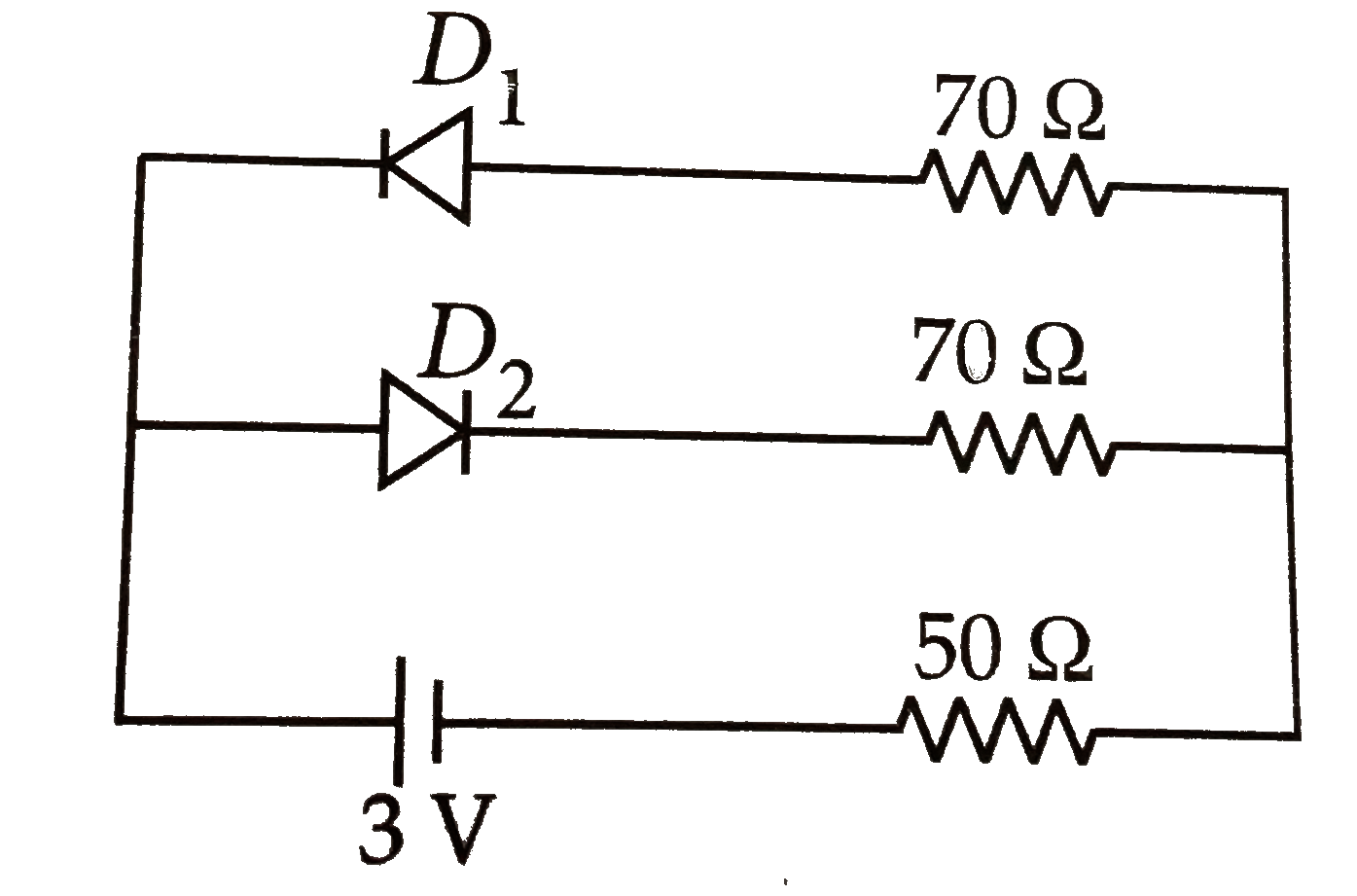 AzeroB0.01C0.02D0.03
AzeroB0.01C0.02D0.03
Resistance of 0.2M solution of an electrolyte is 50Ω. The specific conductance of the solution is 1.3Sm−1. If resistance of the 0.4M solution of the same electrolyte is 260Ω, its molar conductivity is .
View SolutionResistance of 0.2M solution of an electrolyte is 50ohm. The specific conductance of the solution is 1.4Sm−1. The resistance of 0.5M solution of the same electrolyte is 280Ω. The molar conductivity of 0.5M solution of the electrolyte in Sm2mol−1 id
View SolutionA carbon and an aluminium wire are connected in series. If the combination has resistance of 30Ω at 30∘C, what is the resistance of each wire at 0∘Cso that resistance of the combination does not change with temperature.(ac=−0.5×10−3/∘C,aA=4×10−3/∘C)
View SolutionA voltmeter with a resistance 50×103Ω is used to measure voltage in a circuit. To increase its range to 3 times, the additional resistance to be put in series is
View SolutionIf specific resistance of a potentiometer wire is 10–7Ωm, the current flow through it is 0.1A and the cross-sectional area of wire is 10–6m2 then potential gradient will be
View SolutionThe series combination of two batteries, both of the same emf 20 V, but different internal resistance of 10 Ω and 2 Ω , is connected to the parallel combination of two resistors 10 ohm and RΩ . The voltage difference across the battery of internal resistance 10Ω is zero, the value of R(∈Ω) is _________.
View Solution
