Question

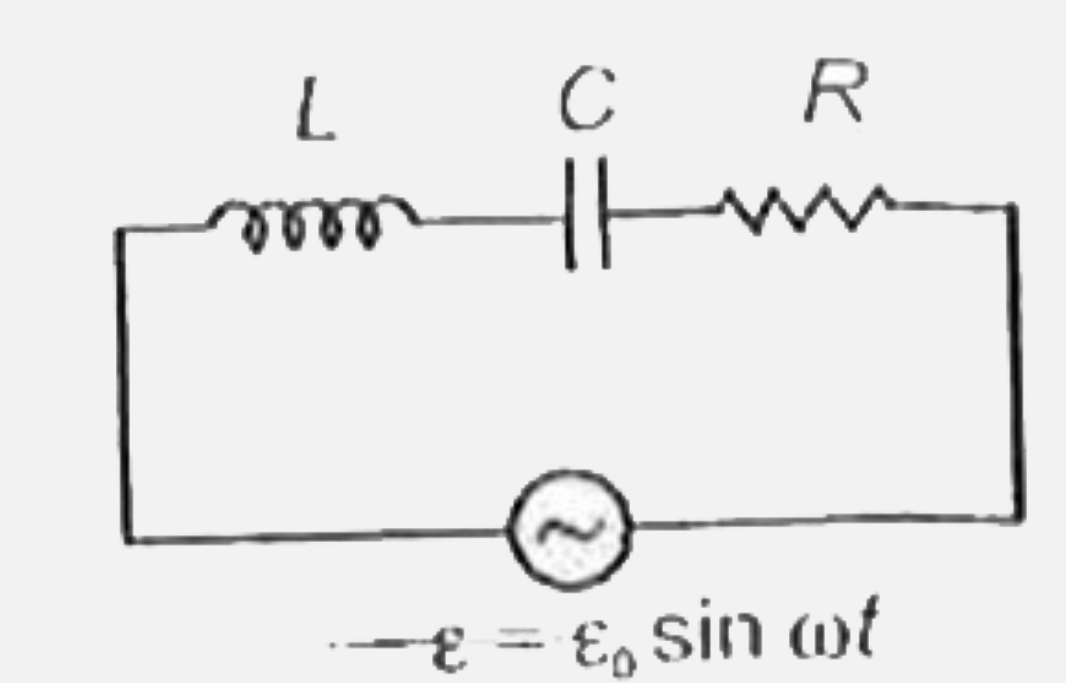
In LCR circuit, the impedance Z is given by $Z=\sqrt{R^2+{(\omega L-\frac{1}{\omega C})}^2}$
The value of Z will be minimum if:

Options

A. `omegaLgt1/(omegaC)`
B. `omegaL=1/(omegaC)`
C. `omegaLlt1/(omegaC)`
D. None of these

Answer

B

Explanation

To find the condition for minimum impedance $$Z$$ in an LCR circuit, we start with the given formula for impedance:

$$Z=\sqrt{R^2+{(\omega L-\frac{1}{\omega C})}^2}$$

Step 1: Understand the Impedance Formula
The impedance \( Z \) consists of two components: the resistance \( R \) and the reactance, which is given by \( \left( \omega L - \frac{1}{\omega C} \right) \). The reactance can be either positive or negative depending on the values of \( \omega L \) and \( \frac{1}{\omega C} \).

Hint: The impedance is minimized when the reactance is zero.

Step 2: Set the Reactance to Zero
To minimize \( Z \), we need the term inside the square root that corresponds to the reactance to be zero:

\( \omega L - \frac{1}{\omega C} = 0 \)

Hint: This equation indicates a balance between the inductive reactance and the capacitive reactance.

Step 3: Solve for \( \omega \)
Rearranging the equation gives us:

\( \omega L = \frac{1}{\omega C} \)

Multiplying both sides by \( \omega \) gives:

\( \omega^2 L C = 1 \)

Hint: This relationship shows how the frequency \( \omega \) relates to the inductance \( L \) and capacitance \( C \).

Step 4: Conclusion
From the equation \( \omega L = \frac{1}{\omega C} \), we can conclude that:

\( \omega L = \frac{1}{\omega C} \implies \omega L = \frac{1}{\omega C} \implies \omega L = \frac{1}{\omega C} \implies \omega L = \frac{1}{\omega C} \)

Thus, the condition for minimum impedance \( Z \) is:

\( \omega L = \frac{1}{\omega C} \)

This means that the correct answer is:

\( \text{Option B: } \omega L = \frac{1}{\omega C} \)

Summary of Steps:
1. Recognize the formula for impedance \( Z \).
2. Set the reactance term to zero for minimization.
3. Solve the resulting equation for \( \omega \).
4. Conclude that the impedance is minimized when \( \omega L = \frac{1}{\omega C} \).