If 1421=x3=6y, then values of x and y are respectively:
2, 9
9, 2
7, 9
2, 7
The correct Answer is:A
To solve the equation 1421=x3=6y, we will break it down into two parts to find the values of x and y.
Step 1: Solve for x
We start with the equation:
1421=x3
To solve for x, we can cross-multiply:
14⋅3=21⋅x
Calculating the left side:
42=21x
Now, divide both sides by 21 to isolate x:
x=4221=2
Step 2: Solve for y
Next, we take the second part of the equation:
1421=6y
Again, we cross-multiply:
14y=21⋅6
Calculating the right side:
14y=126
Now, divide both sides by 14 to isolate y:
y=12614=9
Final Answer
Thus, the values of x and y are respectively:
x=2,y=9
Similar Questions
Knowledge Check
Solve the following system of equations: 2x+3y=9xy, 4x+9y=21xy, where x≠0, y≠0
A0 and 1B1 and 2C2 and 3D1 and 3If 2x+3y=113and5x–7y=313, then the value of x and y are respectively is
A10787,787B10787,−787C16078,−787D17087,−787If 2x+3y=2 and 6x+18y=9, then the values of x and y respectively are:
A3 and 2B2 and 3C4 and 3D3 and 4
Find the values of x and y in the following rectangle :
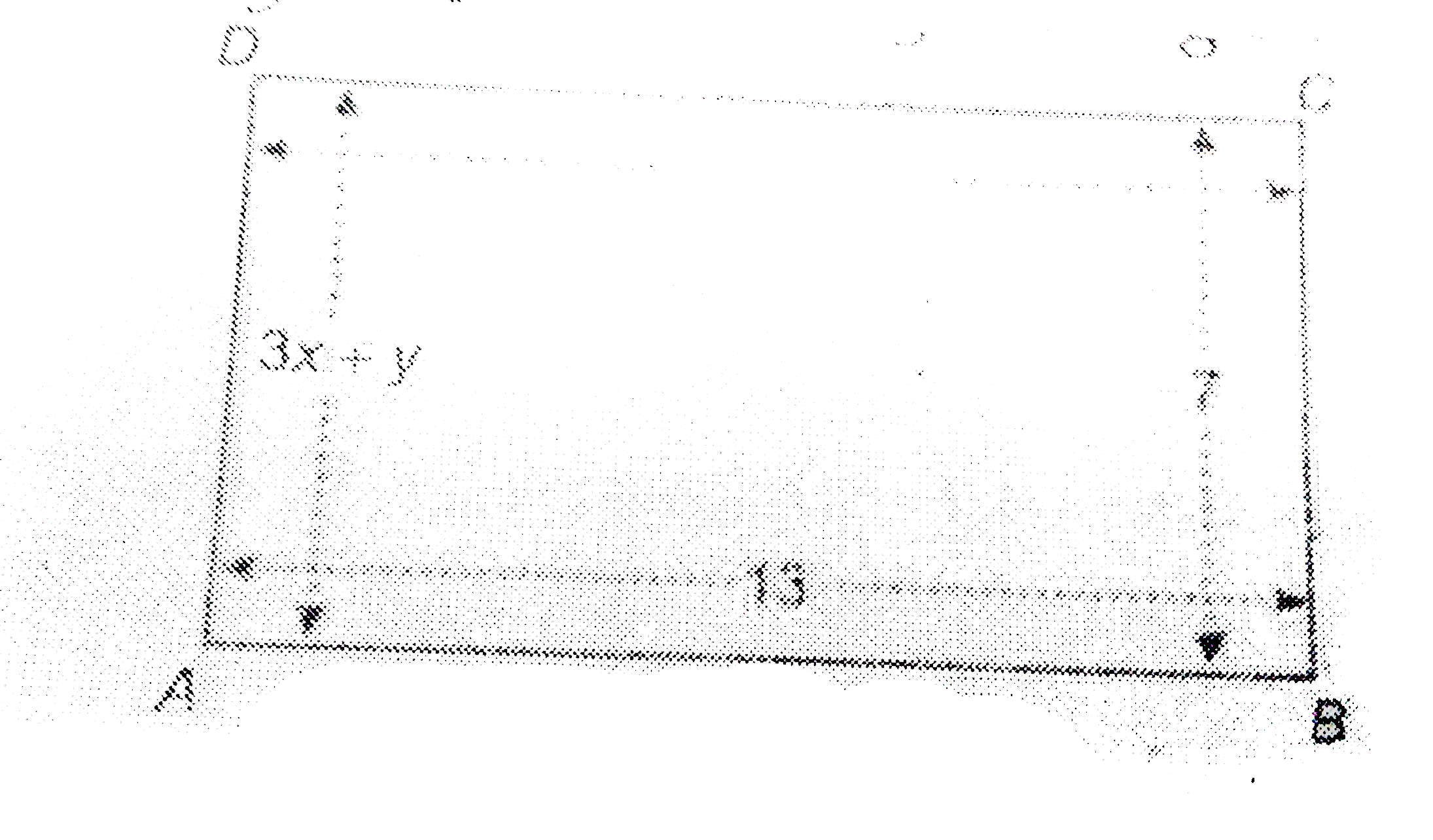
We know that the opposite sides of a rectangle are equal
∴ 3x + y = 7 …(1)
x + 3y = 13 …(2)
9x + 3y = 21 [from (1)]
x + 3y = 13 - - -––––––––––––On subtracting, 8x =8
x = 1
Now, from (1), 3 (1) + y = 7
implies y = 7 - 3
implies y = 4
Hence, the required values of x and y are 1 and 4 respectively.
View SolutionIf the centroid of a triangle formed by (7 , x) , (y , -6) and (9 , 10) is (6 , 3) , then the values of x and y are respectively
View SolutionIf (x-y,x+y)=(2,8), then the values of x and y are respectively.
View SolutionIf x+y−82=x+2y−143=3x+y−1211, then find the values of x and y, respectively.
View SolutionIf [2x30y−1]=[x−3302] then the values of x and y respectively are
View Solution
