The ratio of radii of trajectories of a-particle and proton moving in plane of paper in a region of uniform magnetic field, normal to the plane of paper and having equal linear momentum:
2:1
1:1
2:5
1:2
The correct Answer is:D
To solve the problem of finding the ratio of the radii of the trajectories of an alpha particle and a proton moving in a uniform magnetic field with equal linear momentum, we can follow these steps:
1. Understanding the Motion in a Magnetic Field:
- When a charged particle moves in a magnetic field, it experiences a magnetic force that acts as a centripetal force, causing it to move in a circular path. The radius r of this circular path is given by the formula:
r=mvqB
where:
- m = mass of the particle,
- v = velocity of the particle,
- q = charge of the particle,
- B = magnetic field strength.
2. Equal Linear Momentum:
- We are given that the linear momentum p of both the alpha particle and the proton is equal. Linear momentum is defined as:
p=mv
Therefore, for both particles:
pα=mαvαandpp=mpvp
Since pα=pp, we have:
mαvα=mpvp
3. Expressing Velocity in Terms of Momentum:
- From the momentum equation, we can express the velocities as:
vα=pmαandvp=pmp
4. Substituting Velocity into the Radius Formula:
- Now substituting these expressions for velocity into the radius formula:
rα=mαvαqαB=mα(pmα)qαB=pqαB
rp=mpvpqpB=mp(pmp)qpB=pqpB
5. Finding the Ratio of Radii:
- Now, we can find the ratio of the radii:
rαrp=pqαBpqpB=qpqα
6. Charge of the Particles:
- The charge of a proton qp=e (elementary charge).
- The charge of an alpha particle qα=2e (since it consists of 2 protons).
- Therefore, the ratio becomes:
rαrp=e2e=12
Final Answer:
The ratio of the radii of the trajectories of the alpha particle to the proton is:
rαrp=12
- When a charged particle moves in a magnetic field, it experiences a magnetic force that acts as a centripetal force, causing it to move in a circular path. The radius r of this circular path is given by the formula:
r=mvqB
where:
- m = mass of the particle,
- v = velocity of the particle,
- q = charge of the particle,
- B = magnetic field strength.
- We are given that the linear momentum p of both the alpha particle and the proton is equal. Linear momentum is defined as:
p=mv
Therefore, for both particles:
pα=mαvαandpp=mpvp
Since pα=pp, we have:
mαvα=mpvp
- From the momentum equation, we can express the velocities as:
vα=pmαandvp=pmp
- Now substituting these expressions for velocity into the radius formula:
rα=mαvαqαB=mα(pmα)qαB=pqαB
rp=mpvpqpB=mp(pmp)qpB=pqpB
- Now, we can find the ratio of the radii:
rαrp=pqαBpqpB=qpqα
- The charge of a proton qp=e (elementary charge).
- The charge of an alpha particle qα=2e (since it consists of 2 protons).
- Therefore, the ratio becomes:
rαrp=e2e=12
The ratio of the radii of the trajectories of the alpha particle to the proton is:
rαrp=12
Topper's Solved these Questions
- SAMPLE PAPER 5BOOK - EDUCART PUBLICATIONCHAPTER - SAMPLE PAPER 5EXERCISE - SECTION - B
24 Videos
- SAMPLE PAPER 5BOOK - EDUCART PUBLICATIONCHAPTER - SAMPLE PAPER 5EXERCISE - SECTION - C
6 Videos
- SAMPLE PAPER 3BOOK - EDUCART PUBLICATIONCHAPTER - SAMPLE PAPER 3EXERCISE - SECNTION -C
6 Videos
- SAMPLE PAPER 6BOOK - EDUCART PUBLICATIONCHAPTER - SAMPLE PAPER 6EXERCISE - Section-C
6 Videos
Similar Questions
An α-particle and a proton are moving in the plane of the paper in a region where there is a uniform magnetic field →B directed normal to the plane of the paper. If the two particles have equal linear momenta, what will be the ratio of their trajectories in the field?
View SolutionProtons are shot in a region perpendicular to a uniform magnetic field in a region :
View Solution
Knowledge Check
The ratio of periods of α-particle and proton moving on circular path in uniform magnetic field is _______.
A2:1B1:2C4:1D1:4A charge particle is moving in a plane perpendicular to uniform magnetic field, then
AIts momentum changes but kinetic energy remains the sameBBoth momentum and kinetic energy remain the sameCBoth will changeDKinetic energy change but momentum remains the sameThe counductor AD moves to the right in a uniform magnetic field directed into the plane of the paper.
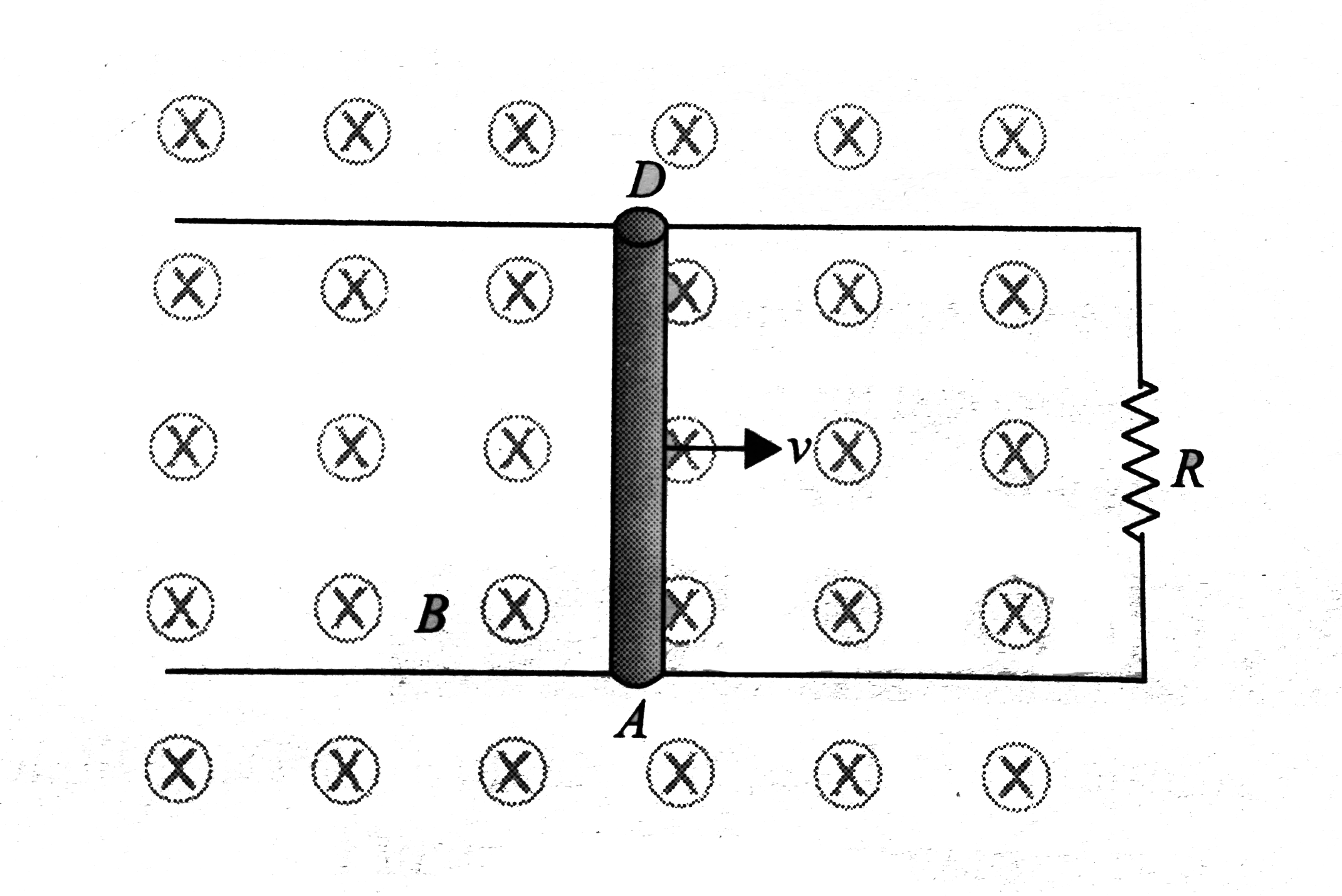 A(a) The free electron in AD will move toward AB(b) D will acquire a positive potential with respect to AC(c ) A current will flow from A→D in AD in closed loopD(d) The current in AD flows from lower to higher potential
A(a) The free electron in AD will move toward AB(b) D will acquire a positive potential with respect to AC(c ) A current will flow from A→D in AD in closed loopD(d) The current in AD flows from lower to higher potential
A charged particle moves through a region of uniform magnetic field perpendicularly. Is the momentum of particle affected ?
View SolutionA charged particle moves through a region of uniform magnetic field perpendicularly, Is the momentum of particle affected?
View SolutionA conducting loop carrying a current I is placed in a uniform magnetic field ponting into the plane of the paper as shown. The loop will have a tendency to

View SolutionAn electron and a proton with equal momentum enter perpendicularly into a uniform magnetic field, then
View SolutionA proton with energy of 2 MeV enters a region of a uniform magnetic field of 2.5 T normally. The magnetic force on the proton is (Take mass of proton to be
View Solution
