In $Δ A B C, D$ is the mid-point of BC and E is the mid-point of AD, then area of $Δ B E D$ is equal to:

$\frac{1}{4}$ x area of $Δ A B C$

$\frac{1}{3}$ x area of $Δ A B C$

$\frac{1}{5}$ x area of $Δ A B C$

$\frac{2}{5}$ x area of $Δ A B C$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

Updated on:21/07/2023

Answer

Step by step video, text & image solution for In DeltaABC,D is the mid-point of BC and E is the mid-point of AD, then area of DeltaBED is equal to: by Maths experts to help you in doubts & scoring excellent marks in Class 14 exams.

Updated on:21/07/2023

Class 14 MATHS QUESTION PAPER 2019

Knowledge Check

In a $Δ A B C, D$ is the mid - point of BC and E is mid - point of AD, BF passes through E. What is the ratio of $A F : F C$ ?

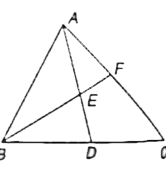A $1 : 1$
B $1 : 2$
C $1 : 3$
D $2 : 3$

In $Δ A B C, D$ is the mid-pointpoint of BC and E is mid-point of $A D . B E$ is produced to meet the side AC in F.Then BF is equal to
View Solution
D is the mid-point of side AB of the triangle ABC.E is the mid-point of CD and F is the mid-point of AE. Prove that $8 \times area of (Δ A F D) = area of Δ A B C$
View Solution
In a $Δ A B C$ , if D is the mid point of BC and AD is perpendicular to AC, then $cos B =$
View Solution
In $Δ A B C$ , D is the midpoint of side BC, E is mid point of AD, F is midpoint of BE and G is mid-point of FC. What part of the area of $Δ A B C$ is the area of $Δ E F G$ ?
View Solution
In parallelogram ABCD, E is the mid-point of AD and F is the mid-point of BC. Prove that BFDE is a parallelogram.
View Solution
$A (a + 1, a - 1), B (a^{2} + 1, a^{2} - 1)$ and $C (a^{3} + 1, a^{3} - 1)$ are given points. D (11, 9) is the mid point of AB and E (41, 39) is the mid point of BC. If F is the mid point of AC then ${(B F)}^{2}$ is equal to $K^{2}$ , where K =
View Solution
in figure , $M$ is the mid-point of $A B$ and $N$ is the mid-point of $B C$ $(1)$ if $A B = B C$ , prove that $A M = N C$
View Solution

In $Δ A B C, D$ is the mid-point of BC and E is the mid-point of AD, then area of $Δ B E D$ is equal to:

The correct Answer is:A

Similar Questions

Knowledge Check

In a $Δ A B C, D$ is the mid - point of BC and E is mid - point of AD, BF passes through E. What is the ratio of $A F : F C$ ?

Recommended Questions

In ΔABC,D is the mid-point of BC and E is the mid-point of AD, then area of ΔBED is equal to:

The correct Answer is:A

Similar Questions

Knowledge Check

In a ΔABC,D is the mid - point of BC and E is mid - point of AD, BF passes through E. What is the ratio of AF:FC?

Recommended Questions

In $Δ A B C, D$ is the mid-point of BC and E is the mid-point of AD, then area of $Δ B E D$ is equal to:

In a $Δ A B C, D$ is the mid - point of BC and E is mid - point of AD, BF passes through E. What is the ratio of $A F : F C$ ?