A steel wire of mass 4.0 g and length 80 cm is fixed at the two ends. The tension in the wire is 50 N. Find the frequency and wavelength of the fourth harmonic of the fundamental. Solution in Gujrati
The correct Answer is:frequency = 250 and λ=40cm
m=(480)gcm
=0.005kgm
T=50N
L=80cm=0.8m
v=√(Tm)
=√(500.005)=100ms
Fundamental frequency
=f=12L√(Tm)
={12×0.8×√(500.005)}
=100(2×0.8)
=1001.6=62.Hz
First =62.5Hz
f4=Frequency of forth harmonic
=4f0=f3=62.5×4
=250Hz
v=f4λ4
→(vf)=100250
=0.4m=40cm
Similar Questions
A steel wire of mass 4.0 g and length 80 cm is fixed at the two ends. The tension in the wire is 50 N. Find the frequency and wavelength of the fourth harmonic of the fundamental.
View SolutionFor one wire tied at both the ends with a tension of 450 N, linear density of mass is 0.05 g/cm. It sounds resonantly for two consecutive frequencies 420 Hz and 490 Hz. Find length of this wire.
View SolutionIf a wire is stretched under tension fixed at both ends. If the radius of wire is halved and tension is doubled the fundamental frequency becomes
View SolutionFundamental frequency of sonometer wire is n. If the length, tension and diameter of wire are tripled the new fundamental frequency is
View SolutionThe frequency of a fundamental tone of a stretched sonometer wire is n. If the length, diameter and tension of the wire is doubled then find the frequency.
View SolutionThe fundamental frequency of a sonometer wire is n . If length tension and diameter of wire are triple then the new fundamental frequency is.
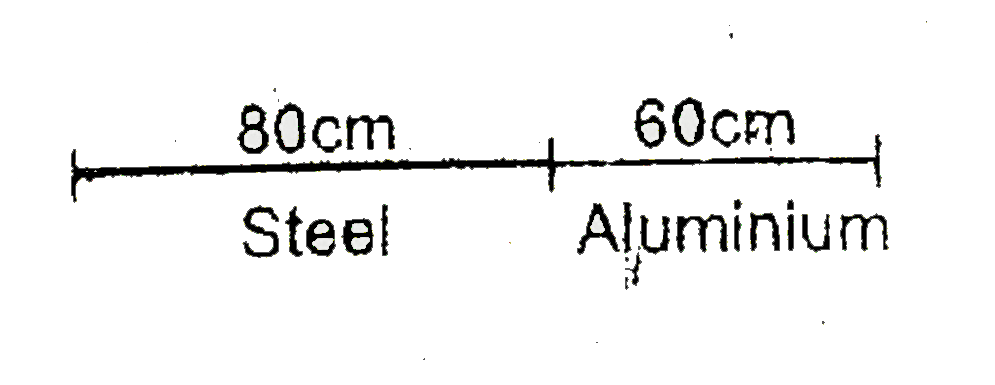
View SolutionFigure shows an aluminium wire of length 60cm joined to a steel wire of length 80cm and stretched between two fixed supports. The tension produced is 40N. The cross-sectional area of the steel wire is 1.0mm2 and that of the aluminimum wire is 3.0mm2 The minimum frequency of a tuning fork which can produce standing waves in the system with the joint as a node is 10P (in Hz) the find P. Given density of aluminimum is 2.6g/cm3 and that of steel is 7.8g/cm3.
View SolutionFundamental frequency of a sonometer wire is n. If
the length and diameter of the wire are doubled
keeping the tension same, then the new fundamental
frequency is
View Solution

