Question

Find the degree of the differential equation ${\left(\frac{dy}{dx}\right)}^2+15\cos x=0$.

a) 4

b) 3

c) 2

d) 1

Answer

D

Explanation

Torque about a point =Total force xx perpendicular distance
From the point to that force
Let anticlockwise torque =+ve
and clockwise acting torque =-ve
Force acting at the point B is 15 N
Therefore
Torque at O due to this force
`=15xx6xx10^-2xxsin37^@`
`=15xx6xx10^-2xx3/5` ltbrge 0.54 N-m (anticlockwise)

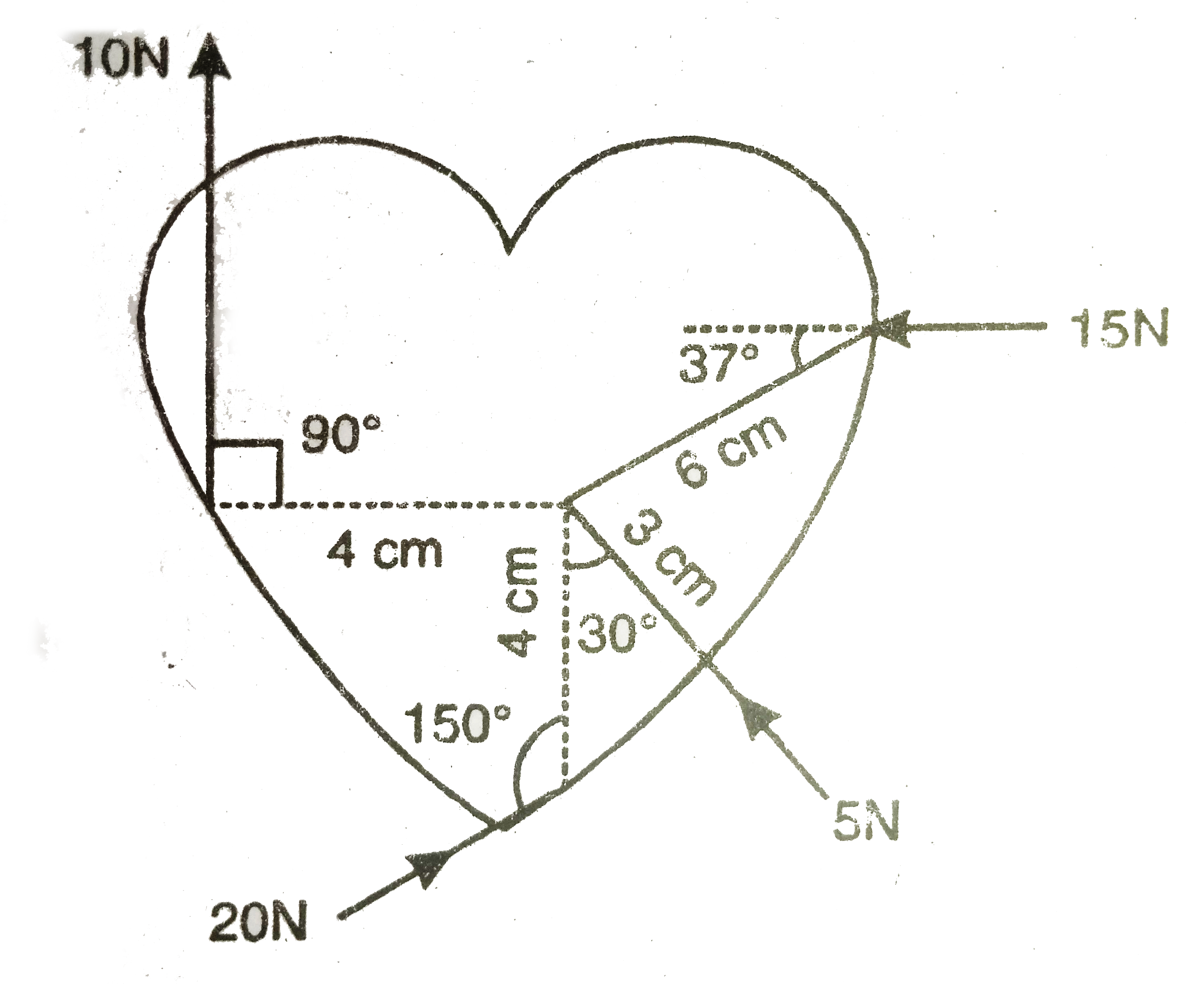
Force acting at the point c is 10 N.
Therefore
Torque at O due to this force
`=10xx4xx10^-2=0.4N-m(clockwise)`
Force acting at the point A is 20 N.
Therefore torque due to this force
`=20xx4xx10^-2sin30^@`
`=20xx4xx10^-2xx1/2`
`=0.4N-m(Anticlockwise)`
Therefore
Resultant torque actint at O
`=(0.54-0.4+0.4)`
`=0.54N-m`