Question

Integrate${\displaystyle \frac{x^2}{e^{x^3}}.}$

${\displaystyle a)–\frac{1}{3e^{x^3}}+C}$

${\displaystyle b)\frac{1}{3}{e}^{x^3}+C}$

${\displaystyle c)–\frac{1}{e^{x^3}}+C}$

${\displaystyle d)e^{x^3}+C}$

Explanation

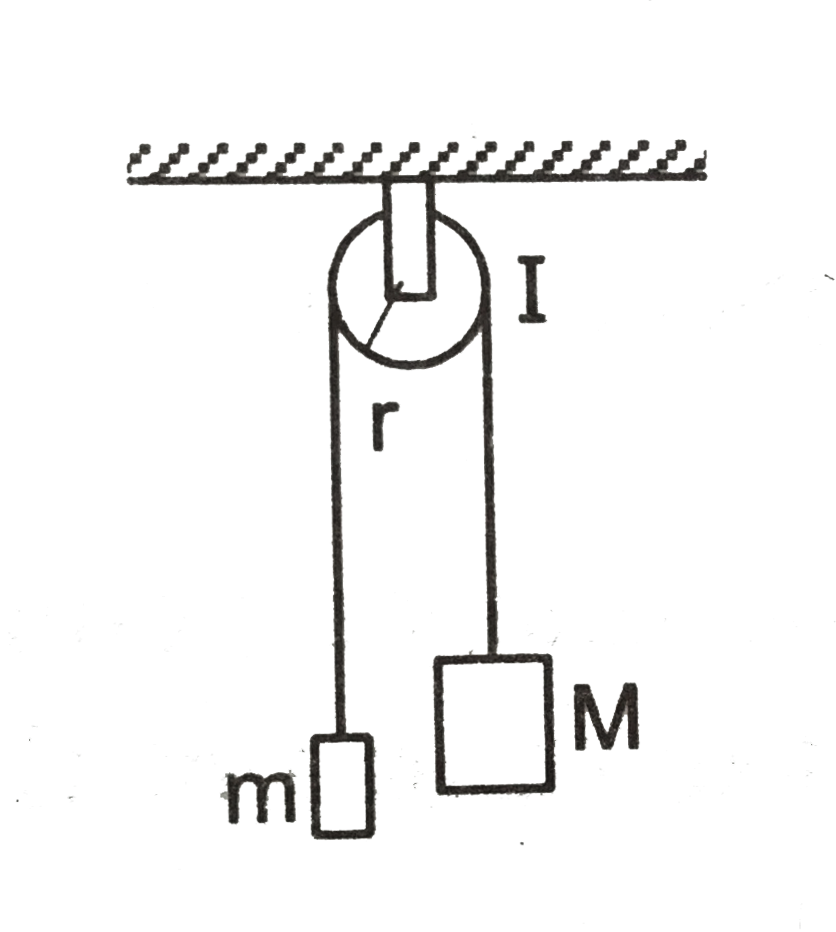
The situation is shown in ure. Let the speed of the masses be v at time t. This will also be the speed of a point on the rim of the wheel and hence the angular velocity of the wheel at time t will be v/r. If the height descended by the mass M is h the loss in the potential energy of the mass plus the pulley system is `mgh-mgh.` The gain in kinetic energy is `1/2Mv^2+1/2mv^2+1/2I(v/r)^2`. An no energy is lost,
`1/2(M=m+1/r^2)v^2=(M-m)gh`
`or, v^2=(2(M-m)gh)/(M+m+1/r^2)`
The angular momentum of the mass M is Mvr and that of the mass is mvr in the same direction. The angular momentum of the pulley is `Iomega=Iv/r.` The total angular momentum is
`[(M+m)r+I/r]v=[(M+m+I/r)r]sqrt((2(M-m)gh)/(M+m+I/r^2)) `
`=sqrt(2(M-m)(M+M+1/r^2)r^2gh)`