What is a vector quantity?
a) A quantity with only magnitude
b) A quantity with only direction
c) A quantity with both magnitude and direction
d) A quantity without directionSolution in Gujrati
The correct Answer is:B, C, D
a. When the particle is released from rest ure the centrifugal force is zero.
N force =mgcosθ=mgcos300
=√32mg
b. When the particle leaves contact with the surface ure N=θ
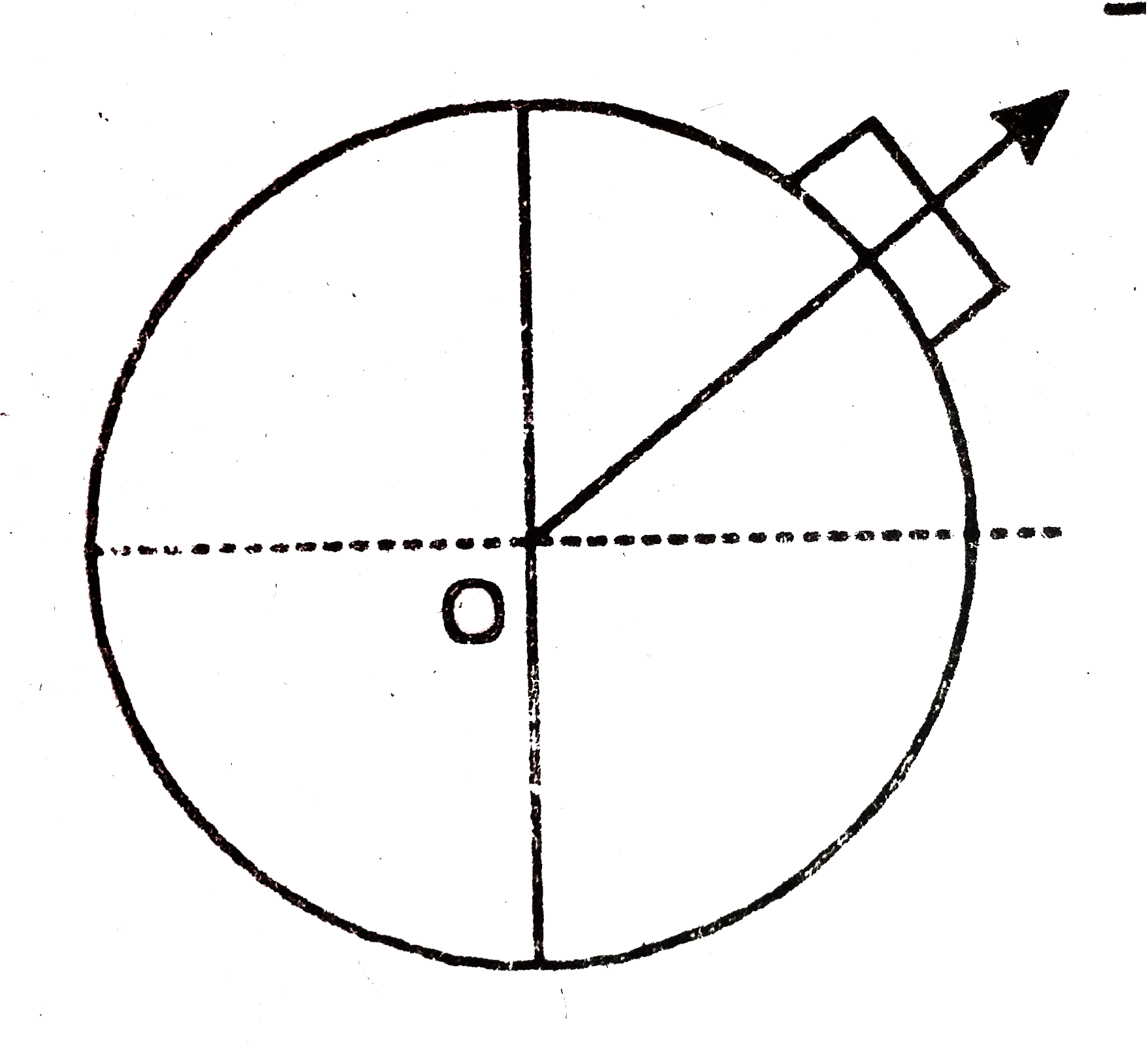
So,mv2R=mgcosθ
→v2=Rcosθ ........i
Aga∈<br>(12)mv2=mgR(cos300−cosθ)
→v2=2rg(√32−cosθ)........ii
omequationiandequationii<br>Rgcostheta=2Rg[sqrt3/2-costheta]<br>rarr 3costheta=sqrt(3)<br>rarr costheta=1/sqrt3<br>or theta=cos^-1 1/sqrt3<br>so,thedistancetravelldbythepartic≤before≤av∈gcontact<br>L=R(theta-pi/6)<br>[∵30^0=(pi/6)]<br>putting the value of theta we get<br>L=0.43R
Similar Questions
A given quantity has both magnitude and direction. It is necessarily a vector.
View SolutionA physical quantity having both magnitude and direction is a vector and if it has only magnitude it is a scalar. Categorize the following physical quantities into scalars and vectors. (a) Force (b) Angular momentum (c) Time (d) Work
View SolutionA quantity has both magnitude and direction. Is it necessarily a vector? Give an example.
View SolutionA quantity which possesses both magnitude and direction _____ a vector.
View SolutionElectric current has both magnitude and direction. Is it a vector quantity?
View Solution"Electric current has both direction and magnitude" - Even though current has both magnitude and direction it is not a vector quantity. Why?
View SolutionI. एक सदिश राशि केवल दिशा द्वारा वर्णित की जा सकती है, जैसे-दाबा
II. एक सदिश राशि परिमाण तथा दिशा दोनों द्वारा वर्णित की जा सकती है, जैसे-वेग।
III. सदिश राशियों के उदाहरण विस्थापन, वेग तथा दाब हैं।
IV. सदिश राशियों के उदाहरण विस्थापन, वेग तथा त्वरण हैं।
उपरोक्त कथनों के आधार पर सही विकल्प का चयन कीजिए।
View SolutionStatement-I : A physical quantity can be regarded as a vector, if magnitude as well as direction is associated with it.
Statement-II : A physical quantity can be regarded as a scalar quantity, if it is associated with magnitude only.
View Solution
