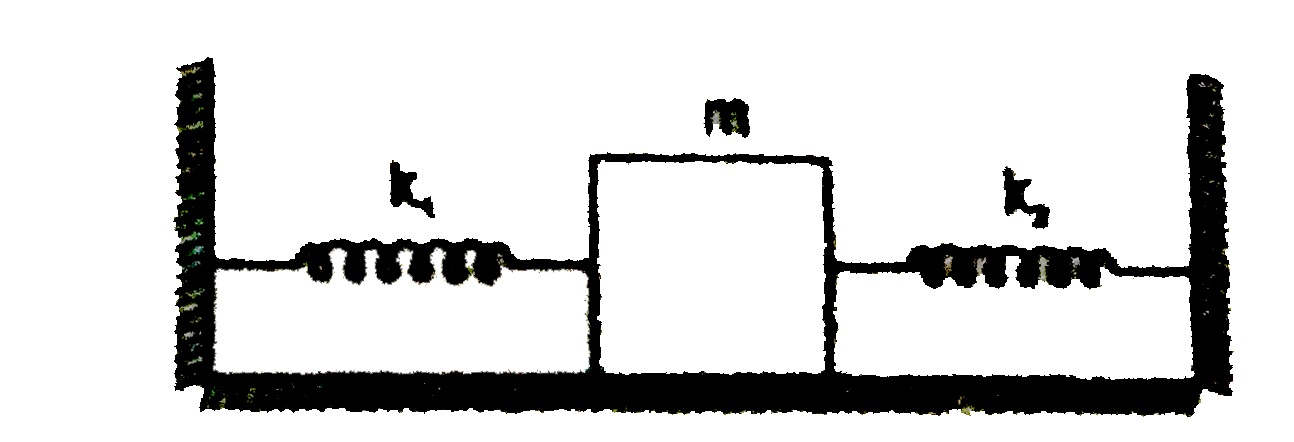
A block of mass m is attached to two unstretched springs of spring constants k1andk2 as shown in figure. The block is displaced towards right through a distance x and is released. Find the speed of the block as it psses through the mean position shown.
 Solution in Gujrati
Solution in Gujrati
The correct Answer is:A, B
The body is displayed x towards righ.
Let the velocity of the body be v at its mean position.
Applying law of conservation of energy
12mv2=12k1x2+12k2x2

→mv2=x2(k1+k2)
→v2=(x2k1+k2m
→v=x√k1+k2m
Similar Questions
A block of mass m is attached to two unstretched springs of spring constant k_(1) and k_(2) as shown in figure. The block is displaced towards right through a distance 'x' and is released. Find the speed of the block as it passes through a distance x//4 from its mean position. All the surfaces are smooth.
View SolutionTwo identical springs of spring constant k are attached to a block of mass m and to fixed supports as shown in figure. When the mass is displaced from equilibrium position by a distance x towards right, find the restoring force.

View SolutionTow identical springs of spring constant k are attached to a block of mass m and to fixed supports as shown in figure. When the mass is displaced from equilibrum position by a distance x towards right, find the restoring force.

View SolutionA block of mass m is attached to two unstretched springs of spring constants k1andk2 as shown in figure. The block is displaced towards right through a distance x and is released. Find the speed of the block as it passes through the mean position shown.

View SolutionA block of mas m is attached to two unstretched sprigs of spring constants k1 and k2 as shown in figure.The block is displaced towards right through a distance x and is released. Find the speed of the block as it passes through the mean positioni?
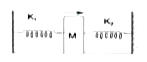
View SolutionA block of mass m is attached to two unstretched springs of springs constant k1 and k2 as shown in figure. The block is displaced towards right through a distance x and is released. Find the speed of the block as it passes through the mean position shown.
View SolutionA block of mass m is attached to two unstretched springs of spring constant k , each as shown. The block is displaced towards right through a distance x and is released The speed of the block as it passes through the mean position will be
View SolutionA block of mass m is attached to four unstretched massless springs of spring constant k_1 and k_2 as shown in figure. The block is displaced towards right through distance x and is released. Speed of block when displacement of block is x/2 from mean position is :
View Solution