A force →F=→v×→A is exerted on a particle in addition to the force of gravity, where →v is the veocity of the particle and →A is a constant vector in the horizontal direction. With what minimum speed a particle of mass m be projected so that it continues to move undeflected with a constant velocity?Solution in Gujrati
When the box accelerating upward
U−mg−mg6
>U=7mgg
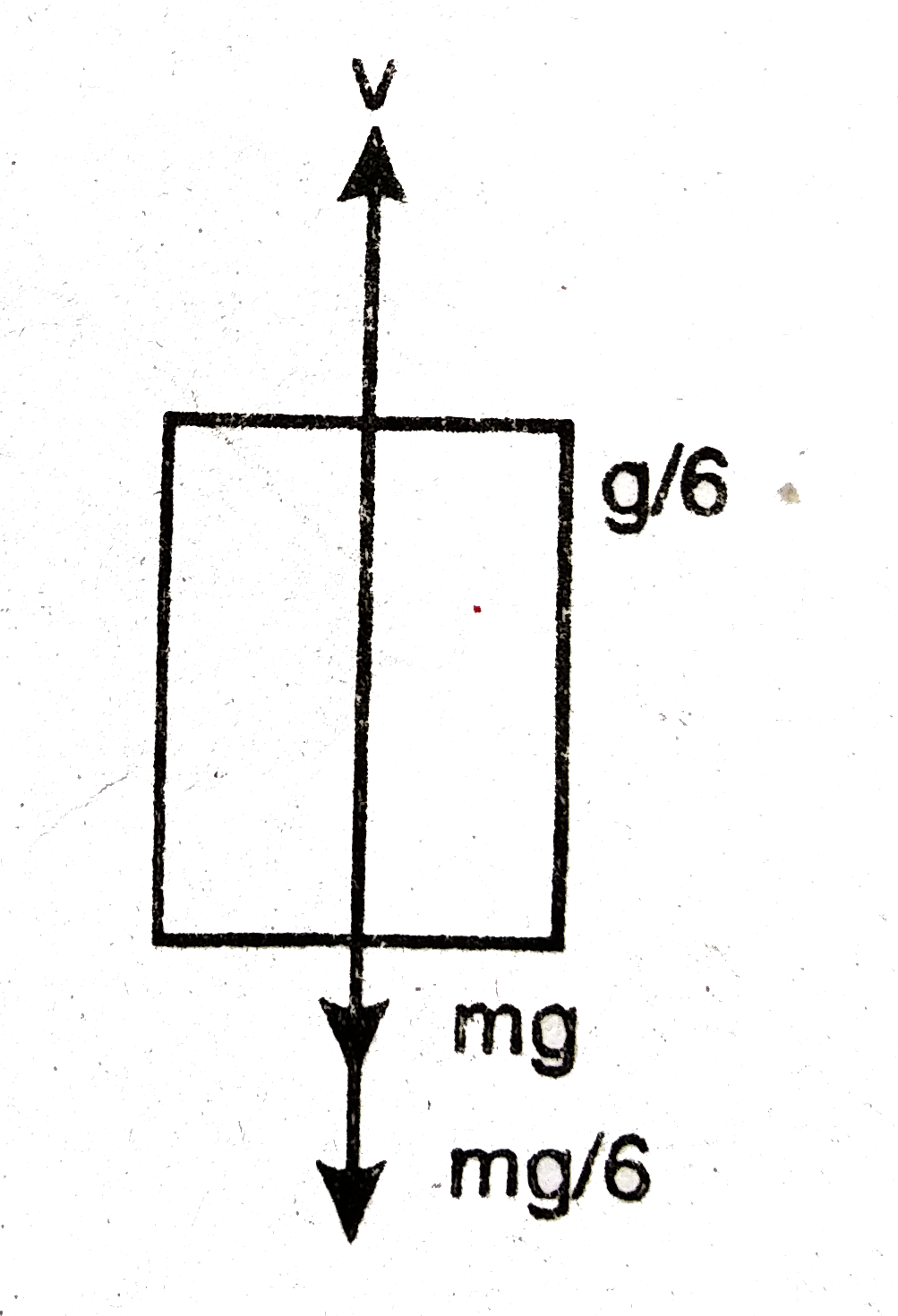
→m=6U7g…i
Mg−U=Mg6
→Mg−Mg6=U
→M=6u5 ……….ii
Massadded=6U5g−6U7g
=6U(7−5)g35g
=6U.2g35g
Fromequationim=6U7h
∴Addedmass=25mg
Similar Questions
Assuming the gravity to be in negative z - direction, a force vec(F)=vec(V)xx vec(A) is exerted on a particle in addition to the force of gravity where vec(V) is the velocity of the particle and vec(A) is a constant vector in positive x - direction. What minimum speed a particle of mass m be projected so that it continues to move underflected with constant velocity ?
View SolutionThe velocity →v of a particle of mass 'm' acted upon by a constant force is given by →v(t)=A[cos(kt)¯i−sin(kt)¯j] . Then the angle between the force and the velocity of the particle is (Here A and k are constants)
View SolutionA force ¯¯¯F=¯¯¯Vׯ¯¯A is exerted on a particle in addition to the force of gravity, where ¯¯¯V is the velocity of the particle and ¯¯¯A is a constant vector in the horizontal direction. The minimum speed of a particle of mass 3kg be projected, so that it continues to move undeflected with a constant velocity is xgA. Then 'x' is (consider the plane of the ground as xy plane)
View SolutionAssuming the gravity to be in negative z - direction, a force vec(F)=vec(V)xx vec(A) is exerted on a particle in addition to the force of gravity where vec(V) is the velocity of the particle and vec(A) is a constant vector in positive x - direction. What minimum speed a particle of mass m be projected so that it continues to move undeflected with constant velocity ?
View Solution40V A constant force vecF is acting on a body of mass m makes it move with constant velocity vecv as shown in the M figure. The power P exerted is
View SolutionForce acting on a particle of mass m moving in straight line varies with the velocity of the particle as F=K//V K is constant then speed of the particle in time t
View SolutionA force →F=(2ˆi+ˆj+ˆk)N is acting on a particle moving with constant velocity →v=(ˆi+2ˆj+ˆk)m/s. Power delivered by force is
View SolutionA force of constant magnitude F acts on a particle moving in a plane such that it is perpendicular to the velocity →v(|→v|=v) of the body and the force is always direct towards a fixed point. Then the angle turned by the velocity vector of the particle as it covers a distance S is (take mass of the particle as m)
View Solution