A heavy particle of mass 0.50 kg is hanging from a string fixed with the roof. Find the force exerted by the string on the particle. Takeg=9.8ms2
 .Solution in Gujrati
.Solution in Gujrati
The correct Answer is:B
The forces acting on the particle are a. pull of the earth 0.50kg×9.8ms2=4.9N vertically downward
b. pull of the string . T vertically upward.
the particle is at rest with respect to the earth (which we assume to be an inertial frame). Hence, the sum of the forces should be zero. Therefore, T is 4.9 N acting vertically upward.
Similar Questions
A 10 kg steel ball is suspended by two strings as shown. The tensions in the two strings are: (g=9.8 m/s2)
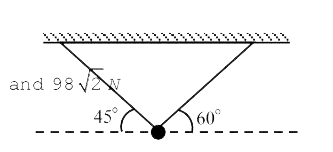 04:51View Solution
04:51View SolutionA heavy particle hanging from a fixed point by a light inextensible string of length l is projected horizonally with speed √gl . Find the speed of the particle and the inclination of the string to the vertical at the instant of the motion when the tension in the string is equal to the weight of the particle.
05:34View SolutionA heavy particle is tied to the end A of a string of length 1.6 m. Its other end O is fixed. It revolves as a conical pendulum with the string making 60^(@) with the vertical. Then (take g = 9.8 m//s )
07:29View SolutionA heavy particle of mass 0.50 kg is hanging from a string fixed with the roof. Find the force exerted by the string on the particle. Take g=9.8ms2
 .02:38View Solution
.02:38View SolutionA heavy particle hanging from a fixed point by a light inextensible string of length l is projected horizonally with speed sqrt(gl) . Find the speed of the particle and the inclination of the string to the vertical at the instant of the motion when the tension in the string is equal to the weight of the particle.
05:24View SolutionA heavy particle hanging from a fixed point by a light inextensible string of length l is projected horizontally with speed (gl) . Then the speed of the particle and the inclination of the string to the vertical at the instant of the motion when the tension in the string equal the weight of the particle-
04:53View SolutionA pendulum bob of mass 50 gm is hanging from the ceiling of a lift by a light , inextensible string. Choose the correct options ( g = 9.8 m/s2)
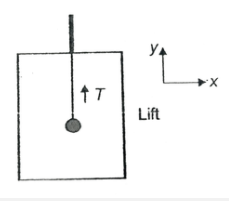 04:29View Solution
04:29View SolutionA heavy particle hanging from a string of length I is projected horizontally with speed sqrt(gl) . Find the speed of the particle at the point where the tension in the string equals weight of the particle.
03:43View Solution
