Each of the following question is followed by two statements I, and Il. You have to study the question and the statements and decide which of the statement(s) is/are necessary to answer the question.
What is the probability of drawing 2 red balls?
Statement I: there are 4 red and 5 blue balls in the bag.
Statement II: there are 20 balls in the bag out of which 5 are yellow and rest are blue & red.
Only Statement I alone.
Only Statement II alone.
Both Statements I and II together.
Neither Statement I nor II is sufficient
The correct Answer is:A
To determine the probability of drawing 2 red balls from a bag, we need to analyze the provided statements.
1. Understanding the Question: We need to find the probability of drawing 2 red balls from a bag. The probability formula is:
P(A)=Number of favorable outcomesTotal outcomes
2. Analyzing Statement I:
- Statement I states that there are 4 red and 5 blue balls in the bag.
- This means the total number of balls in the bag is:
Total balls=4 (red)+5 (blue)=9 balls
- The number of favorable outcomes for drawing 2 red balls can be calculated using combinations:
Favorable outcomes=(42)=4×32×1=6
- The total outcomes for drawing 2 balls from 9 is:
Total outcomes=(92)=9×82×1=36
- Therefore, the probability of drawing 2 red balls is:
P(2 red)=636=16
- Conclusion: Statement I is sufficient to answer the question.
3. Analyzing Statement II:
- Statement II states that there are 20 balls in the bag, out of which 5 are yellow, and the rest are blue and red.
- This means there are 15 balls that are either red or blue, but it does not specify how many are red and how many are blue.
- Without knowing the exact number of red balls, we cannot determine the probability of drawing 2 red balls.
- Conclusion: Statement II is not sufficient to answer the question.
Final Conclusion:
- Only Statement I is sufficient to answer the question about the probability of drawing 2 red balls.
- Therefore, the answer is Option 1: Only Statement I alone is sufficient.
P(A)=Number of favorable outcomesTotal outcomes
- Statement I states that there are 4 red and 5 blue balls in the bag.
- This means the total number of balls in the bag is:
Total balls=4 (red)+5 (blue)=9 balls
- The number of favorable outcomes for drawing 2 red balls can be calculated using combinations:
Favorable outcomes=(42)=4×32×1=6
- The total outcomes for drawing 2 balls from 9 is:
Total outcomes=(92)=9×82×1=36
- Therefore, the probability of drawing 2 red balls is:
P(2 red)=636=16
- Conclusion: Statement I is sufficient to answer the question.
- Statement II states that there are 20 balls in the bag, out of which 5 are yellow, and the rest are blue and red.
- This means there are 15 balls that are either red or blue, but it does not specify how many are red and how many are blue.
- Without knowing the exact number of red balls, we cannot determine the probability of drawing 2 red balls.
- Conclusion: Statement II is not sufficient to answer the question.
- Only Statement I is sufficient to answer the question about the probability of drawing 2 red balls.
- Therefore, the answer is Option 1: Only Statement I alone is sufficient.
Topper's Solved these Questions
- DATA SUFFICIENCY BOOK - ADDA247CHAPTER - DATA SUFFICIENCY EXERCISE - PRELIMS QUESTIONS (LEVEL-2)
42 Videos
- DATA SUFFICIENCY BOOK - ADDA247CHAPTER - DATA SUFFICIENCY EXERCISE - MAINS QUESTIONS
20 Videos
- DATA SUFFICIENCY BOOK - ADDA247CHAPTER - DATA SUFFICIENCY EXERCISE - BASIC QUESTIONS
15 Videos
- DATA INTERPRETATIONBOOK - ADDA247CHAPTER - DATA INTERPRETATIONEXERCISE - Previous Year Questions
56 Videos
- INEQUALITYBOOK - ADDA247CHAPTER - INEQUALITYEXERCISE - Previous Year Questions
75 Videos
Similar Questions
Following are the questions based on two statements and answer the following based on the given statements.
What will be respective ratio of saving of Veer & Deepak.
Statement I. Income of Veer is 4% less than that of Sameer and also expenditure of Veer is 12.5% less than that of Sameer. Deepak spend 35 th of his income.
Statement II. Sameer save Rs. 7000 & Veer save Rs. 7400. Income of Deepak is Rs, 1000 more than that of Sameer,
View SolutionTo answer the following questions, which of the information given in the Statements (A), (B), (C) and (D) or (1), (2), (3) and (4) below is/are necessary/ sufficient?
A certain salesman's yearly income is determined by a base salary plus a commission on the sales he makes during the year. Did the salesman's base salary account for more than half of the salesman's yearly income last year? (Base salary is same for both years)
1. If the amount of the commission had been 30 percent higher, the salesman's income would have been 10 percent higher last year.
2. The difference between the amount of the salesman's base salary and the amount of the last year commission was equal to 50 percent of the salesman's base salary last year.
View SolutionEach question below is followed by two Statements I and II. You have to determine whether the data given in the statements are sufficient for answering the question. You should use the data and your knowledge of Mathematics to choose between the possible answers.
The ages of P and G are in the ratio of 7:5. What is the age of P?
I. The age of P and N are in the ratio of 3:1
II After 7 yr, the ratio of ages of P and G will be 4:3.
View SolutionGiven below pie chart shows percentage distribution of total orders of ice cream purchased by five different shops and table shows percentage of orders of ice cream sold by these five shops. Read the data carefully and answer the questions.
Total orders of ice cream purchased by all shops together = 400
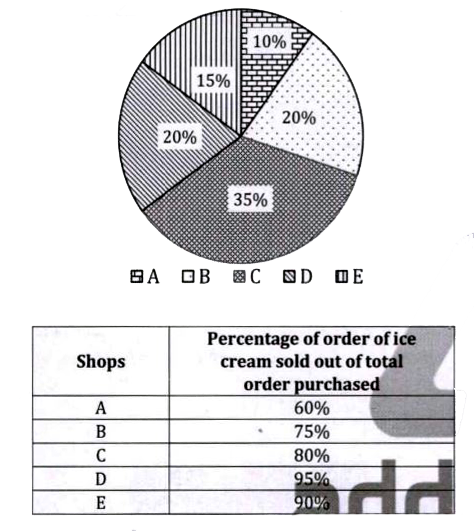
Total unsold order of ice cream by shop E is what percent more than total unsold order of ice cream by shop D?
View SolutionIn each question two equations numbered (I) and (II) are given. You should solve both the equations and mark appropriate answer
I. x2=256
II. y2−17y+16=0
View SolutionA bag contains 5 red balls and some blue balls. If the probability of drawing a blue ball is double that of a red ball, determine the number of blue balls in the bag.
View SolutionA bag contains 5 red balls and some blue balls. If the probability of drawing a blue ball is double that of a red ball, find the number of blue balls in the bag.
View SolutionA bag contains 5 red balls and some blue balls. If the probability of drawing a blue ball is double that of a red ball, find the number of blue balls in the bag.
View SolutionA bag contains 5 red balls and some blue balls, If the probability of drawing a blue ball is double that of a red ball, Find the number of blue balls in the bag.
View SolutionA bag contains 5 red balls and some blue balls. If the probability of drawing a blue ball is double that of a red ball, determine the number of blue balls in the bag.
View SolutionA bag contains 5 red balls and some blue balls. If the probability of drawing a blue ball double that of a red ball determine the number of blue balls in the bag.
View SolutionA bag contains 5 red balls and some blue balls. If the probability of drawing a blue ball is double that of a red ball, determine the number of blue balls in the bag.
View SolutionA bag contains 5 red balls and some blue balls. If the probability of drawing a blue ball is double that of a red ball, determine the number of blue balls in the bag.
View SolutionA bag contains 5 red balls and some blue balls. If the probability of drawing a blue ball is double that of a red ball, determine the number of blue balls in the bag.
View SolutionA bag contains 5 red balls and some blue balls. If the probability of drawing a blue ball is double that of a red ball, determine the number of blue balls in the bag.
View SolutionA bag contains 5 red balls and some blue balls. If the probability of drawing a blue ball is double that of a red ball, determine the number of blue balls in the bag.
View Solution
