Study the information carefully and answer the questions given below.
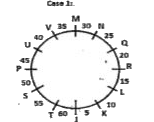
Certain number of persons are sitting around a circular table, which has a circumference of 390 cm. All the persons are facing towards the center. They are sitting at distances to each other which are consecutive multiple of five. Only three persons sits between J and Q. The distance between J and L is 15cm. The distance between J and M is more than 25cm. The distance between L and M is 90cm. The distance between M and U is 75cm. U is not the immediate neighbour of L. U sits on the immediate left of P. Only three persons sits between P and N. N is not the immediate neighbour of J and L. The distance between R and K is 25cm. R sits 4th to the right of T. The distance between V and M is LCM of 7 and 5. S sits third to the right of V.
Who among the following sits 4th to the right of R?
U
P
V
S
The correct Answer is:C
Certain number of persons are sitting around a circular table but not given the number of persons, but from some other information we can conclude the number of persons in the circle. As it is given that all persons are sitting at distances to each other which are consecutive multiple of five and circumference of circle is 390 cm, so between persons distances are 5, 10, 15, 20, 25, 30, 35, 40, 45, 50, 55 and 60. From that condition we can deduce that there are only 12 persons in the circle.
Now, we have to solve the seating arrangement
Only three persons sits between ) and Q. The distance between J and L is 15cm. The distance between J. and M is more than 25cm. The distance between Land M is 90cm.

The distance between M and U is 75cm. U is not the immediate neighbour of L. U sits on the immediate left of P. Only three persons sits between P and N.

N is not the immediate neighbour of J and L. So, from these statement case 2 will be eliminated. The distance between R and K is 25cm. R sits 4th to the right of T. The distance between V and M is LCM of 7 and 5. S sits third to the right of V.
Similar Questions
श्री मोहित ने एक निश्चित दूरी के लिए ट्रेडमिल पर चलने का फैसला किया है। पहले दिन, वह एक निश्चित गति से चलता है। अगले दिन, वह ट्रेडमिल की गति को 1 किमी/घंटा बढ़ाता है, वह 6 मिनट कम लेता है और यदि वह गति को 1 किमी/घंटा कम करता है, तो वह 9 मिनट अधिक लेता है। उसने प्रतिदिन कितनी दूरी चलने का निश्चय किया?
View Solutionएक ठोस की bcc संरचना है । दो निकटतम परमाणुओं के मध्य की दुरी 1.73 Å है । कोष्ठिका की कोर लम्बाई है :
View SolutionA तथा B धातु युक्त द्विअंगी मिश्रधातु के इकाई सेल की ccp संरचना है जिसमे A परमाणु कोनो पर तथा B परमाणु घन के प्रत्येक फलक के केंद्र पर स्थित होते है । यदि इस मिश्रधातु के क्रिस्टलीकृत के दौरान, इकाई सेल में दो A परमाणु लुप्त हो जाते हैं, तब प्रति इकाई सेल का पूर्ण संघटन होगा :
View Solutionयदि a घन के फलक की लम्बाई है, तो घन के अन्त: केंद्रित परमाणु एवं कोने वाले परमाणु के बीच की दुरी होगी :
View Solutionबिन्दुओ (4,3,7) और (1,-1,-5) के बीच की दुरी है
View Solutionप्राथमिक तथा द्वितीयक वाहितमल उपचार के बीच पाए जाने वाले मुख्य अंतर कौन से हैं ?
View SolutionP and Q are the middle points of two chords (not diameters) AB and AC respectively of a circle with centre at a point O. The lines OP and OQ are produced to meet the circle respectively at the points R and S. T is any point on the major arc between the points R and S of the circle. If ∠BAC=32∘,∠RTS = ?
View SolutionLet an be the nth term of an AP, if 100∑r=1a2r=α and 100∑r=1a2r−1=β, then the common difference of the AP is
View Solution