In ∠P:∠Q:∠R=2:2:5. A line parallel to QR is drawn which touches PQ and RT at A and B respectively. What is the value of ∠PBA−∠PAB ?
60
30
24
36
The correct Answer is:A
To solve the problem step by step, we will use the properties of angles in triangles and the concept of parallel lines.
Step 1: Understand the given ratio of angles
We are given that:
angle P:angle Q:angle R=2:2:5
Let us denote the angles as:
angle P=2x,angle Q=2x,angle R=5x
Step 2: Use the angle sum property of triangles
The sum of angles in a triangle is 180 degrees. Therefore, we can write:
angle P+angle Q+angle R=180∘
Substituting the values we have:
2x+2x+5x=180∘
This simplifies to:
9x=180∘
Step 3: Solve for x
To find the value of x, we divide both sides by 9:
x=180∘9=20∘
Step 4: Calculate the angles
Now we can find the individual angles:
angle P=2x=2×20∘=40∘
angle Q=2x=2×20∘=40∘
angle R=5x=5×20∘=100∘
Step 5: Analyze the parallel lines
Since line AB is parallel to line QR, we can use the property of alternate interior angles:
- angle PBA=angle PRQ
- angle PAB=angle PQR
Step 6: Substitute the angles
From our earlier calculations:
angle PBA=angle PRQ=100∘
angle PAB=angle PQR=40∘
Step 7: Calculate angle PBA−angle PAB
Now we can find the difference:
angle PBA−angle PAB=100∘−40∘=60∘
Final Answer
Thus, the value of angle PBA−angle PAB is:
60∘
We are given that:
angle P:angle Q:angle R=2:2:5
Let us denote the angles as:
angle P=2x,angle Q=2x,angle R=5x
The sum of angles in a triangle is 180 degrees. Therefore, we can write:
angle P+angle Q+angle R=180∘
Substituting the values we have:
2x+2x+5x=180∘
This simplifies to:
9x=180∘
To find the value of x, we divide both sides by 9:
x=180∘9=20∘
Now we can find the individual angles:
angle P=2x=2×20∘=40∘
angle Q=2x=2×20∘=40∘
angle R=5x=5×20∘=100∘
Since line AB is parallel to line QR, we can use the property of alternate interior angles:
- angle PBA=angle PRQ
- angle PAB=angle PQR
From our earlier calculations:
angle PBA=angle PRQ=100∘
angle PAB=angle PQR=40∘
Now we can find the difference:
angle PBA−angle PAB=100∘−40∘=60∘
Thus, the value of angle PBA−angle PAB is:
60∘
Similar Questions
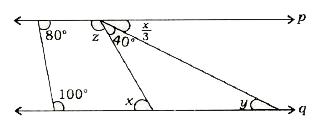
In the figure given above, p and q are parallel lines. What are the values of the angles x, y and z?
View SolutionIn △PQR, ∠Q=84∘,∠R=48∘, PS⊥QR at S and the bisector of ∠P meet QR at T. What is a measure of ∠SPT?
△PQR में,∠Q=84∘,∠R=48∘, PS⊥QR और ∠P का द्विभाजक QR से T पर मिलता है ∠SPT का मान क्या होगा ?
View Solution
Knowledge Check
In ΔPQR,∠P:∠Q:∠R=2:2:5.A line parallel to QR is drawn which touches PQandPR at A and B respectively. What is the value of ∠PBA–∠PAB?
A60B30C24D36In ΔPQR,∠P:∠Q:∠R=1:3:5. What is the value of (in degress) of ∠R−∠P?
A30B80C45D60In a ΔABC∠A:∠B:∠C=2:3:4 A line CD drawn || to AB then the ∠ACD is :
A40°B60°C80°D20°
In a ΔABC,∠A:∠B:∠C=2:3:4 A line CD drawn parallel to AB, then ∠ACD is
View SolutionA circle passing through points Q and R of triangle PQR, cut the sides PQ and PR at point X and Y respectively. If PQ = PR, then what is the value (in degrees) of ∠PRQ+∠QXY ?
View SolutionIf ΔPQRis right angled at Q,PQ=12and∠PRQ=30∘, then what is the value of QR?
View SolutionIf ΔPQRis right angled at Q,PQ=12and∠PRQ=30∘, then what is the value of QR?
View SolutionΔPQR is right angled at point Q. If PQ=8cmand PR=(QR+2)cm. What is the value (in cm) of PR?
View Solution