A body moving in a curved path possesses a velocity 3 m/s towards north at any instant of its motion .After 10s ,the velocity of the body was found to be 4 m/s towards west.Calculate the average acceleration during this interval.
To solve this problem the vector nature of velocity must be taken into account.
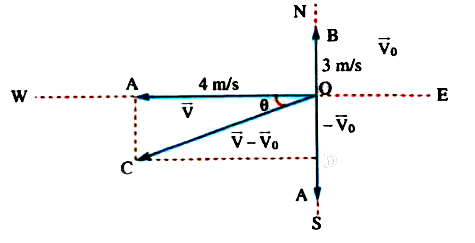
In the figure, the initial velocityv0and the final velocity v arfe drawn from a common origin . The vecto difference of them is found by the parallelogram method.
The magnitude of difference is
|v−v0|=OC=√OA2+AC2
=√42+32=5m/s
The direction is given by
tanθ=34=0.75,θ=370
Average acceleration =|→v−→v0|t=510=0.5m/s2at37∘south of west
Similar Questions
A particle is moving eastwards with velocity of 5m/s. In 10sec the velocity changes to 5m/s northwards. The average acceleration in this time is.
View SolutionA particle is moing with a velocity of 10m/s towards east. After 10s its velocity changes to 10m/s towards north. Its average acceleration is:-
View SolutionA body is moving with velocity 30m/s towards east. After 10s its velocity becomes 40m/s towards north. The average acceleration of the body is.
View SolutionA body is moving with velocity 30m/s towards east. After 10s its velocity becomes 40m/s towards north. The average acceleration of the body is.
View SolutionThe velocity-time graph of an object is given below. Calculate the average acceleration of the object in the time interval of 4 s to 6 s.

View SolutionA boy standing at the top of a tower of 20m. Height drops a stone. Assuming g=10ms−2 towards north. The average acceleration of the body is.
View SolutionA body moves from rest with a uniform acceleration and travels 270 m in 3 s. Find the velocity of the body at 10 s after the start.
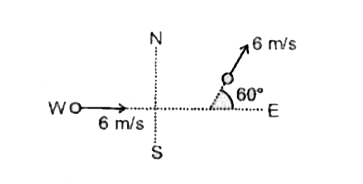
View SolutionA particle is moving eastwards with a speed of 6 m/s. After 6 s, the particle is found to be moving with same speed in a direction 60∘ north of east. The magnitude of average acceleration in this interval of time is
View SolutionFig. 2.38 shows the displacement of a body at different times.
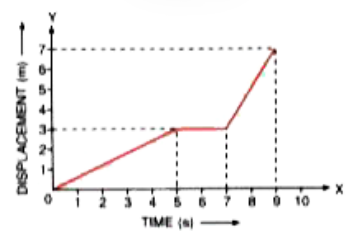
(a) Calculate the velocity of the body as it moves for time interval (i) 0 to 5 s,
(ii) 5 s to 7 S and
(iii) 7 s to 9 s.
(b) Calculate the average velocity during the time interval 5 s to 9 s. [Hint : From 5 s to 9 s, displacement = 7 m - 3 m = 4 m ]
View SolutionA body of mass m1 moving with a velocity 3m/s collides with another body at rest of m2 . After collision the velocities of the two bodies are 2m/s and 5m/s , respectively , along the direction of motion of m1. The ratio m1/m2 is
View Solution
