Two particles of masses m1 and m2 (m1>m2) are separated by a distance d. The shift in the centre of mass when the two particles are interchanged.
(m1+m2)dm1−m2
(m1−m2)dm1+m2
m1dm1−m2
m2dm1−m2
The correct Answer is:B
Step by step video & image solution for Two particles of masses m_(1) and m_(2) (m_(1) gt m_(2)) are separated by a distance d. The shift in the centre of mass when the two particles are interchanged. by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Similar Questions
Two bodies of masses m1andm2(<m1) are connected to the ends of a massless cord and allowed to move as shown in figure. The pulley is both massless and frictionless. The acceleration of the centre of mass is (m1−m2)n(m1+m2)2g. Find the value of n.

View SolutionTwo particles A1andA2 of masses m1,m2(m1>m2) have the same de-broglie wavelength. Then
View Solution
Knowledge Check
Two particles A1andA2 of masses m1,m2(m1>m2) have the same de-broglie wavelength. Then
Atheir momenta are the same.Btheir energies are the same.Cmomentum of A1 is less than the momentum of A2.Denergy of A1 is more than the energy of A2.
Two masses m1 and m2(m1>m2) are falling from the same height when same air resistance acts on them
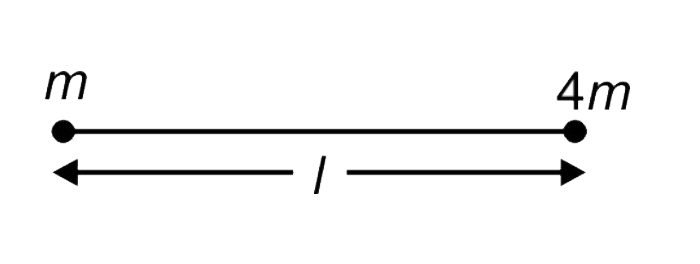
View SolutionTwo particles having masses m and 4m are separated by distance l. The distance of the centre of mass from m is x1andx2 is the distance of point at which gravitational field intensity is zero. Find the value of x1x2
View SolutionTwo massive particles of masses M & m (M>m) are separated by a distance l. They rotate with equal angular velocity under their gravitational attraction. The linear speed of the particle of mass m is
View SolutionTwo masses m1 and m2(m2>m1) are hanging vertically over frictionless pulley. The acceleration of the two masses is :

View SolutionTwo masses m1 and m2(m2>m1) are hanging vertically over frictionless pulley. The acceleration of the two masses is :

View SolutionTwo point particles of mass m and 2m are initially separated by a distance 4a. They are then released to become free to move. Find the velocities of both the particles when the distance between them reduces to a.
View SolutionA mass M is broken into two parts of masses m1 and m2. How are m1 and m2 related so that force of gravitational attraction between the two parts is maximum?
View Solution
