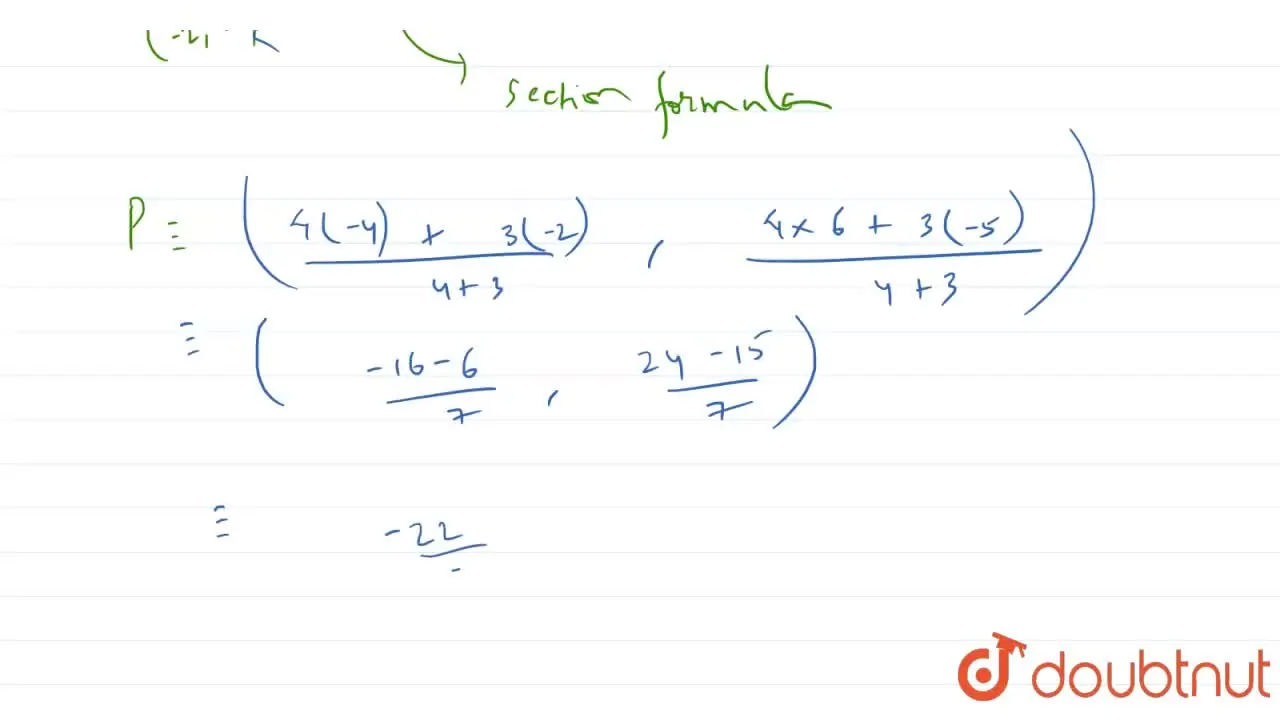
If A=(2,3), B=(-2-5), C=(-4,6) and if P is a point on BC such that AP bisects the angle A, then find P.
The correct Answer is:(−227,97)
Step by step video & image solution for If A=(2,3), B=(-2-5), C=(-4,6) and if P is a point on BC such that AP bisects the angle A, then find P. by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Similar Questions
If A=(2, -3, 7), B = (-1, 4, -5) and P is a point
on the line AB such that AP:BP=3:2 then P
has the coordinatesView SolutionA(4,3),B(6,5)andC(5,−2) are the vertices of a ΔABC, if P is a point on BC such that BP:PC=2:3. Find the co-ordinates of P and then prove then ar (ΔABP):ar(ΔACP)=2:3.
View SolutionGiven a triangle ABC in which A = (4, -4) B = (0,5) and C = (5, 10). A point P lies on BC such that BP : PC = 3 : 2. Find the length of line segment AP.
View SolutionA(1,4)andB(4,8) are two points. P is a point on AB such that AP=AB+BP. If AP=10, find the coordinates of P.
View SolutionThe coordinates of A,B,C are (6,3),(−3,5),(4,−2) , respectively, and P is any point (x,y) . Show that the ratio of the area of PBC to that of ABC is |x+y−2|7.
View SolutionThe coordinates of A,B,C are (6,3),(−3,5),(4,−2) , respectively, and P is any point (x,y) . Show that the ratio of the area of PBC to that of ABC is |x+y−2|7.
View SolutionFind the equation of the plane determined by the points A(3, –1, 2), B(5, 2, 4) and C(–1, –1, 6) and hence find the distance between the plane and the point P(6, 5, 9).
View SolutionFind a, b such that 7.2, a, b, 3 are in A.P.
View SolutionA(3,2,0),B(5,3,2)C(−9,6,−3) are three points forming a triangle. AD, the bisector of angle BAC meets BC in D. Find the coordinates of the point D.
View SolutionThe coordinates of A, B, C are (6, 3), (−3, 5) and (4, −2) respectively and P is any point (x, y) . Show that the ratio of the areas of triangles PBC and ABC is ∣∣∣x+y−27∣∣∣ .
View Solution