Three resistors R1,R2,andR3 , and are connected in series. Find out equivalent resistance of combination.
To find the equivalent resistance of three resistors R1,R2, and R3 connected in series, we can follow these steps:
1. Understand the Series Connection: In a series connection, the same current flows through each resistor. The total voltage across the series combination is the sum of the voltages across each resistor.
2. Write the Voltage Drops: Let the voltage drops across the resistors be V1,V2, and V3. According to Ohm's law, we can express these voltage drops as:
V1=I⋅R1
V2=I⋅R2
V3=I⋅R3
where I is the current flowing through the circuit.
3. Total Voltage: The total voltage V across the series combination is the sum of the individual voltage drops:
V=V1+V2+V3
4. Substituting the Voltage Drops: Substitute the expressions for V1,V2, and V3 into the equation for total voltage:
V=I⋅R1+I⋅R2+I⋅R3
5. Factor Out the Current: Since I is common in all terms, we can factor it out:
V=I(R1+R2+R3)
6. Relate to Equivalent Resistance: According to Ohm's law, the total voltage can also be expressed in terms of the equivalent resistance Req:
V=I⋅Req
7. Set the Equations Equal: Since both expressions equal V, we can set them equal to each other:
I⋅Req=I(R1+R2+R3)
8. Cancel the Current: Assuming I≠0, we can divide both sides by I:
Req=R1+R2+R3
9. Conclusion: The equivalent resistance Req of three resistors connected in series is given by:
Req=R1+R2+R3
V1=I⋅R1
V2=I⋅R2
V3=I⋅R3
where I is the current flowing through the circuit.
V=V1+V2+V3
V=I⋅R1+I⋅R2+I⋅R3
V=I(R1+R2+R3)
V=I⋅Req
I⋅Req=I(R1+R2+R3)
Req=R1+R2+R3
Req=R1+R2+R3
Topper's Solved these Questions
- CURRENT ELECTRICITYBOOK - AAKASH SERIESCHAPTER - CURRENT ELECTRICITYEXERCISE - EXERCISE (SHORT ANSWER QUESTIONS)
12 Videos
- CURRENT ELECTRICITYBOOK - AAKASH SERIESCHAPTER - CURRENT ELECTRICITYEXERCISE - EXERCISE (VERY ANSWER QUESTIONS)
44 Videos
- CURRENT ELECTRICITYBOOK - AAKASH SERIESCHAPTER - CURRENT ELECTRICITYEXERCISE - PROBLEMS (LEVEL-II)
27 Videos
- COMMUNICATION SYSTEMS BOOK - AAKASH SERIESCHAPTER - COMMUNICATION SYSTEMS EXERCISE - PRACTICE EXERCISE (TV Antenna)
16 Videos
- CURRENT ELECTRICITYBOOK - AAKASH SERIESCHAPTER - CURRENT ELECTRICITYEXERCISE - EXERCISE - IA
2 Videos
Similar Questions
A number of resistors R1,R2,R3... are connected in series such that R, is the equivalent resistance of series combination. A current I is flowing in the circuit due to a potential V applied across the circuit. V1,V2,V3.... are potential difference across R1,R2,R3.... respectively. Choose the incorrect statement
View SolutionTwo resistors of 2.092 and 3.0 22 are connected
in series. Find the effective resistance of combination .
View Solution
Knowledge Check
Two resistances R1=100±3ΩandR2=200±4Ω are connected in series . Find the equivalent resistance of the series combination.
A(66.7±1.8)ΩB(66.7±4.0)ΩC(66.7±3.0)ΩD(66.7±7.0)ΩTwo resistors of resistances R1=(300±3)Ω and R2=(500±4)Ω are connected in series. The equivalent resistance of the series combination is
A(800±1)ΩB(800±7)ΩC(200±7)ΩD(200±1)Ω
The resistance 4R,16R,64R....∞ are connected in series. Find their equivalent resistance.
View SolutionTwo resistances R1=100±3ΩandR2=200±4Ω are connected in series . Find the equivalent resistance of the series combination.
View SolutionTwo resistors of resistance R1 and R2 having R1>R2 are connected in parallel. For equivalent resistance R, the correct statement is
View SolutionTwo resistors of resistance R1 and R2 having R1>R2 are connected in parallel. For equivalent resistance R, the correct statement is
View SolutionShow by a diagram how two resistors R1andR2 are joined in parallel. Obtain an expression for the total resistance of the combination.
View SolutionTwo ideal batteries of emf V1andV2 and three resistances R1,R2andR3 are connected as shown in the figure. The current in resistance R2 would be zero if
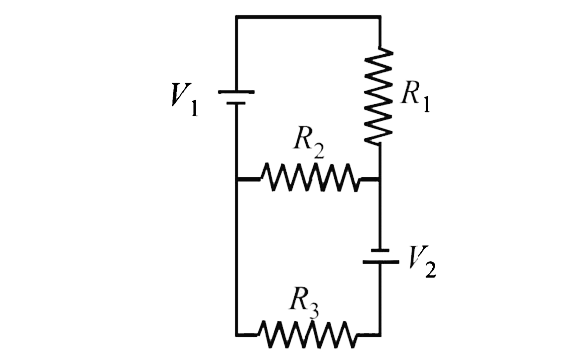
View Solution
