A charge 2C is moved between two points where potentials are 17 and 8V respectively. What is the work done in moving the charge.
The correct Answer is:14 Joules
To find the work done in moving a charge between two points with different electric potentials, we can follow these steps:
1. Identify the Given Values:
- Charge (Q) = 2 C
- Initial potential (V1) = 17 V
- Final potential (V2) = 8 V
2. Calculate the Change in Potential (ΔV):
- The change in potential (ΔV) is given by the formula:
ΔV=Vf−Vi
- Here, Vf is the final potential (V2) and Vi is the initial potential (V1).
- Substituting the values:
ΔV=V2−V1=8V−17V=−9V
3. Use the Work Done Formula:
- The work done (W) in moving a charge in an electric field is given by:
W=Q⋅ΔV
- Substituting the values:
W=2C⋅(−9V)
4. Calculate the Work Done:
- Now, calculate the work done:
W=2⋅(−9)=−18 Joules
5. Final Answer:
- The work done in moving the charge is −18 Joules.
- Charge (Q) = 2 C
- Initial potential (V1) = 17 V
- Final potential (V2) = 8 V
- The change in potential (ΔV) is given by the formula:
ΔV=Vf−Vi
- Here, Vf is the final potential (V2) and Vi is the initial potential (V1).
- Substituting the values:
ΔV=V2−V1=8V−17V=−9V
- The work done (W) in moving a charge in an electric field is given by:
W=Q⋅ΔV
- Substituting the values:
W=2C⋅(−9V)
- Now, calculate the work done:
W=2⋅(−9)=−18 Joules
- The work done in moving the charge is −18 Joules.
Topper's Solved these Questions
- ELECTRIC FIELD AND POTENTIALBOOK - AAKASH SERIESCHAPTER - ELECTRIC FIELD AND POTENTIALEXERCISE - PROBLEMS (LEVEL-II)
26 Videos
- ELECTRIC FIELD AND POTENTIALBOOK - AAKASH SERIESCHAPTER - ELECTRIC FIELD AND POTENTIALEXERCISE - EXERCISE (VERY SHORT ANSWER QUESTIONS)
23 Videos
- ELECTRIC CHARGES AND FIELDSBOOK - AAKASH SERIESCHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - Practice Exercise
57 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - AAKASH SERIESCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Additional Exercise
11 Videos
Similar Questions
(i) Can two equaipotential surfaces intersect each other ? Give reasons
(ii) Two charges −qand+q are located at point A (0, 0 -a) and B(0, 0, +a) respectively. How much work is done in moving a test charge from point P(7,0,0) to Q(−3,0,0) ?
View SolutionTwo points P and Q are maintained at the potentials of 10V and -4V, respectively. The work done in moving 100 electrons from P to Q is:
View SolutionA uniform electric field E exists between two oppositely charged plates (Fig. 3.38). What will be the work done in moving a charge q along a closed rectangular path ?
 .
.
View SolutionA charge (−q) and another charge (Q) are kept at two points AandB respectively. Keeping the charge (+Q) fixed at B, the charge (−q) at A is moved to another point C such that ABC forms an equilateral triangle of side l. The net work done in moving teh charge (−q) is
View SolutionExplain the concept of electric potential difference in terms of work done in transferring the charge.
View SolutionOn moving a charge of 2C from a point A where potential is +12V to a point B where potential is -12 V, the work done is -
View SolutionFigures (a) and (b) show the field lines of a positive and negative point charge respectively.
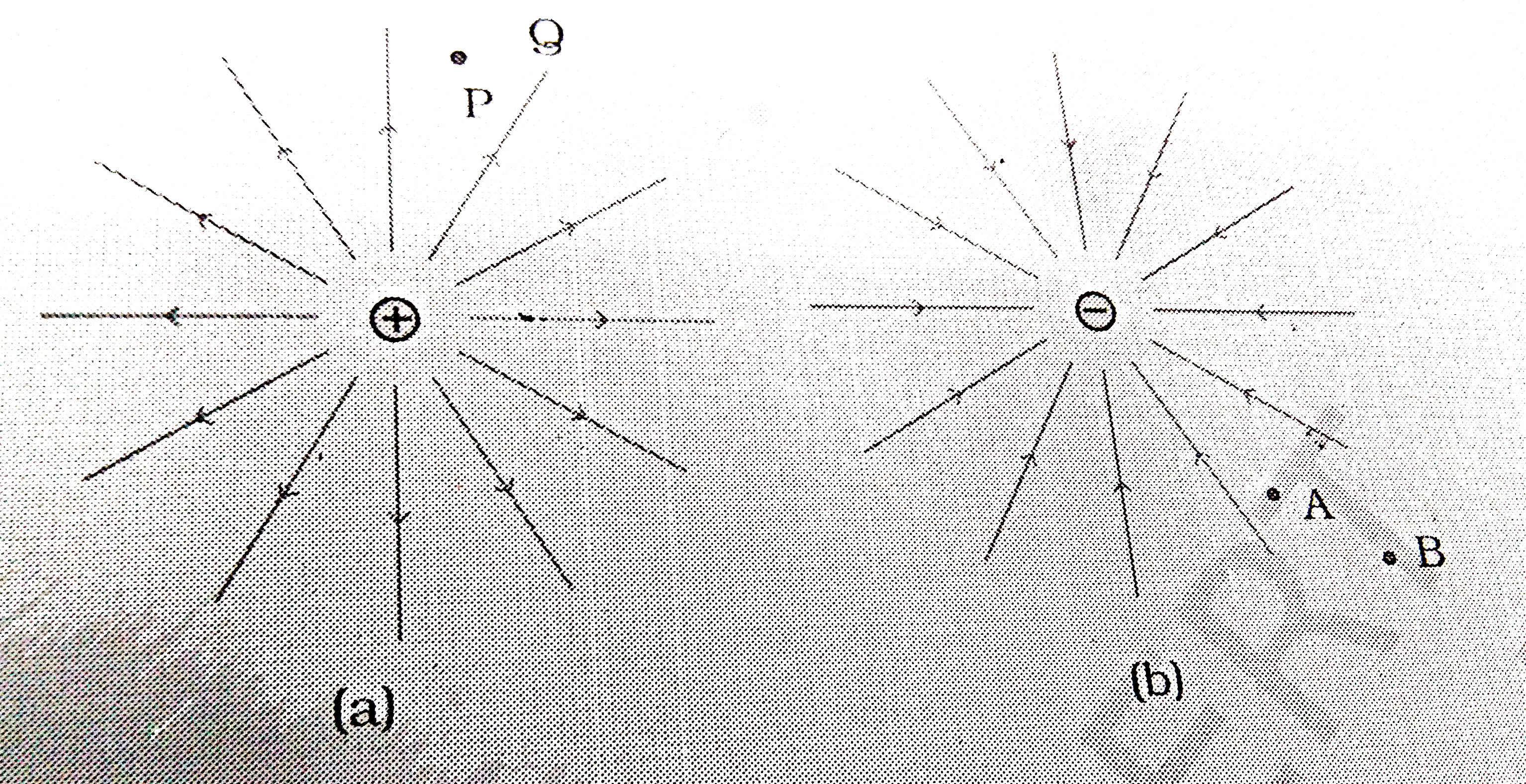
(a) Give the signs of the potential difference VP−VQ,VB−VA.
(b) Give the sign of the potential energy difference of a small negative charge between the points Q and P, A and B.
(c) Give the sign of the work done by the field in moving a small positive charge from Q to P.
(d) Give the sign of the work done by the external agency in moving a small negative charge from B to A.
(e) Does the kinetic energy of a small negative charge increase or decrease in going from B to A?
View SolutionTwo capacitors with capacities C1 and C2 ,are charged to potentials V1 and V2 respectively.When they are connected in parallel,the ratio of their respective charges is ....?
View SolutionDefine the emf (E) of a cell and the potential difference (V) across a resistor (R) in terms of the work done in moving a unit charge. State the relation between these two works and the work it charge through a cell connected across the resistor. Take the internal resistance of the cell as r. Hence, obtain expression for the current i in the circuit.
View SolutionTwo positive point charges of 12μC and 8μC are 10cm apart. The work done in bringing then 4cm closer is
View Solution
