A 12Ω resistance and an inductance of 0.05/π Hz with negligible resistance are connected in series. Across the ends of this circuit is connected a 130 V alternating voltage of frequency 50 Hz. Calculate the alternating current in the circuit and the potential difference across the resistance and that across the inductance.
The correct Answer is:10A,120V and 50 V
To solve the given problem step by step, we will follow these calculations:
Step 1: Calculate the Angular Frequency (ω)
The angular frequency (ω) is given by the formula:
ω=2πf
where f=50 Hz.
Calculating ω:
ω=2×π×50=100πrad/s
Step 2: Calculate the Inductive Reactance (XL)
The inductive reactance (XL) is given by the formula:
XL=ωL
where L=0.05π H.
Calculating XL:
XL=(100π)×(0.05π)=100×0.05=5Ω
Step 3: Calculate the Impedance (Z)
The impedance (Z) in an LR circuit is given by:
Z=√R2+X2L
where R=12Ω and XL=5Ω.
Calculating Z:
Z=√122+52=√144+25=√169=13Ω
Step 4: Calculate the Alternating Current (I)
The alternating current (I) can be calculated using Ohm's law for AC circuits:
I=VZ
where V=130V.
Calculating I:
I=13013=10A
Step 5: Calculate the Potential Difference Across the Resistance (VR)
The potential difference across the resistance (VR) is given by:
VR=I×R
Calculating VR:
VR=10×12=120V
Step 6: Calculate the Potential Difference Across the Inductance (VL)
The potential difference across the inductance (VL) is given by:
VL=I×XL
Calculating VL:
VL=10×5=50V
Summary of Results
- The alternating current in the circuit is 10A.
- The potential difference across the resistance is 120V.
- The potential difference across the inductance is 50V.
---
The angular frequency (ω) is given by the formula:
ω=2πf
where f=50 Hz.
ω=2×π×50=100πrad/s
The inductive reactance (XL) is given by the formula:
XL=ωL
where L=0.05π H.
XL=(100π)×(0.05π)=100×0.05=5Ω
The impedance (Z) in an LR circuit is given by:
Z=√R2+X2L
where R=12Ω and XL=5Ω.
Z=√122+52=√144+25=√169=13Ω
The alternating current (I) can be calculated using Ohm's law for AC circuits:
I=VZ
where V=130V.
I=13013=10A
The potential difference across the resistance (VR) is given by:
VR=I×R
VR=10×12=120V
The potential difference across the inductance (VL) is given by:
VL=I×XL
VL=10×5=50V
- The alternating current in the circuit is 10A.
- The potential difference across the resistance is 120V.
- The potential difference across the inductance is 50V.
Topper's Solved these Questions
- ALTERNATING CURRENTBook:AAKASH SERIESChapter:ALTERNATING CURRENTExercise:Additional Exercise
Explore 11 Videos
- ALTERNATING CURRENTBook:AAKASH SERIESChapter:ALTERNATING CURRENTExercise:PROBLEMS
Explore 28 Videos
- ALTERNATING CURRENTBook:AAKASH SERIESChapter:ALTERNATING CURRENTExercise:Problems (Level - I)
Explore 16 Videos
- APPENDICES ( REVISION EXERCISE )Book:AAKASH SERIESChapter:APPENDICES ( REVISION EXERCISE )Exercise:REVISION EXERCISE (MAGNETISM AND MATTER )
Explore 52 Videos
Similar Questions
An inductance and a resistance are connected in series with an AC potential . In this circuit
View SolutionA 0.21 H inductor and a 12Ω resistance are connected in series to a 220 V, 50 Hz ac source.
The current in the circuit is
View SolutionA coil of inductance 5.0 mH and negligible resistance is connected to an alternating voltage V=10sin(100t). The peak current in the circuit will be:
View SolutionA coil of resistance 300Ω and inductance 1.0 henry is connected across an voltages source of frequency 300/2πHz. The phase difference between the voltage and current in the circuit is
View SolutionTo a circuit of 1Ω resistance and 0.01 henry inductance is connected a 200 Volt line of frequency 50 cycles/ second. Calculate the reactance, the impedance and the current in the circuit and also the lag in phase between alternating voltage and current
View SolutionAn alternating voltage is connected in series with a resistance R and inductance L if the potential drop across the resistance is 200V and across the inductance is 150V, then the applied voltage is
View SolutionAn alternating voltage, of angular frequency ω is induced in electric circuit consistin of inductance L and capacitance C, connected in parallel. Then across the inductance coil
View SolutionAn electric lamp, whose resistance is 20Ω and a conductor of 4Ω resistance are connected to a 6V battery as shown in (Fig. 3.18) Calculate.
(a) the total resistance of the circuit,
(b) the current through the circuit, and
( c) the potential difference across the electric lamp and the conductor.
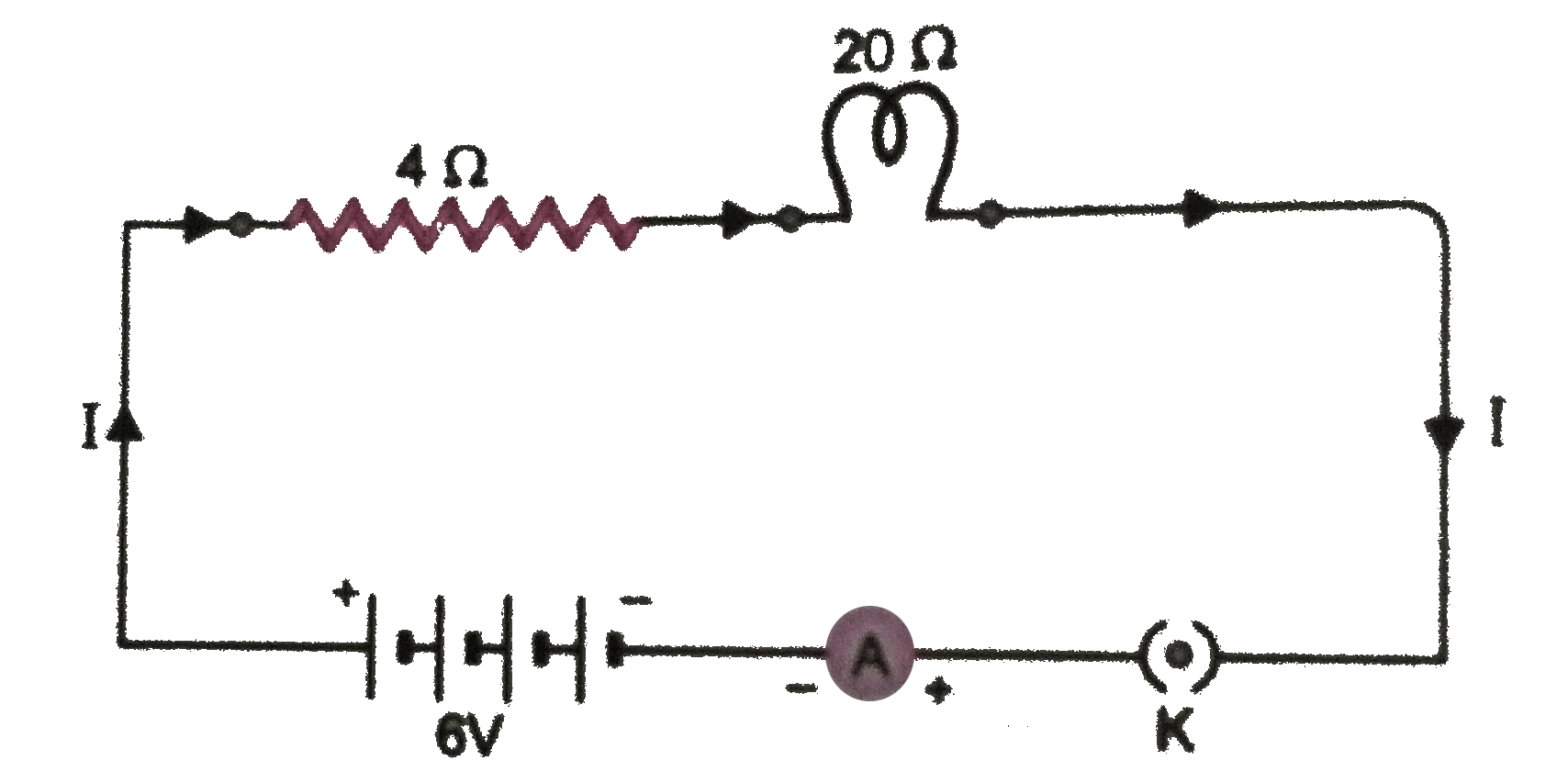 .
.
View SolutionA battery of emf 2 V and initial resistance 1Ω is
connected across terminals A and B of the circuit shown
in figure
 .
.
View SolutionAn inductive circuit contains a resistance of 10 ohms and an inductance of 2 henry. If an alternating voltage of 120 V and frequency 60 Hz is applied to this circuit, the current in the circuit would be nearly
View Solution