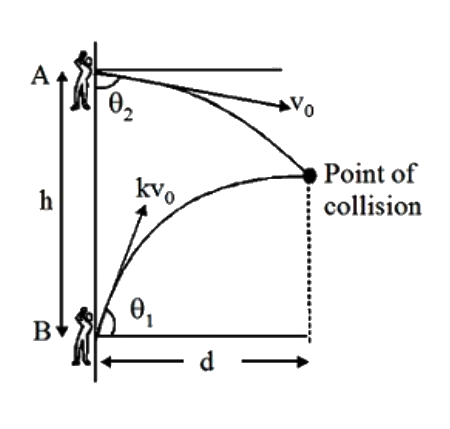
At the same instant two boy throw balls A and B from the window with a speed $v_{0}$ and $k v_{0}$ respectively , where k is a constant . Show that the balls with collide if $K = {cos θ}_{2} / {cos θ}_{1}$ .

Video Solution

Answer

Step by step video solution for At the same instant two boy throw balls A and B from the window with a speed v_(0) and kv_(0) respectively , where k is a constant . Show that the balls with collide if K = cos theta _(2)// cos theta_(1). by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.

Updated on:21/07/2023

Class 11 PHYSICS PROJECTILE MOTION

Similar Questions

At the same instant , two boys throw balls A and B from the positions shown with a speed $v_{0} and k v_{0}$ respectively , where k is a constant. For what value of k , balls will collide ? Relevant data is available in the figure .

View Solution
Show that: $t a n (60^{0} + θ) t a n (60^{0} - θ) = \frac{2 cos 2 θ + 1}{2 cos 2 θ - 1}$
View Solution
Eliminate $θ$ from the following equation : $x = h + a cos θ, y = k + b sin θ$
View Solution
A ball is thrown from a point with a speed 'v^(0)' at an elevation angle of $θ$ . From the same point and at the same instant , a person starts running with a constant speed $\frac{' v_{0}'}{2}$ to catch the ball . Will the person be able to catch the ball ? If yes, what should be the angle of projection $θ$ ?
View Solution
A ball is thrown from a point with a speed 'v^(0)' at an elevation angle of $θ$ . From the same point and at the same instant , a person starts running with a constant speed $\frac{' v_{0}'}{2}$ to catch the ball . Will the person be able to catch the ball ? If yes, what should be the angle of projection $θ$ ?
View Solution
A ball is thrown from a point with a speed 'v^(0)' at an elevation angle of $θ$ . From the same point and at the same instant , a person starts running with a constant speed $\frac{' v_{0}'}{2}$ to catch the ball . Will the person be able to catch the ball ? If yes, what should be the angle of projection $θ$ ?
View Solution
Solve $cos θ \cdot cos 2 θ \cdot cos 3 θ = \frac{1}{4}, 0 \leq θ \leq π$
View Solution
For all $θ$ in $[0, π / 2]$ , show that $cos (sin θ) > sin (cos θ)$ .
View Solution
Find the general value of $θ$ from the equation $cos θ + cos 2 θ + cos 3 θ = 0$ .
View Solution
The three sides of a triangle are $L_{r} + x {cos θ}_{r} + y {sin θ}_{r} - p_{r} = 0$ where r = 1,2,3 . Show that the orthocentre is given by
$L_{1} cos (θ_{2} - θ_{3}) = L_{2} cos (θ_{3} - θ_{1}) = L_{3} cos (θ_{1} - θ_{2})$ .
View Solution

At the same instant two boy throw balls A and B from the window with a speed v0 and kv0 respectively , where k is a constant . Show that the balls with collide if K=cosθ2/cosθ1.

Similar Questions

Recommended Questions

At the same instant two boy throw balls A and B from the window with a speed $v_{0}$ and $k v_{0}$ respectively , where k is a constant . Show that the balls with collide if $K = {cos θ}_{2} / {cos θ}_{1}$ .