The PE of a satellite is -U . Its KE is
U
2U
−U
U/2
The correct Answer is:C
To find the kinetic energy (KE) of a satellite given its potential energy (PE) as -U, we can follow these steps:
1. Understanding Potential Energy:
The potential energy (PE) of a satellite in orbit around a planet is given by the formula:
PE=−GMmr
where G is the gravitational constant, M is the mass of the planet, m is the mass of the satellite, and r is the distance from the center of the planet to the satellite.
2. Given Information:
According to the problem, the potential energy of the satellite is given as:
PE=−U
This implies:
−GMmr=−U⇒GMmr=U
3. Finding Kinetic Energy:
The kinetic energy (KE) of the satellite can be expressed in terms of its orbital velocity (v0):
KE=12mv20
The orbital velocity v0 can be derived from the gravitational force acting on the satellite, which provides the necessary centripetal force for circular motion:
GMmr2=mv20r
From this equation, we can solve for v20:
v20=GMr
4. Substituting into Kinetic Energy:
Now, substituting v20 into the kinetic energy formula:
KE=12m(GMr)
5. Relating Kinetic Energy to Potential Energy:
We already established that:
U=GMmr
Therefore, we can express the kinetic energy in terms of U:
KE=12U
6. Final Answer:
Thus, the kinetic energy of the satellite is:
KE=U2
Conclusion:
The kinetic energy of the satellite is U2.
The potential energy (PE) of a satellite in orbit around a planet is given by the formula:
PE=−GMmr
where G is the gravitational constant, M is the mass of the planet, m is the mass of the satellite, and r is the distance from the center of the planet to the satellite.
According to the problem, the potential energy of the satellite is given as:
PE=−U
This implies:
−GMmr=−U⇒GMmr=U
The kinetic energy (KE) of the satellite can be expressed in terms of its orbital velocity (v0):
KE=12mv20
The orbital velocity v0 can be derived from the gravitational force acting on the satellite, which provides the necessary centripetal force for circular motion:
GMmr2=mv20r
From this equation, we can solve for v20:
v20=GMr
Now, substituting v20 into the kinetic energy formula:
KE=12m(GMr)
We already established that:
U=GMmr
Therefore, we can express the kinetic energy in terms of U:
KE=12U
Thus, the kinetic energy of the satellite is:
KE=U2
The kinetic energy of the satellite is U2.
Similar Questions
In an orbit if the time of revolution of satellite is T , then PE is proportional to
View SolutionIn the graph shown, the PE of earth-satellite system is shown by a solid line as a function of distance r (the separation between earth's centre and satellite). The total energy of the two objects which may or may not be bounded to the earth are shown in the figure by dotted lines.
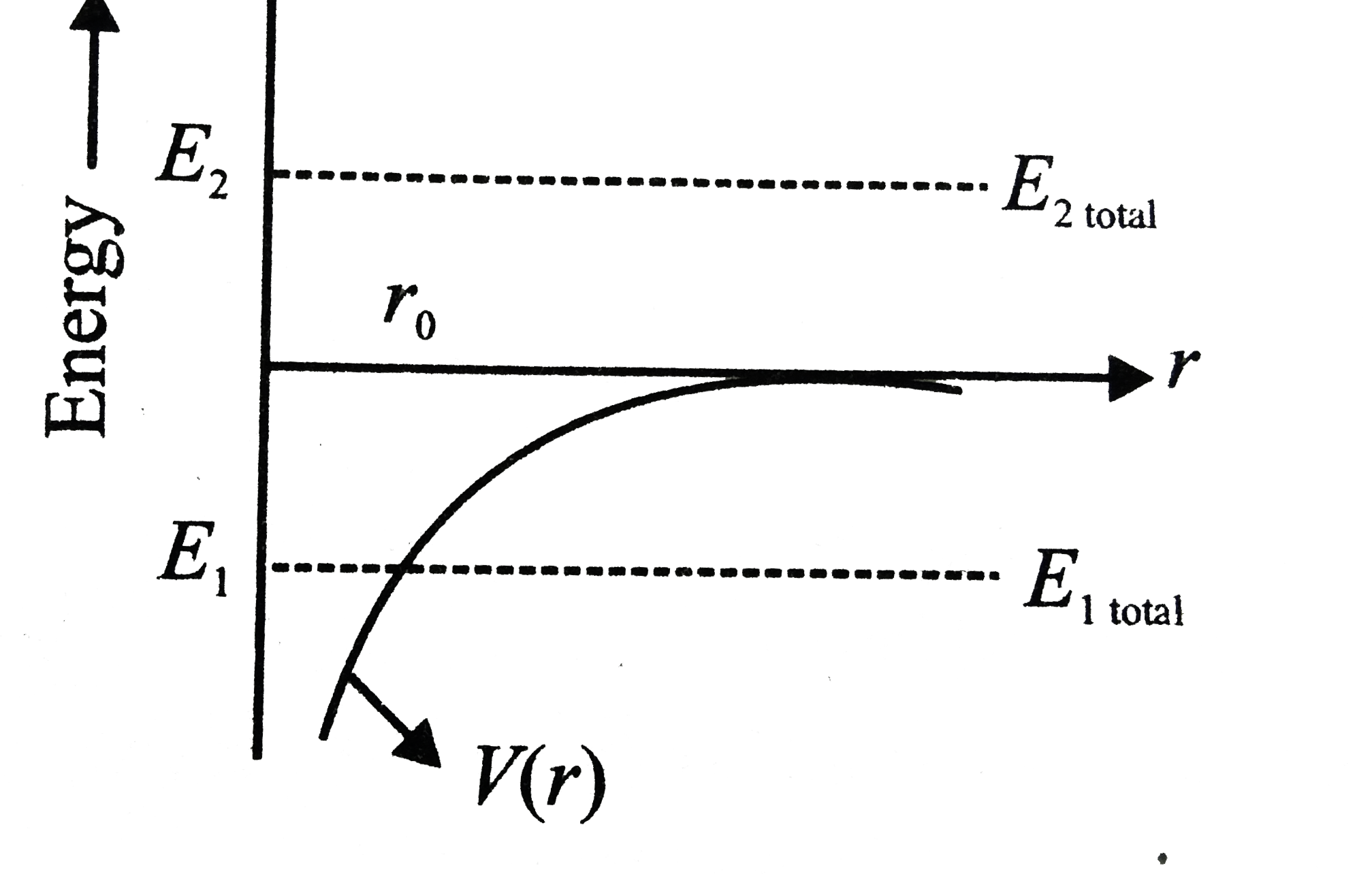
Based on the above information, answer the following questions.
Mark the correct statement(s).
View SolutionIn the graph shown, the PE of earth-satellite system is shown by a solid line as a function of distance r (the separation between earth's centre and satellite). The total energy of the two objects which may or may not be bounded to the earth are shown in the figure by dotted lines.
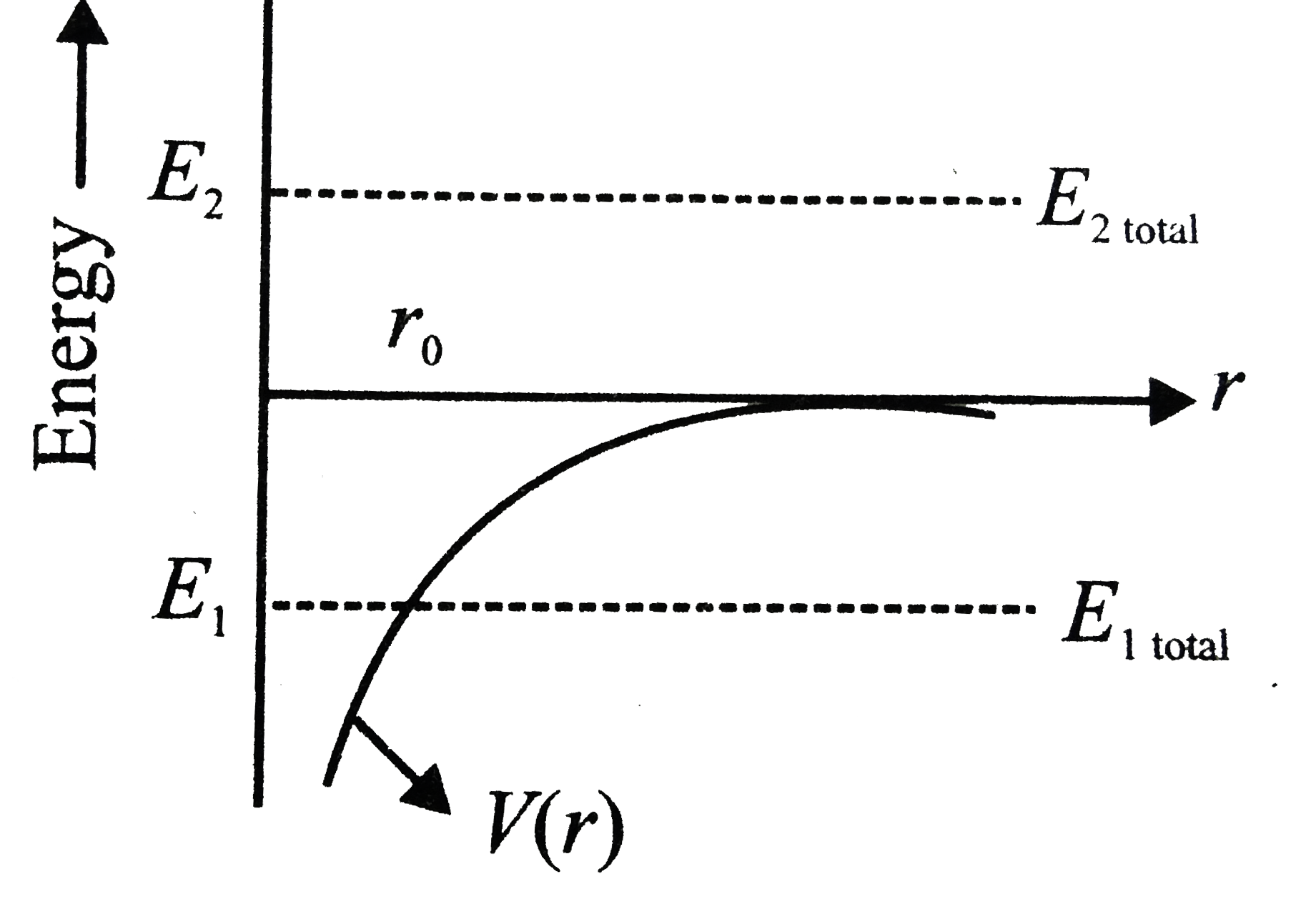
Based on the above information, answer the following questions.
If object having total energy E1 is having the same PE curve as shown in the figure, then
View SolutionIn the graph shown, the PE of earth-satellite system is shown by a solid line as a function of distance r (the separation between earth's centre and satellite). The total energy of the two objects which may or may not be bounded to the earth are shown in the figure by dotted lines.

Based on the above information, answer the following questions. If both the objects have the same PE curve as shown in the figure, then
View SolutionThe correct graph representing the variation of total energy (Et), kinetic energy (Ek) and potential energy (U) of a satellite with its distance form the centre of earth is
View SolutionRadius of orbit of satellite of earth is R. Its kinetic energy is proportional to
View SolutionCan we find the mass of a satellite by finding its Time period?
View SolutionAssertion : If radius of earth suddenly shrinks to half its present without changing its mass value, then the period of an earth's satellite will not change
Reason : Time period of a satellite does not upon the mass of earth.
View SolutionA satellite moves around the earth in a circular orbit with speed v. If m is the mass of the satellite, its total energy is
View SolutionIf the P.E. of an electron is −68eV in hydrogen atom then find out K.E.,E of orbit where electron exist & radius of orbit.
View Solution
