A body is moving under the expense of a constant power. If .X. is the displacement in time. t1, then .x. is proportional to
t2/3
t3/2
t2
t1/2
The correct Answer is:B
To solve the problem, we need to analyze the relationship between displacement x and time t when a body is moving under the influence of a constant power.
1. Understanding Power:
Power P is defined as the rate at which work is done. Mathematically, it can be expressed as:
P=F⋅v
where F is the force and v is the velocity of the body.
2. Expressing Power in Terms of Velocity:
Since the power is constant, we can denote it as k:
F⋅v=k
3. Relating Force to Mass and Acceleration:
According to Newton's second law, force can also be expressed as:
F=m⋅a
where m is the mass and a is the acceleration. Since acceleration a can be expressed as the derivative of velocity with respect to time:
a=dvdt
4. Substituting for Force:
Substituting the expression for force into the power equation gives:
m⋅a⋅v=k
or
m⋅v⋅dvdt=k
5. Rearranging the Equation:
Rearranging this equation leads to:
m⋅v⋅dv=k⋅dt
6. Integrating Both Sides:
We can integrate both sides. The left side integrates with respect to v and the right side with respect to t:
∫m⋅vdv=∫kdt
This results in:
m2v2=k⋅t+C
where C is the constant of integration.
7. Finding Velocity:
Solving for v:
v2=2kmt+2Cm
For simplicity, we can assume C=0 (starting from rest), leading to:
v2=2kmt
Thus,
v=√2kmt
8. Relating Displacement to Velocity:
Since velocity v is also defined as the derivative of displacement x with respect to time t:
v=dxdt
We can substitute for v:
dxdt=√2kmt
9. Integrating to Find Displacement:
Now we integrate to find x:
dx=√2km√tdt
Integrating both sides gives:
x=√2km⋅23t3/2+C
Again, assuming C=0, we have:
x=23√2kmt3/2
10. Final Relationship:
Thus, we conclude that:
x∝t3/2
Conclusion:
The displacement x is proportional to t3/2.
Power P is defined as the rate at which work is done. Mathematically, it can be expressed as:
P=F⋅v
where F is the force and v is the velocity of the body.
Since the power is constant, we can denote it as k:
F⋅v=k
According to Newton's second law, force can also be expressed as:
F=m⋅a
where m is the mass and a is the acceleration. Since acceleration a can be expressed as the derivative of velocity with respect to time:
a=dvdt
Substituting the expression for force into the power equation gives:
m⋅a⋅v=k
or
m⋅v⋅dvdt=k
Rearranging this equation leads to:
m⋅v⋅dv=k⋅dt
We can integrate both sides. The left side integrates with respect to v and the right side with respect to t:
∫m⋅vdv=∫kdt
This results in:
m2v2=k⋅t+C
where C is the constant of integration.
Solving for v:
v2=2kmt+2Cm
For simplicity, we can assume C=0 (starting from rest), leading to:
v2=2kmt
Thus,
v=√2kmt
Since velocity v is also defined as the derivative of displacement x with respect to time t:
v=dxdt
We can substitute for v:
dxdt=√2kmt
Now we integrate to find x:
dx=√2km√tdt
Integrating both sides gives:
x=√2km⋅23t3/2+C
Again, assuming C=0, we have:
x=23√2kmt3/2
Thus, we conclude that:
x∝t3/2
The displacement x is proportional to t3/2.
Similar Questions
A body is moving undirectionally under the influence of a source of constatn power. It displacement in time t is proportional to (i) t1/2 (ii) t (iii) t3/2 (iv) t2
View SolutionA body of mas m starts from rest with a constant power. If velocity of the body at displacement s is v, then the correct alternative is
View Solution
Knowledge Check
A body is moving unidirectionally under the influence of a source of constant power supplying energy. Which of the diagrams shown in figure. Correctly shows the displacement-time curve for its motion ?
A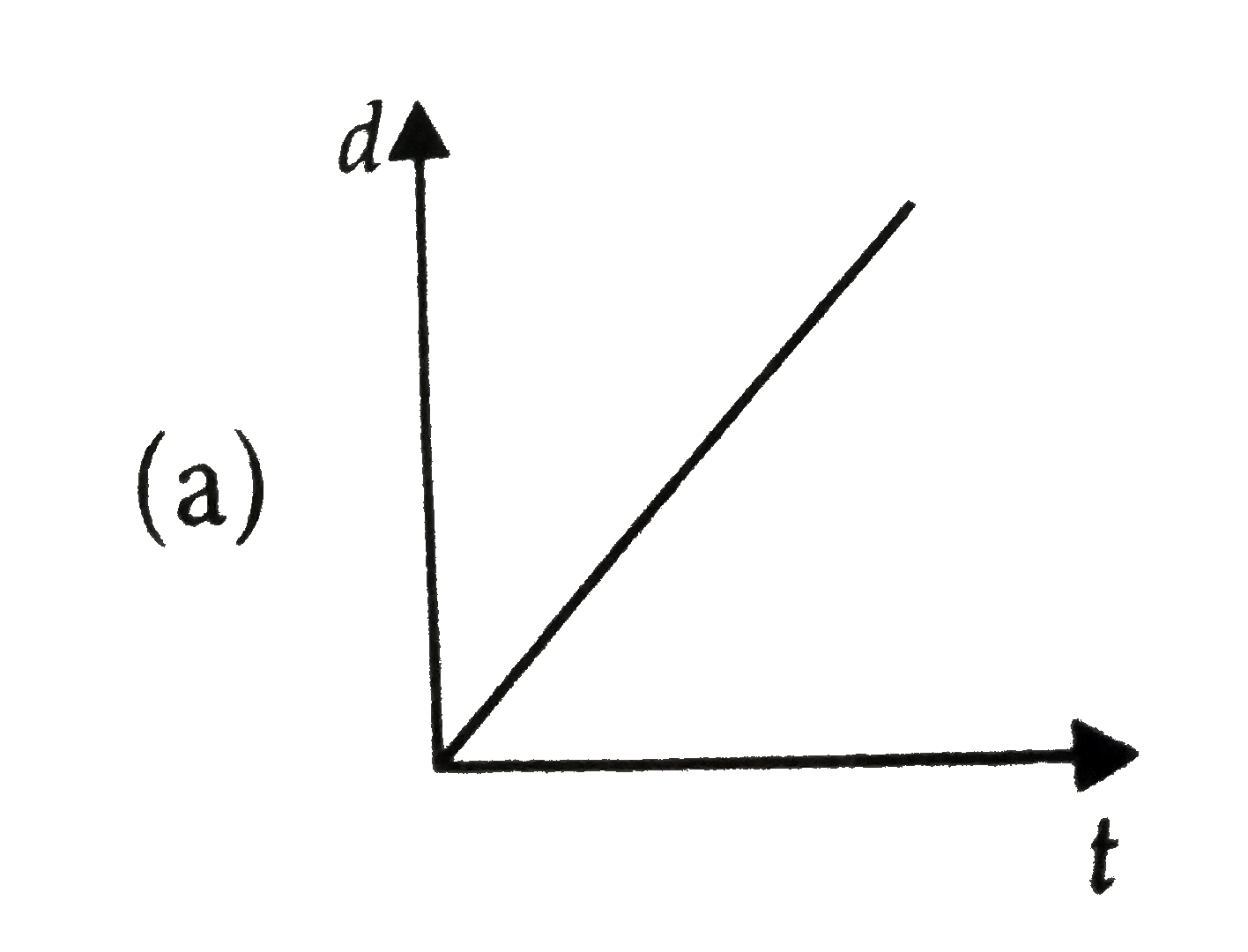 B
B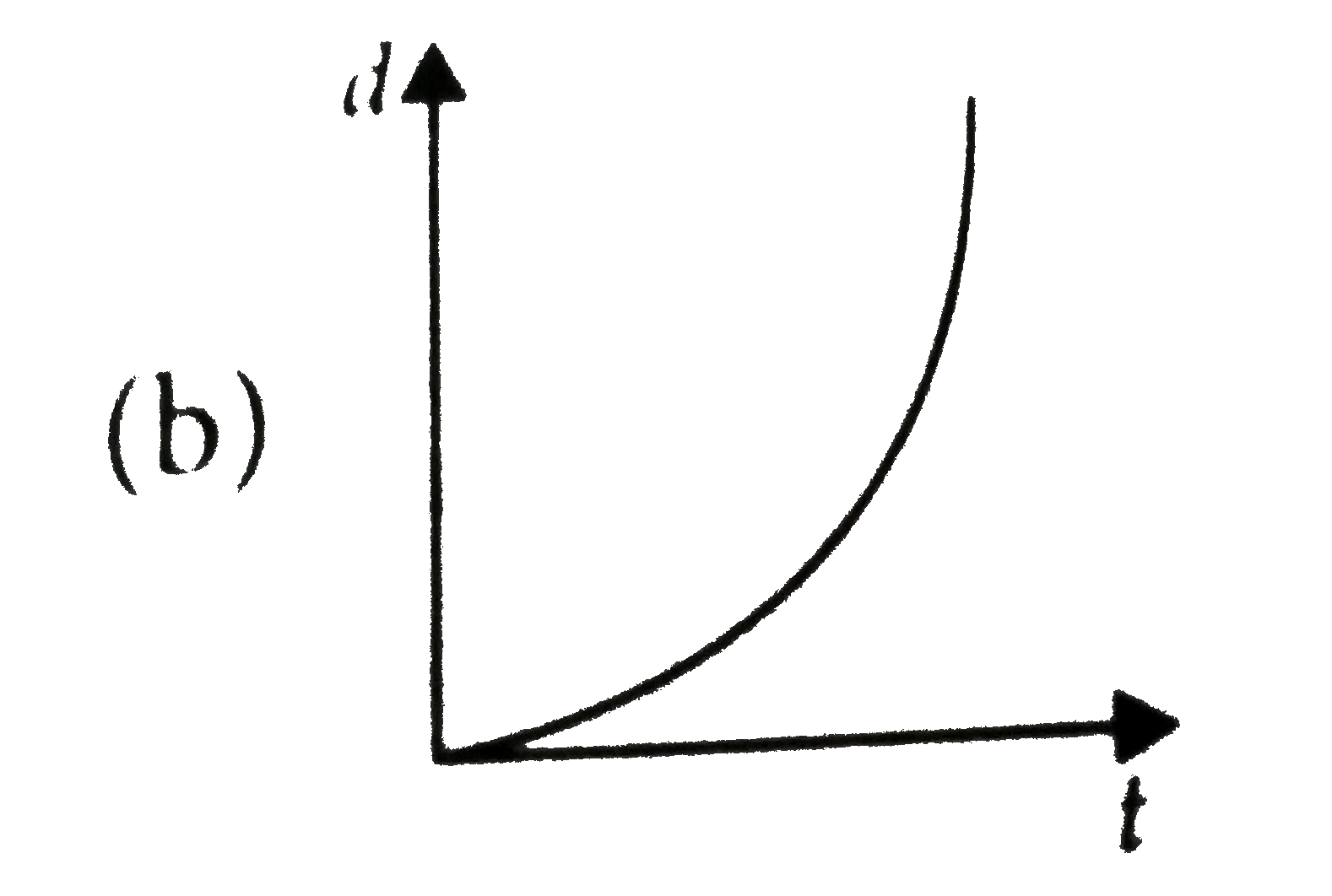 C
C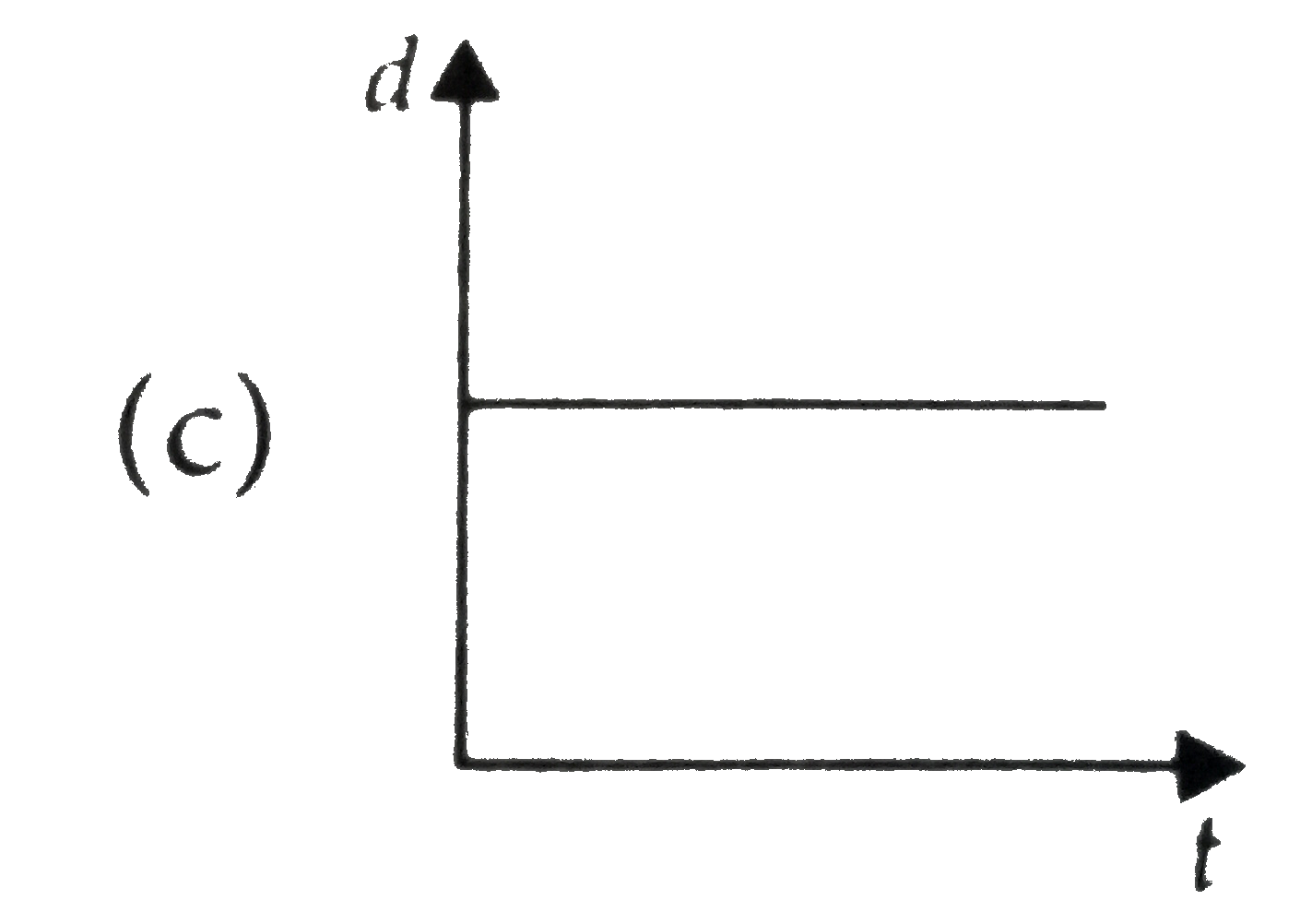 D
D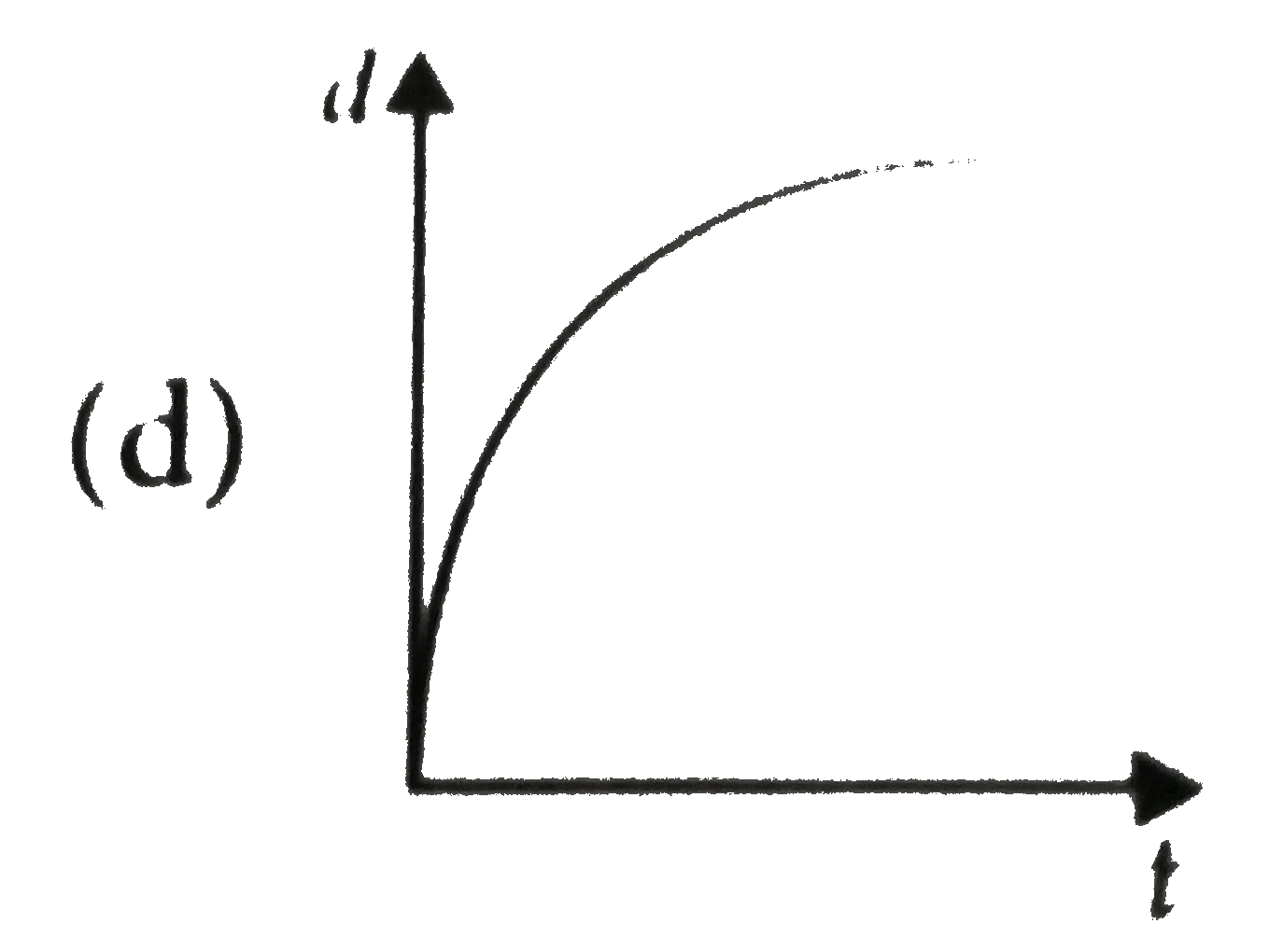
If x denotes displacement in time t and x=a cost, then acceleration is :-
View SolutionA body is moved along a straight line by a machine delivering constant power . The distance moved by the body is time t is proptional to
View SolutionA body is moved along a straight line by a machine delivering constant power . The distance moved by the body is time t is proptional to
View SolutionA body is moved along a straight line by a machine delivering constant power . The distance moved by the body is time t is proptional to
View SolutionA body is moving from rest under constant acceleration and let S1 be the displacement in the first (p−1) sec and S2 be the displacement in the first p sec. The displacement in (p2−p+1) sec. will be
View SolutionA body is moving from rest under constance accelration and let S1 be the displacement in the first (p−1) sec and S2 be the displacement in the first p sec. The displacement in (p2−p+1)th sec will be
View SolutionA body is moving unidirectionally under the influence of a source of constant power supplying energy. Which of the diagrams shown in figure. Correctly shows the displacement-time curve for its motion ?
View Solution
