The work done by a force →F=(−6x2ˆi)N in displacing a particle from x = 4mtox = -2mis
−240J
360J
420J
144J
The correct Answer is:D
To find the work done by the force →F=−6x2^i in displacing a particle from x=4m to x=−2m, we can follow these steps:
Step 1: Understand the Work Done Formula
The work done W by a force →F when a particle moves through a displacement d→x is given by:
dW=→F⋅d→x
In this case, since the force is in the x direction, we can express it as:
dW=Fdx
Step 2: Substitute the Force
Given the force →F=−6x2^i, we can write:
dW=−6x2dx
Step 3: Set Up the Integral for Work Done
To find the total work done as the particle moves from x=4m to x=−2m, we need to integrate:
W=∫−24−6x2dx
Step 4: Perform the Integration
We can factor out the constant:
W=−6∫−24x2dx
Now, we calculate the integral:
∫x2dx=x33
Thus,
W=−6(x33)−24
Step 5: Evaluate the Integral at the Limits
Now we substitute the limits into the integrated function:
W=−6((−2)33−(4)33)
Calculating the cubes:
(−2)3=−8and(4)3=64
So we have:
W=−6(−83−643)
This simplifies to:
W=−6(−8−643)=−6(−723)
Step 6: Simplify the Expression
Calculating the fraction:
W=−6(−24)=144J
Final Answer
Thus, the work done by the force in displacing the particle from x=4m to x=−2m is:
W=144J
The work done W by a force →F when a particle moves through a displacement d→x is given by:
dW=→F⋅d→x
In this case, since the force is in the x direction, we can express it as:
dW=Fdx
Given the force →F=−6x2^i, we can write:
dW=−6x2dx
To find the total work done as the particle moves from x=4m to x=−2m, we need to integrate:
W=∫−24−6x2dx
We can factor out the constant:
W=−6∫−24x2dx
Now, we calculate the integral:
∫x2dx=x33
Thus,
W=−6(x33)−24
Now we substitute the limits into the integrated function:
W=−6((−2)33−(4)33)
Calculating the cubes:
(−2)3=−8and(4)3=64
So we have:
W=−6(−83−643)
This simplifies to:
W=−6(−8−643)=−6(−723)
Calculating the fraction:
W=−6(−24)=144J
Thus, the work done by the force in displacing the particle from x=4m to x=−2m is:
W=144J
Similar Questions
The work done by a force →F=(−6x3ˆi) N in displacing a particle from x = 4m to x = - 2m is
View SolutionA force F=−kx2(x≠0) acts on a particle in x-direction. Find the work done by this force in displacing the particle from. x=+a to x=+2a. Where, k is a positive constant.
View SolutionA force F=−kx2(x≠0) acts on a particle in x-direction. Find the work done by this force in displacing the particle from. x=+a to x=2a. Here, k is a positive constant.
View SolutionThe work done by the forces →F=2ˆi−3ˆj+2ˆk in moving a particle from (3,4,5) to (1,2,3) is (A) 0 (B) 32 (C) −4 (D) −2
View SolutionA force (F) acting on a particle varies work done by a particle varies with the with the position x as shown in figure
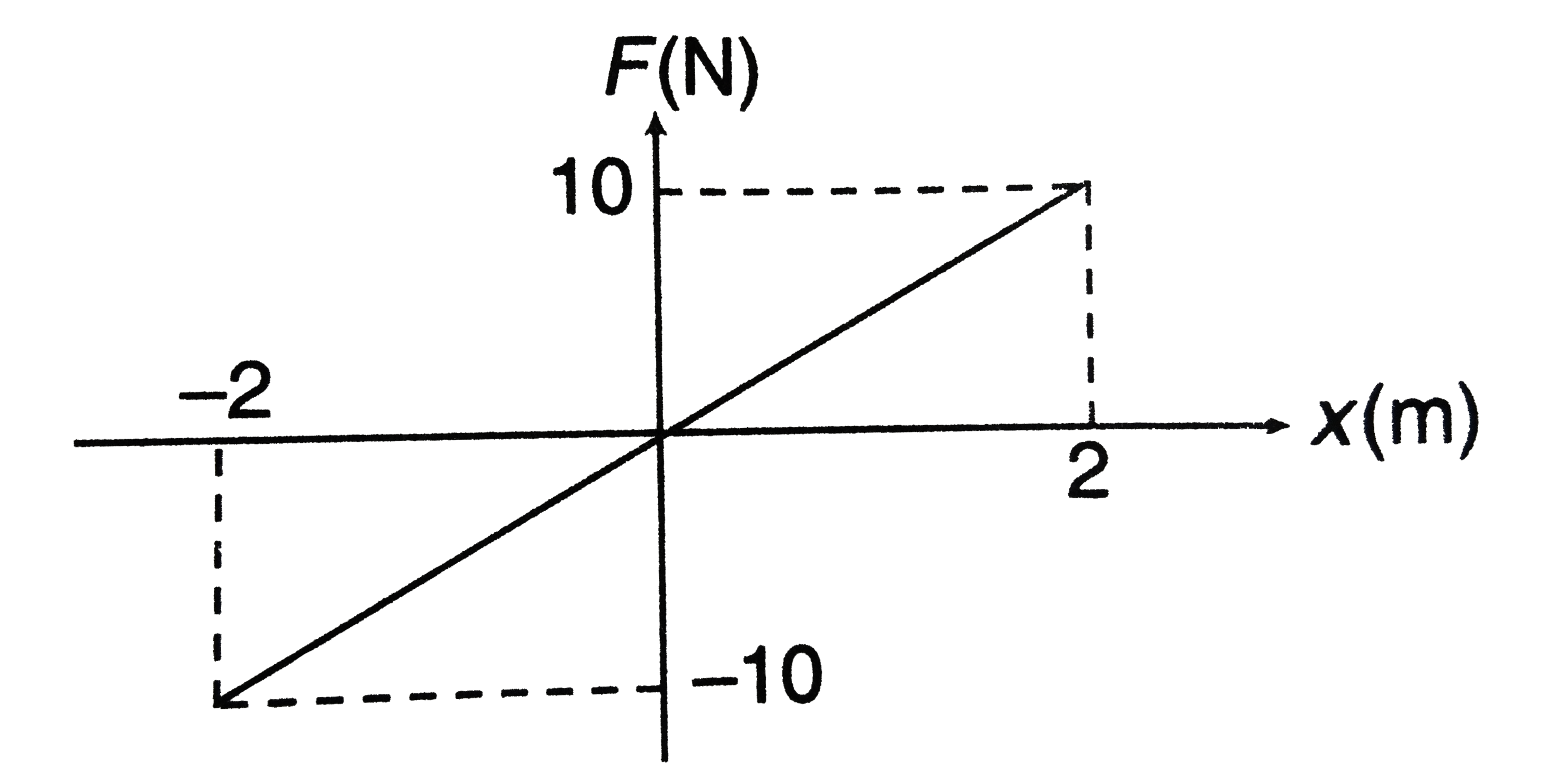 .
.
Find the work done by by force in displacing the particle from .
(a) x=−2m to x=0
(b) x=0 to x=2m..
View SolutionA particle of mass 2kg travels along a straight line with velocity v=a√x, where a is a constant. The work done by net force during the displacement of particle from x=0 to x=4m is
View SolutionThe work done by the forces →F=2ˆi−ˆj−ˆk in moving an object along the vectors 3ˆi+2ˆj−5ˆk is:
View SolutionWhen a force →F=(6ˆi−2ˆj) N displaces a particle by →S=(2ˆi−3ˆj) m, then average power is 0.4w. The time of action of force is
View SolutionWork done by a force F=(ˆi+2ˆj+3ˆk) acting on a particle in displacing it from the point r1=ˆi+ˆj+ˆk to the point r2=ˆi−ˆj+ˆ2k is
View SolutionWork done when a force F=(ˆi+2ˆj+3ˆk)N acting on a particle takes it from the point
r1=(ˆi+ˆk) the point r2=(ˆi−ˆj+2ˆk) is .
View Solution