When a number of liquid drops each of surface charge density σ and energy E combine, a large drop is formed. If the charge density of the large drop is 3 σ , its energy is
81E
3E
27E
243 E
The correct Answer is:D
To solve the problem step by step, we will analyze the situation of small liquid drops merging to form a larger drop, considering the conservation of charge and volume.
Step 1: Define the parameters for small drops
Let:
- σ = surface charge density of small drops
- E = energy of one small drop
- r = radius of one small drop
- A = surface area of one small drop = 4πr2
- q = charge on one small drop = σ⋅A=σ⋅4πr2
Step 2: Define the parameters for the large drop
Let:
- R = radius of the large drop
- The surface charge density of the large drop is given as 3σ.
- The surface area of the large drop = 4πR2
- The charge on the large drop = 3σ⋅4πR2
Step 3: Conservation of charge
The total charge before merging (from n small drops) must equal the charge of the large drop:
n⋅q=3σ⋅4πR2
Substituting q:
n⋅(σ⋅4πr2)=3σ⋅4πR2
Canceling 4πσ from both sides:
nr2=3R2(1)
Step 4: Conservation of volume
The volume of n small drops must equal the volume of the large drop:
n⋅(43πr3)=43πR3
Canceling 43π:
nr3=R3(2)
Step 5: Relate R and r using equations (1) and (2)
From equation (1):
R2=nr23
Substituting this into equation (2):
nr3=(nr23)3/2
Simplifying:
nr3=n3/2r333/2
Canceling r3 (assuming r≠0):
n=n3/233/2
Rearranging gives:
n1/2=33/2⇒n=27
Step 6: Calculate the energy of the large drop
The electrostatic potential energy U of a drop is given by:
U=14πϵ0q2R
For one small drop:
E=14πϵ0q2r
For the large drop:
Elarge=14πϵ0(3q)2R
Substituting q=σ⋅4πr2:
Elarge=14πϵ0(3⋅σ⋅4πr2)2R
Now, substituting R using n=27:
Elarge=14πϵ0(3q)2nr23=14πϵ09q227r23=14πϵ027q2r2
Thus:
Elarge=27⋅E
Final Step: Conclusion
The energy of the large drop is:
Elarge=243E
Let:
- σ = surface charge density of small drops
- E = energy of one small drop
- r = radius of one small drop
- A = surface area of one small drop = 4πr2
- q = charge on one small drop = σ⋅A=σ⋅4πr2
Let:
- R = radius of the large drop
- The surface charge density of the large drop is given as 3σ.
- The surface area of the large drop = 4πR2
- The charge on the large drop = 3σ⋅4πR2
The total charge before merging (from n small drops) must equal the charge of the large drop:
n⋅q=3σ⋅4πR2
Substituting q:
n⋅(σ⋅4πr2)=3σ⋅4πR2
Canceling 4πσ from both sides:
nr2=3R2(1)
The volume of n small drops must equal the volume of the large drop:
n⋅(43πr3)=43πR3
Canceling 43π:
nr3=R3(2)
From equation (1):
R2=nr23
Substituting this into equation (2):
nr3=(nr23)3/2
Simplifying:
nr3=n3/2r333/2
Canceling r3 (assuming r≠0):
n=n3/233/2
Rearranging gives:
n1/2=33/2⇒n=27
The electrostatic potential energy U of a drop is given by:
U=14πϵ0q2R
For one small drop:
E=14πϵ0q2r
For the large drop:
Elarge=14πϵ0(3q)2R
Substituting q=σ⋅4πr2:
Elarge=14πϵ0(3⋅σ⋅4πr2)2R
Now, substituting R using n=27:
Elarge=14πϵ0(3q)2nr23=14πϵ09q227r23=14πϵ027q2r2
Thus:
Elarge=27⋅E
The energy of the large drop is:
Elarge=243E
Topper's Solved these Questions
- CAPACITORS BOOK - AAKASH SERIESCHAPTER - CAPACITORS EXERCISE - LECTURE SHEET (EXERCISE - II) (LEVEL - I (ADVANCED)) (More than one correct answer Type Questions )
1 Videos
- CAPACITORS BOOK - AAKASH SERIESCHAPTER - CAPACITORS EXERCISE - LECTURE SHEET (EXERCISE - II) (LEVEL - I (ADVANCED)) (Linked ComprehensionType Questions )
7 Videos
- CAPACITORS BOOK - AAKASH SERIESCHAPTER - CAPACITORS EXERCISE - LECTURE SHEET (EXERCISE - II) (LEVEL - I (MAIN)) (Straight Objective Type Questions )
6 Videos
- ATOMSBOOK - AAKASH SERIESCHAPTER - ATOMSEXERCISE - PRACTICE EXERCISE
21 Videos
- COMMUNICATION SYSTEM BOOK - AAKASH SERIESCHAPTER - COMMUNICATION SYSTEM EXERCISE - LECTURE SHEET (LEVEL - I) (MAIN) (EXERCISE - IV) (Straight Objective & More than one Correct Answer Type Questions)
20 Videos
Similar Questions
A parallel plate capacitors of plate area A has a total surface charge density +σ on one plate and a total surface charge density −σ on the other plate. The force one plate due to the other plate is given by :
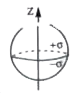
View SolutionA hollow insulating spherical shell has a surfac charge distribution placed upon it, such that the upper hemisphere has a uniform surface charge density σ. While the lower hemisphere has a uniform surface charge density −σ.as shown in the figure. Their interface lies in x-y plane which
View Solution
Knowledge Check
When a charge of amount Q is given to an isolated metal plate X of surface area A, its surface charge density becomes σ1. When an isolated identical plate Y is brought close to X the surface charge density on X becomes σ2. When Y is earthed the surface charge density on X becomes σ3. Choose the incorrect option.
Aσ1=QABσ1=Q2ACσ1=σ2Dσ3=QA
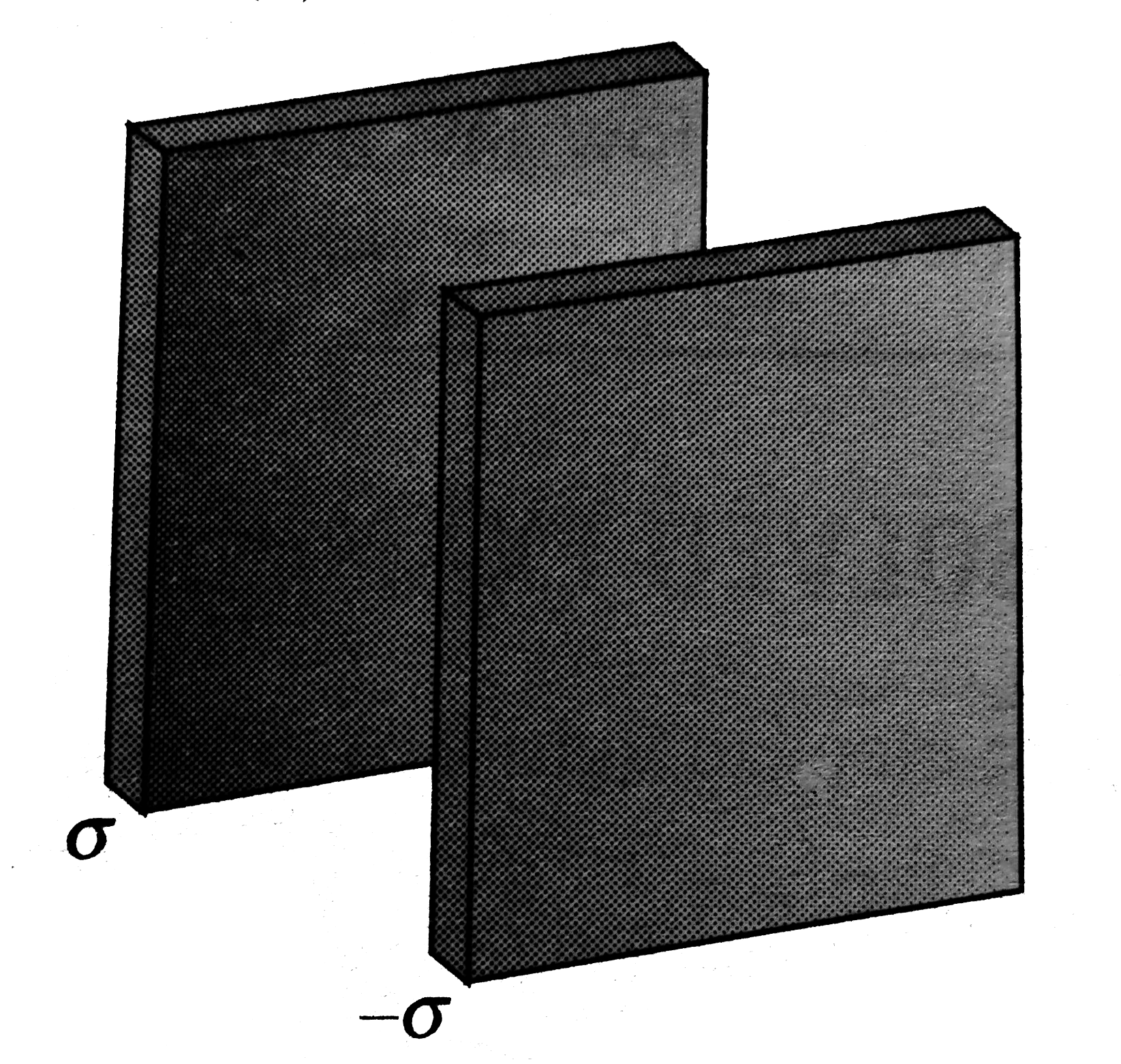
Two infinite, non-conducting sheets of charge are parallel to each other, as shown in figure. The sheet on the left has a uniform surface charge density σ, and the one on the right has a uniform charge density −σ. Calculate the electric field at points (a) to the left of, (b) in between, and (c) to the right of the two sheets.
View SolutionA large number of liquid drops each of radius 'a' coalesce to form a single spherical drop of radius b. The energy released in the process is converted into kinetic energy of the big drops formed. The speed of big drop will be
View SolutionA liquid of diameter D breaks up into 64 tiny drops. If the surface tension of the liquid is σ what is the change in energy?
View SolutionEight small drops, each of radius r and having same charge q are combined to form a big drop. The ratio between the potentials of the bigger drop and the smaller drop is
View Solution(a) Use Gauss's theorem to find the electric field due to a uniformly charged infinitely
Iarge plane thin sheet with surface charge density.Σ
(b) An infinitely large thin plane sheet has a uniform surface charge density +σ. Obtain the
expression for the amount of work done in bringing a point charge q from infinity to a
point , distant r, in front of the charged plane sheet.
View SolutionIf n drops, each of capacitance C and charged to a potential V, coalesce to form a big drop, the ratio of the energy stored in the big drop to that in each small drop will be
View SolutionSixty four spherical drops each of radius 2cm and carrying 5C charge combine to form a bigger drop. Its capacity is.
View Solution
