Arrange the ascending order of polarising angles for air-glass, air-water, and water-glass interfaces?
water-glass, air-water, air-glass
air-water, air-glass, water-glass
air-glass, air-water, water-glass
air-water, water-glass, air-glass
The correct Answer is:A
To find the ascending order of polarizing angles for the air-glass, air-water, and water-glass interfaces, we will use the formula for the polarizing angle (also known as Brewster's angle), which is given by:
tan(θp)=n2n1
where n1 is the refractive index of the first medium and n2 is the refractive index of the second medium.
Step 1: Identify the refractive indices
- Refractive index of air (nair) = 1.00
- Refractive index of water (nwater) = 1.33
- Refractive index of glass (nglass) = 1.50
Step 2: Calculate the polarizing angle for air-glass interface
Using the formula:
tan(θp1)=nglassnair=1.501.00=1.50
Now, we find θp1:
θp1=tan−1(1.50)≈56.31∘
Step 3: Calculate the polarizing angle for air-water interface
Using the formula:
tan(θp2)=nwaternair=1.331.00=1.33
Now, we find θp2:
θp2=tan−1(1.33)≈53.06∘
Step 4: Calculate the polarizing angle for water-glass interface
Using the formula:
tan(θp3)=nglassnwater=1.501.33≈1.13
Now, we find θp3:
θp3=tan−1(1.13)≈48.37∘
Step 5: Arrange the angles in ascending order
Now we have the polarizing angles:
- θp1 (air-glass) ≈ 56.31°
- θp2 (air-water) ≈ 53.06°
- θp3 (water-glass) ≈ 48.37°
Arranging these in ascending order:
1. Water-glass: θp3≈48.37∘
2. Air-water: θp2≈53.06∘
3. Air-glass: θp1≈56.31∘
Final Answer
The ascending order of polarizing angles is:
1. Water-glass
2. Air-water
3. Air-glass
- Refractive index of air (nair) = 1.00
- Refractive index of water (nwater) = 1.33
- Refractive index of glass (nglass) = 1.50
Using the formula:
tan(θp1)=nglassnair=1.501.00=1.50
Now, we find θp1:
θp1=tan−1(1.50)≈56.31∘
Using the formula:
tan(θp2)=nwaternair=1.331.00=1.33
Now, we find θp2:
θp2=tan−1(1.33)≈53.06∘
Using the formula:
tan(θp3)=nglassnwater=1.501.33≈1.13
Now, we find θp3:
θp3=tan−1(1.13)≈48.37∘
Now we have the polarizing angles:
- θp1 (air-glass) ≈ 56.31°
- θp2 (air-water) ≈ 53.06°
- θp3 (water-glass) ≈ 48.37°
1. Water-glass: θp3≈48.37∘
2. Air-water: θp2≈53.06∘
3. Air-glass: θp1≈56.31∘
The ascending order of polarizing angles is:
1. Water-glass
2. Air-water
3. Air-glass
Topper's Solved these Questions
- WAVES OPTICSBook:AAKASH SERIESChapter:WAVES OPTICSExercise:EXERCISE -IB (ASSERTION AND REASON)
Explore 67 Videos
- WAVES OPTICSBook:AAKASH SERIESChapter:WAVES OPTICSExercise:EXERCISE -II (INTERFERENCE)
Explore 26 Videos
- WAVES OPTICSBook:AAKASH SERIESChapter:WAVES OPTICSExercise:EXERCISE -IA (MATCHING TYPE QUESTIONS)
Explore 6 Videos
- WAVESBook:AAKASH SERIESChapter:WAVESExercise:EXERCISE-III (Doppler effect :)
Explore 15 Videos
Similar Questions
Knowledge Check
The critical angle for glass-air interface is :
A24∘B48∘C42∘D45∘
State the approximate value of the critical angle for (a) glass-air surface
(b) water-air surface.
View SolutionArrange air, glass, and water in the ascending order of optical density.
View SolutionFig. (a) and (b) show refraction of an incident ray in air at 60∘ with the normal to a glass-air and water-air interface respectively. Predict the angle of refraction of an incident ray in water at 45∘ with the normal to a water glass interface. Take .aμg=1.32.
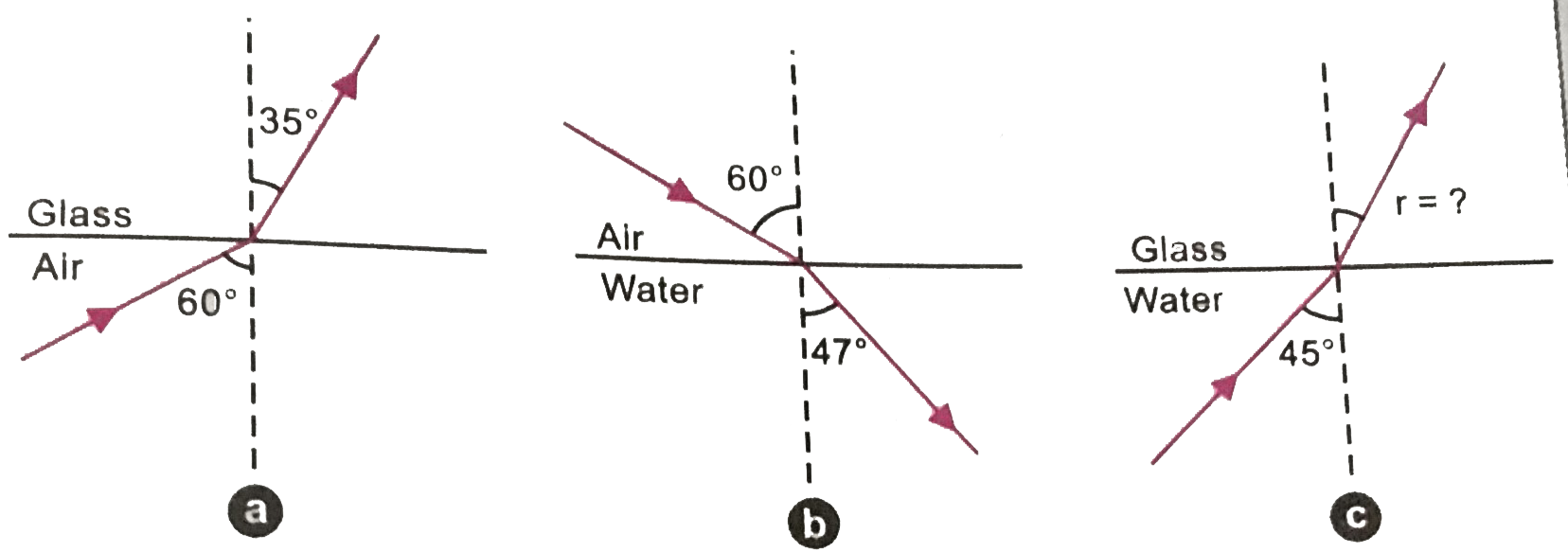 .
.
View SolutionIf the polarising angle for air glass interface is 56.3∘, what is the angle of refraction in glass ?
View SolutionThe refractive index of glass is 3/2. What is the polarising angle for glass
View SolutionAnswer the following:
(a) Define wavefront. Use Huygens' principle to verify the laws of refraction.
(b) How is linearly polarised light obtained by the process of scattering of light? Find the Brewster angle for air-glass interface, when the refractive index of glass is 1.5 .
View SolutionAn optical fibre (RI = 1.72) is surrounded by a glass coating (RI= 1.50). Find the critical angle for total internal reflection at the fibre-glass interface.
View Solution