Three particles of masses 8 kg, 4 kg and 4 kg situated at (4,1), (-2,2) and (1,-3) are acted upon by external forces 6¯jN,−6¯iN and 14¯iN. The acceleration of centre of mass of the system is
0.625ms−2
6.25ms−2
2.2ms−2
22ms−2
The correct Answer is:A
To find the acceleration of the center of mass of the system of three particles, we will follow these steps:
Step 1: Identify the masses and positions of the particles
We have three particles with the following properties:
- Particle 1: Mass m1=8kg at position (4,1)
- Particle 2: Mass m2=4kg at position (−2,2)
- Particle 3: Mass m3=4kg at position (1,−3)
Step 2: Identify the forces acting on each particle
The external forces acting on the particles are:
- Force on Particle 1: →F1=6^jN
- Force on Particle 2: →F2=−6^iN
- Force on Particle 3: →F3=14^iN
Step 3: Calculate the acceleration of each particle
Using Newton's second law, the acceleration →a of each particle can be calculated as:
→ai=→Fimi
- For Particle 1:
→a1=6^j8=34^jm/s2
- For Particle 2:
→a2=−6^i4=−32^im/s2
- For Particle 3:
→a3=14^i4=72^im/s2
Step 4: Calculate the total mass of the system
The total mass M of the system is:
M=m1+m2+m3=8+4+4=16kg
Step 5: Calculate the acceleration of the center of mass
The acceleration of the center of mass →acm is given by:
→acm=m1→a1+m2→a2+m3→a3M
Substituting the values:
→acm=8(34^j)+4(−32^i)+4(72^i)16
Calculating each term:
- For Particle 1:
8⋅34^j=6^j
- For Particle 2:
4⋅−32^i=−6^i
- For Particle 3:
4⋅72^i=14^i
Now, summing these:
→acm=(−6^i+14^i+6^j)16=(8^i+6^j)16
This simplifies to:
→acm=12^i+38^jm/s2
Step 6: Calculate the magnitude of the acceleration of the center of mass
The magnitude |→acm| is calculated as:
|→acm|=√(12)2+(38)2
Calculating each term:
(12)2=14,(38)2=964
Finding a common denominator (64):
14=1664
Thus,
|→acm|=√1664+964=√2564=58m/s2
Final Answer
The acceleration of the center of mass of the system is:
58m/s2or0.625m/s2
We have three particles with the following properties:
- Particle 1: Mass m1=8kg at position (4,1)
- Particle 2: Mass m2=4kg at position (−2,2)
- Particle 3: Mass m3=4kg at position (1,−3)
The external forces acting on the particles are:
- Force on Particle 1: →F1=6^jN
- Force on Particle 2: →F2=−6^iN
- Force on Particle 3: →F3=14^iN
Using Newton's second law, the acceleration →a of each particle can be calculated as:
→ai=→Fimi
→a1=6^j8=34^jm/s2
→a2=−6^i4=−32^im/s2
→a3=14^i4=72^im/s2
The total mass M of the system is:
M=m1+m2+m3=8+4+4=16kg
The acceleration of the center of mass →acm is given by:
→acm=m1→a1+m2→a2+m3→a3M
→acm=8(34^j)+4(−32^i)+4(72^i)16
- For Particle 1:
8⋅34^j=6^j
4⋅−32^i=−6^i
4⋅72^i=14^i
→acm=(−6^i+14^i+6^j)16=(8^i+6^j)16
→acm=12^i+38^jm/s2
The magnitude |→acm| is calculated as:
|→acm|=√(12)2+(38)2
Calculating each term:
(12)2=14,(38)2=964
Finding a common denominator (64):
14=1664
Thus,
|→acm|=√1664+964=√2564=58m/s2
The acceleration of the center of mass of the system is:
58m/s2or0.625m/s2
Similar Questions
Two particles of masses 2 kg and 4 kg are approaching each other with acceleration 1ms−2 and 2ms−2, respectively, on a smooth horizontal surface. Find the acceleration of center of mass of the system.
View SolutionA body of mass 10 kg is acted upon by two perpendicular forces , 6 N and 8 N . The resultant acceleration of the body is
View Solution
Knowledge Check
A body of mass 10kg is acted upon by two per pendicular forces 6N and 8N. The resultant ac-celeration of the body is .
A1ms−2 at angle of tan−1(34)w.r.t.8NforceB0.2ms−2 at angle of tan−1(34)w.r.t.8NforceC1ms−2 at angle of tan−1(43)w.r.t.8NforceD0.2ms−2 at angle of tan−1(43)w.r.t.8Nforce
Three point particles of masses 1.0 kg 2 kg and 3 kg are placed at three comers of a right angle triangle of sides 4.0 cm , 3.0 cm and 5.0 cm as shown in the figure . The centre of mass of the system is at point :
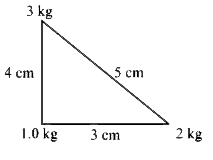
View SolutionThree point particles of masses 1.0 kg 2 kg and 3 kg are placed at three comers of a right angle triangle of sides 4.0 cm , 3.0 cm and 5.0 cm as shown in the figure . The centre of mass of the system is at point :

View SolutionThree particles of masses 1kg,2kg and 3kg are situated at the corners of an equilateral triangle move at speed 6ms−1,3ms−1 and 2ms−1 respectively. Each particle maintains a direction towards the particles at the next corners symmetrically. Find velocity of CM of the system at this instant
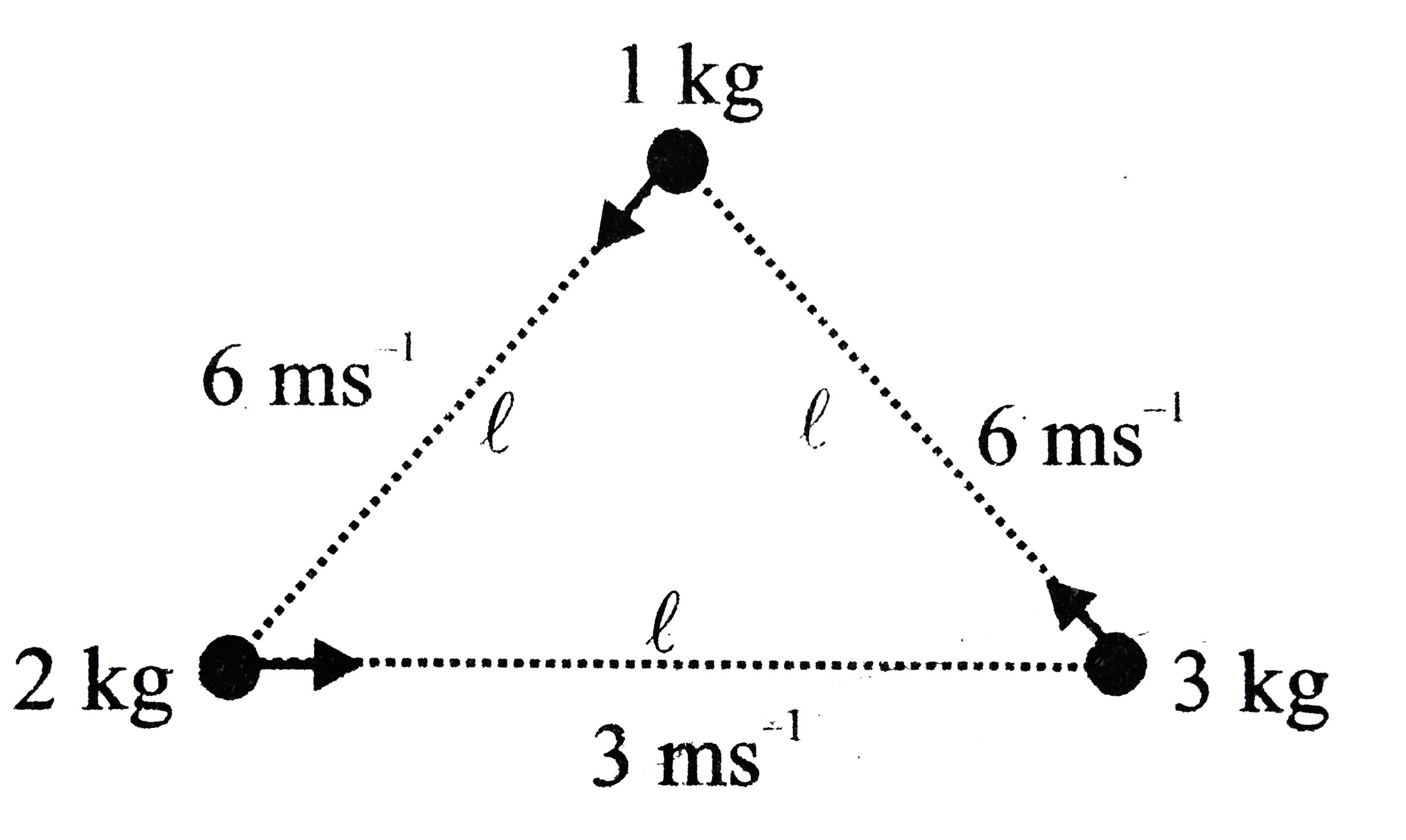
View SolutionTwo blocks of masses 6kg and 4kg are attached to the two ends of a massless string passing over a smooth fixed pulley. if the system is released, the acceleration of the centre of mass of the system will be
View SolutionParticles of masses 1 kg and 3 kg at (2i+5j+13k) m and (−6i+4j−2k)m then instantaneous position of their centre of mass is
View SolutionParticles of masses 1kg and 2kg are at (i+2j+3k) m and (−2i+4j+2k)m then instantaneous position of their centre of mass is
View SolutionThree particles of masses 1.0 kg 2.0 kg and 3.0 kg are placed at the corners A,B and C respectively of an equilateral triangle ABC of edge 1m. Locate the centre of mass of the system.
View Solution