Find the stretch in the springs shown in figure. The respective data are given in the figure. The friction and masses in pulleys are negligible. Find the extension in spring?

a) As mass M is in equilibrium, the tension in the string with which it is hanging will be Mg. As pulley is massless the tension in the upper string must be half of the lower string, Mg/2. The stretch in the spring must be such that the restoring force in the string is equal to the tension in it. Thus we have
k1x=Mg2orx1=Mg2k1
(b) Here again mass M is in equilibrium, thus the tension in the string connected to it must be equal to Mg and hence the restoring force in the lower spring will also be Mg. If x1 be the extension in this spring, we have
k1x1=Mgorx1=Mgk1
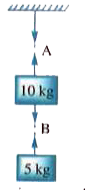
As pulley is light, the tension in upper string must be twice that of the lower srting, 2Mg which is equal to restoring force in the upper spring. If x1 is the extension in the upper spring, we have
k2x2=Mgorx2=2Mgk2
Wherever a body is in equilibrium, due to inertia it is maintained in a state of rest. If any of the supporting force a body is removed, body start from rest with some acceleration in the direction opposite to the force applied by the support, as all other forces acting on body will have a resultant in that direction.
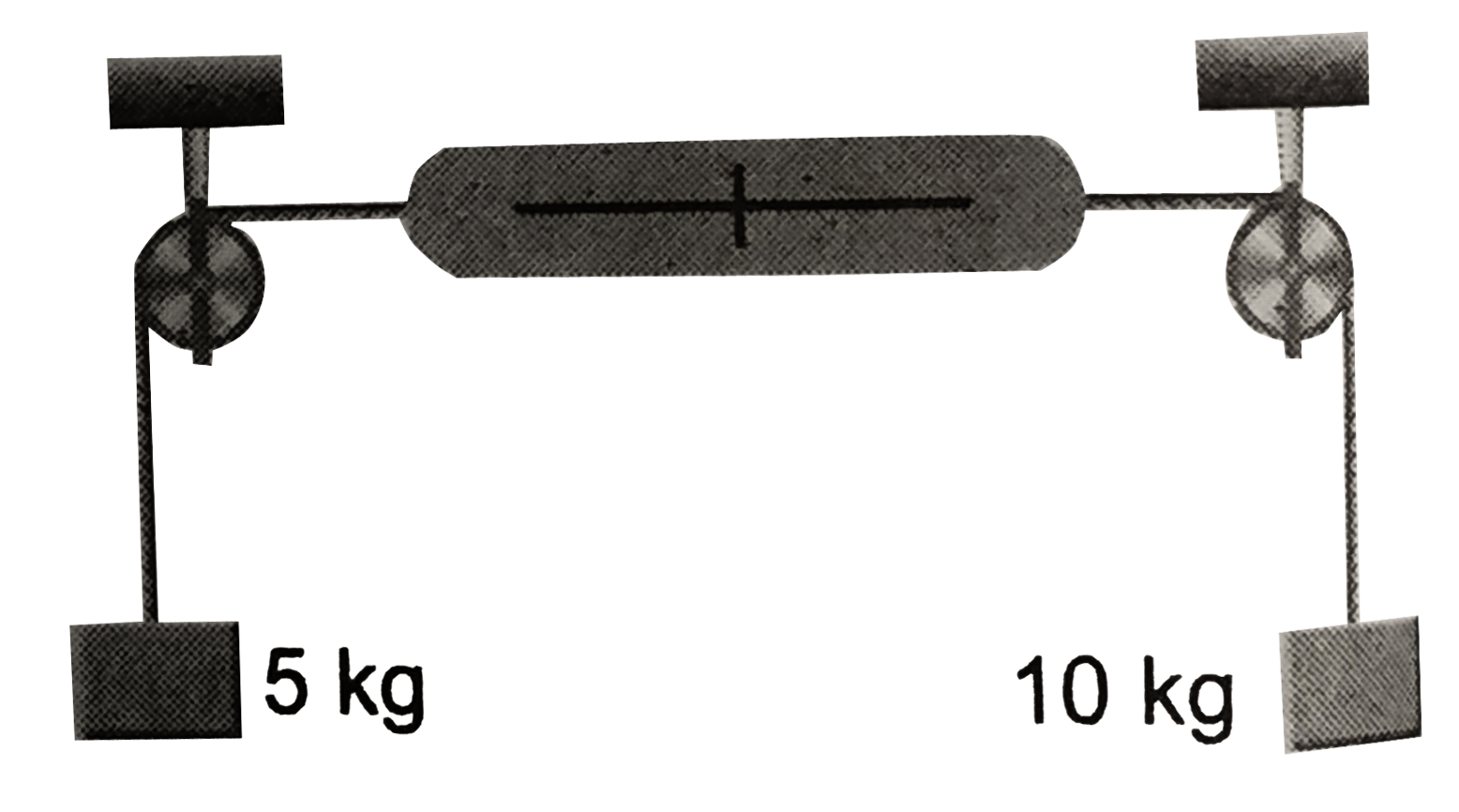
We discuss the situation with an example shown in figure. Two masses 5 kg and 10 kg are hanging in equilibrium with the strings A and B. If tensions in the strings A and B are T1andT2 respectively, then we have
T1=5g=50N,T2=15g=150N
If at some instant we break string B. tension T1 becomes zero instantane-ously and block will start falling from rest under gravity and 10 kg block, as it is still in equilibrium, T2 will instantly change to 10 N to balance its weight.
If instead of string B, we break the string A, tension T2 becomes zero and both the blocks will start falling with acceleration g.
Now T1 is just an internal force for the system of two blocks and as there is no interaction between the two blocks and both are falling under gravity T1 will also instantly become zero.
Now to understand the concept in a better way we slightly modify the situation of previous example. Instead of strings, we use springs as shown in figure. Initially the blocks are in equilibrium . If spring B breaks, its tension will instantly become zero as we are using ideal springs (massless/ inertialess),but due to inertia initially 10kg mass will not move from its initial position, so tension in upper spring will not change instantly hence it will accelerate in upward direction with acceleration given as
a=150−10010=5m/s2
If spring A breaks tension in spring A will instantly become zero, but as due to inertia 5 kg and 10 kg blocks will start moving from rest. As initally just after breaking of spring A the two masses are at rest, tension in spring B will not change at this instant which is T = 5g =50 N,
Thus at this instant (just after spring A is cut)
acceleration of 10 kg mass is a1=10g+kx10=100+5010=15m/s2
and that of 5 kg mass is a2=5g−kx5=50−505=0m/sec2

Similar Questions
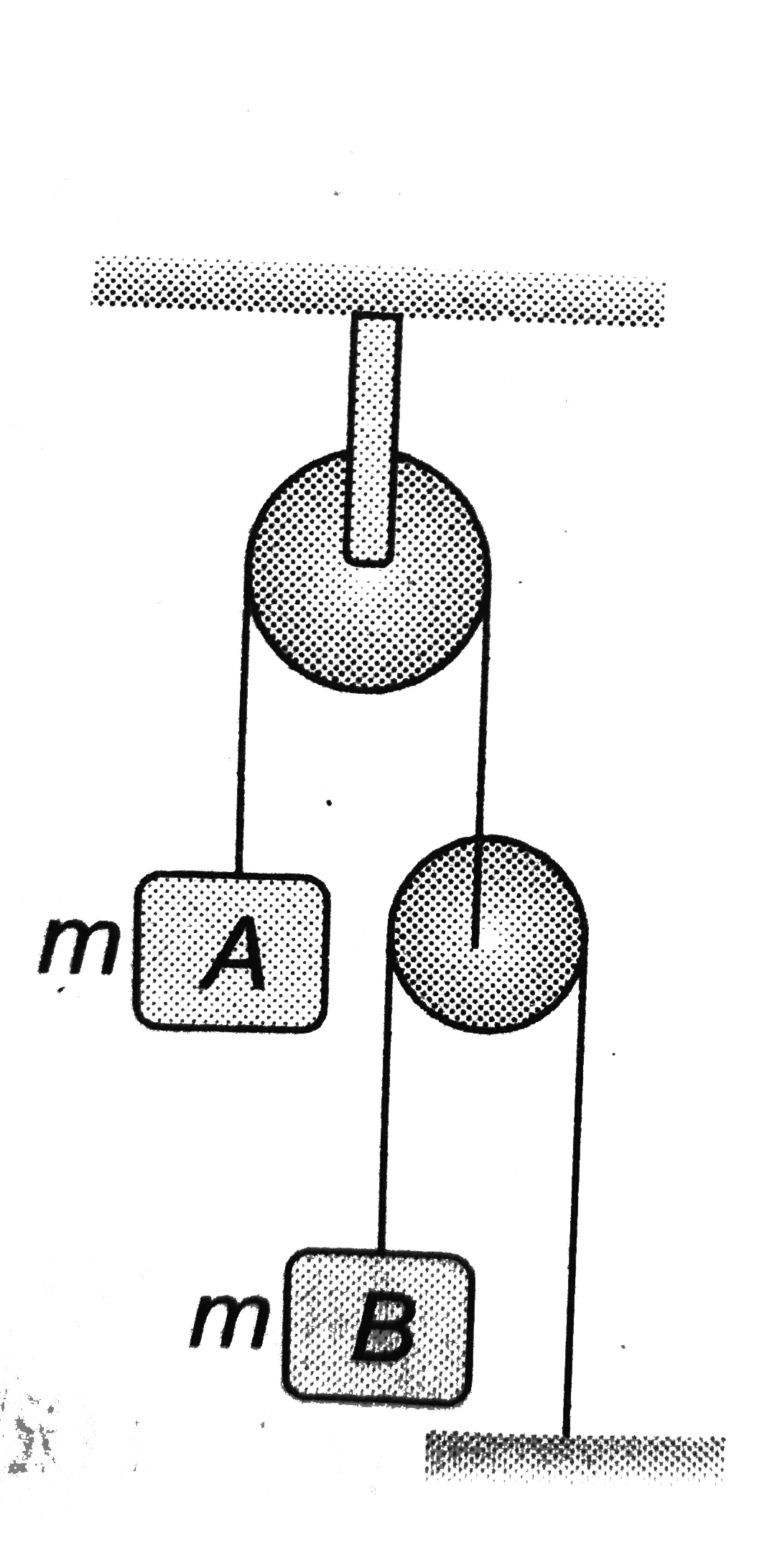
In the figure shown neglecting friction and mass of pulley, what is the acceleration of mass B ?
View SolutionIn the arrangement shown in the figure, the acceleration of the pulley is, (Ignore friction)

View SolutionA block of mass m is arranged on the wedge as shown in figure . The wedge angle is θ. If the masses of pulley and thread and negligible and friction is absent , find the acceleration of the wedge
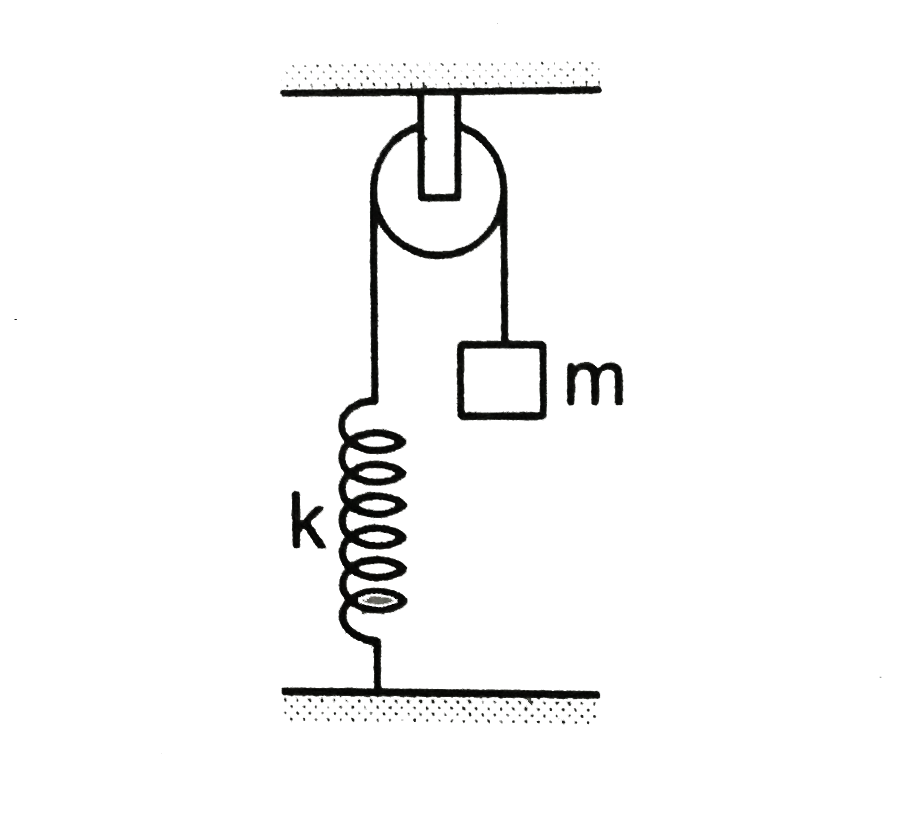
View SolutionThe string the spring and the puley shown in figure are light. Find the time period of the mass m.
View SolutionThree blocks of masses m1,m2andm3 are connected as shown in the figure. All the surfaces are frictionless and the string and the pulleys are light. Find the acceleration of m1
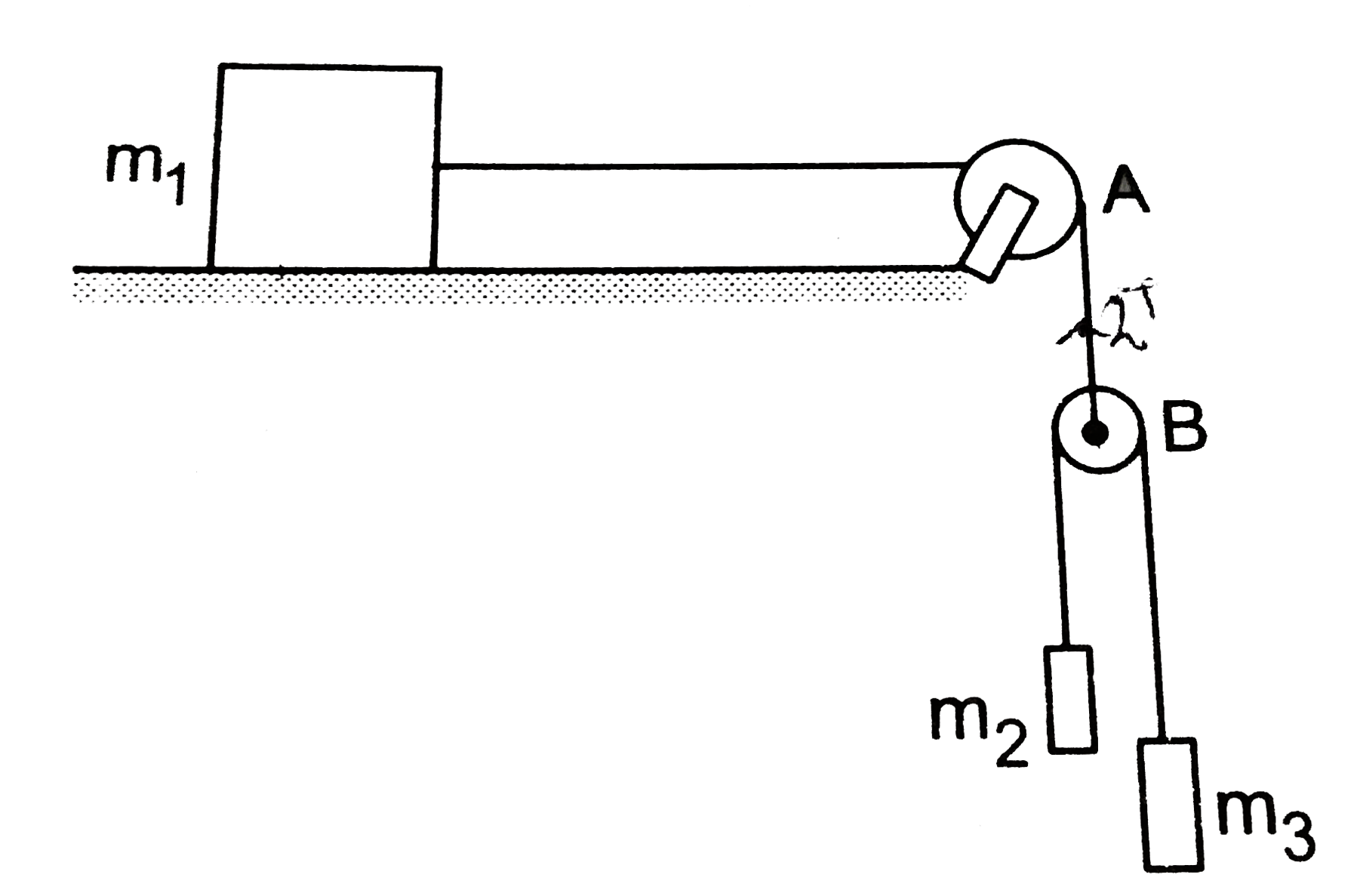
View SolutionFind the ratio of the extension in upper spring to lower spring
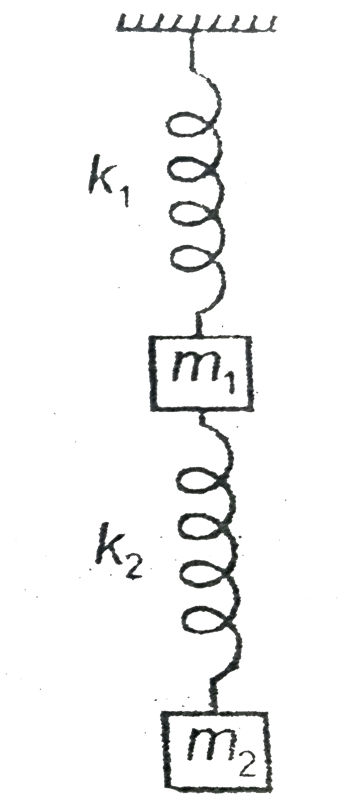
View Solution
For the given dimensions shown in figure, find critical value of coefficient of friction μ
View SolutionFind the maximum energy stored in the spring shown in figure, for which the block remains stationary on the rough horizontal surface.

View SolutionFor the arrangement shown in the figure, the reading of spring balance is
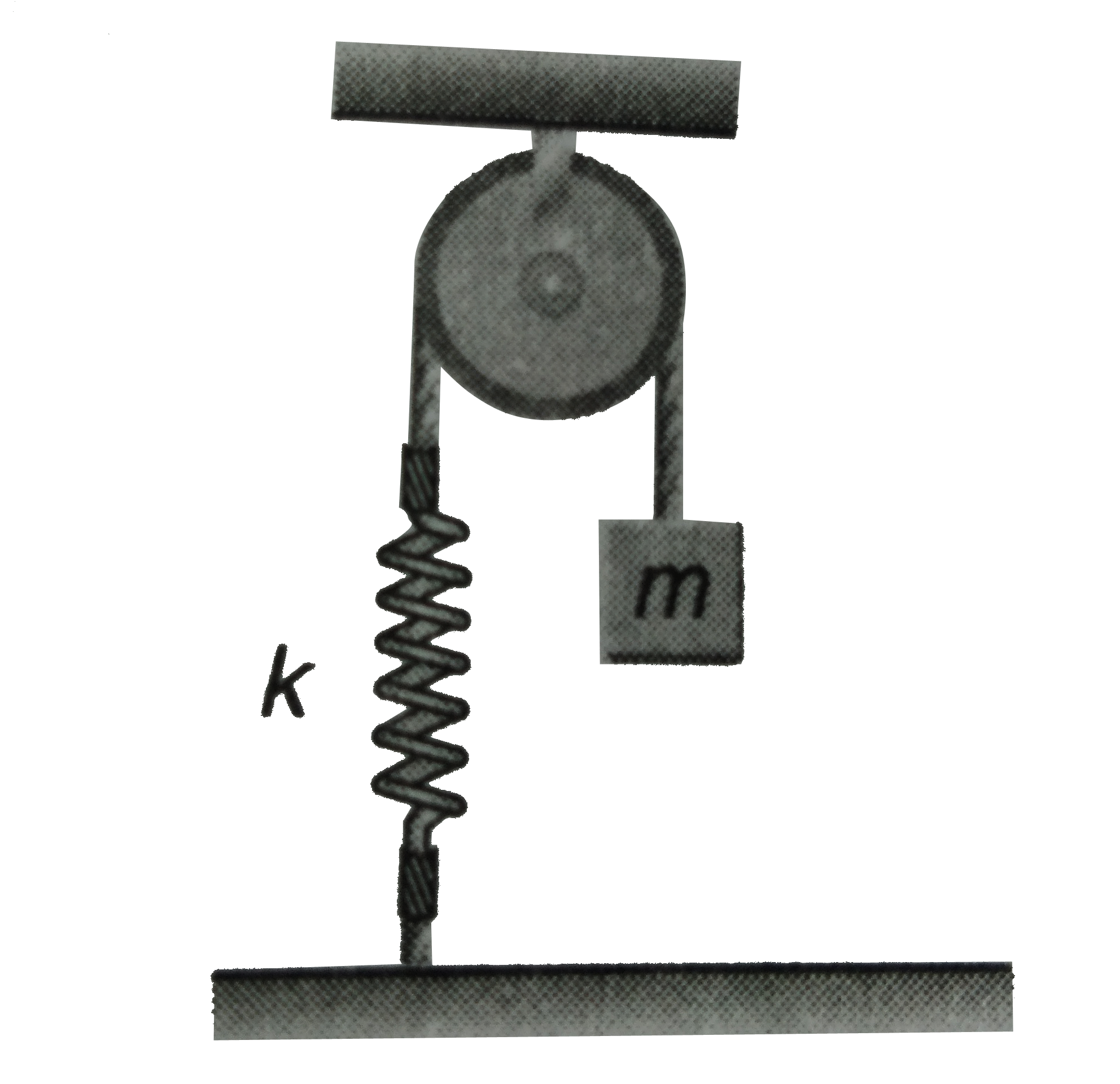
View SolutionThe string, spring and the pulley shown in figure are light. Find the time period of the mass m.
View Solution
