A force of (4x2+3x)N acts on a particle which displaces it from x=2m to x=3m. The work done by the force is
32.8 J
3.28 J
0.328J
zero
The correct Answer is:A
To find the work done by the force F=(4x2+3x)N when a particle is displaced from x=2m to x=3m, we will use the formula for work done, which is given by the integral of force over displacement:
W=∫x2x1Fdx
1. Identify the Force Function:
The force acting on the particle is given as:
F(x)=4x2+3x
2. Set the Limits of Integration:
The particle is displaced from x1=2m to x2=3m.
3. Write the Integral for Work Done:
The work done by the force as the particle moves from x=2 to x=3 is:
W=∫32(4x2+3x)dx
4. Calculate the Integral:
We will compute the integral:
W=∫(4x2+3x)dx
The integral can be solved term by term:
∫4x2dx=4x33+C
∫3xdx=3x22+C
Therefore, the integral becomes:
W=(4x33+3x22)32
5. Evaluate the Integral at the Limits:
Now we will evaluate this expression from x=2 to x=3:
W=(4(3)33+3(3)22)−(4(2)33+3(2)22)
Calculating the first part:
=4⋅273+3⋅92=36+13.5=49.5
Now calculating the second part:
=4⋅83+3⋅42=323+6=323+183=503
6. Combine the Results:
Now, we can combine the results:
W=49.5−503
Converting 49.5 to a fraction:
49.5=992
Finding a common denominator (which is 6):
W=992−1006
Converting 992 to sixths:
992=2976
Thus,
W=2976−1006=1976≈32.83J
Final Answer:
The work done by the force is approximately:
W≈32.83J
The force acting on the particle is given as:
F(x)=4x2+3x
The particle is displaced from x1=2m to x2=3m.
The work done by the force as the particle moves from x=2 to x=3 is:
W=∫32(4x2+3x)dx
We will compute the integral:
W=∫(4x2+3x)dx
The integral can be solved term by term:
∫4x2dx=4x33+C
∫3xdx=3x22+C
Therefore, the integral becomes:
W=(4x33+3x22)32
Now we will evaluate this expression from x=2 to x=3:
W=(4(3)33+3(3)22)−(4(2)33+3(2)22)
=4⋅273+3⋅92=36+13.5=49.5
=4⋅83+3⋅42=323+6=323+183=503
Now, we can combine the results:
W=49.5−503
Converting 49.5 to a fraction:
49.5=992
Finding a common denominator (which is 6):
W=992−1006
Converting 992 to sixths:
992=2976
Thus,
W=2976−1006=1976≈32.83J
The work done by the force is approximately:
W≈32.83J
Similar Questions
Forces of magnitudes 5 and 3 units acting in the directions 6ˆi+2ˆj+3ˆkand3ˆi−2ˆi+6ˆk respectively act on a particle which is displaced from the point ( 2,2,-1) to ( 4,3,1) . The work done by the forces, is
View SolutionForces of magnitudes 5 and 3 units acting in the directions 6ˆi+2ˆj+3ˆkand3ˆi−ˆi+6ˆk respectively act on a particle which is displaced from the point ( 2,2,-1) to ( 4,3,1) . The work done by the forces, is
View Solution
Knowledge Check
A force F acting on an object varies with distance x as shown in the figure. The work done by the force in moving the object from x=0 to x = 20 m is
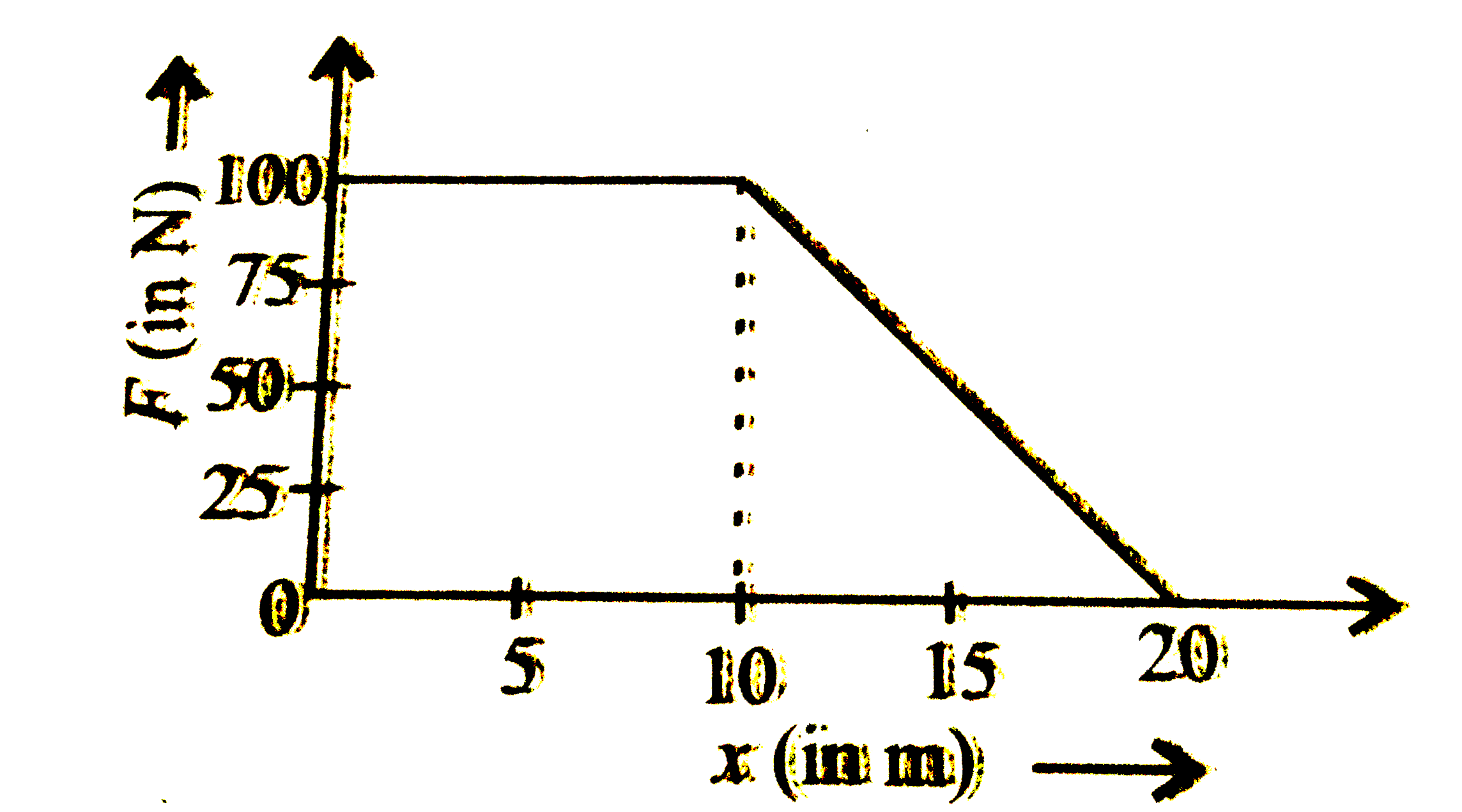 A500 JB1000 JC1500 JD2000 J
A500 JB1000 JC1500 JD2000 J
Consider a variable force F=(3x+5)N acting on a body and if it is displaced from x=2m to x=4m, calculate the work done by this force.
View SolutionA positon dependent force ,F=8−4x+3x2N acts on a small body of mass 2 kg and displaces it from x=0 to x=5 m. The work done in joule is
View SolutionA force f=10+2x acts on a particle moving in straight line on x axis the work done by this force during a displacement from x=0 and x=3m
View SolutionA force (3ˆi+4ˆj) newton acts on a boby and displaces it by (2ˆi+3ˆj) metre. The work done by the force is
View SolutionA force→F=(7−2x+3x2) N is applied on a 2 kg mass which displaces it from x = 0 to x = 5 m. Work done in joule is -
View SolutionA force F=(2+x) acts on a particle in x-direction where F is in newton and x in meter. Find the work done by this force during a displacement from 1. 0 m to x = 2.0 m.
View SolutionA force F=(2+x) acts on a particle in x-direction where F is in newton and x in metre. Find the work done by this force during a displacement form 1. 0 m to x = 2.0 m.
View Solution
