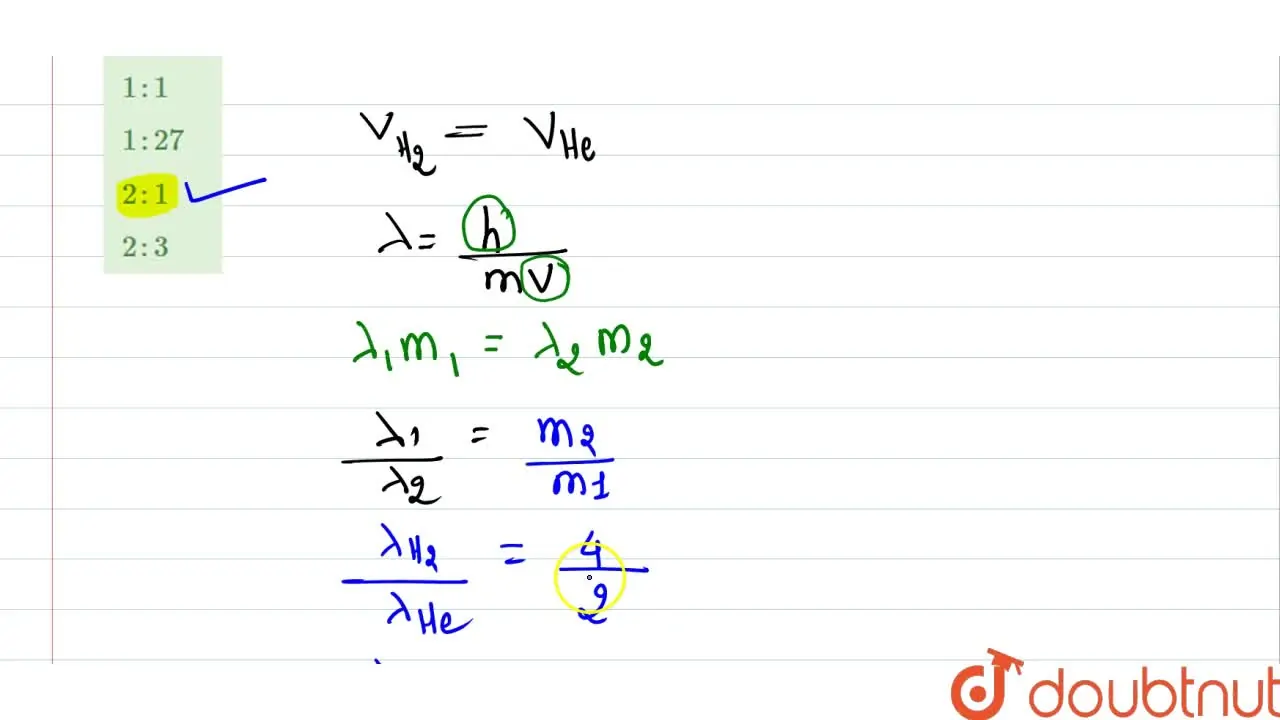
A hydrogen molecule and helium atom are moving with the same velocity. Then the ratio of their de Broglie wavelength is
1:1
1:27
2:1
2:3
The correct Answer is:C
Step by step video, text & image solution for A hydrogen molecule and helium atom are moving with the same velocity. Then the ratio of their de Broglie wavelength is by Chemistry experts to help you in doubts & scoring excellent marks in Class 11 exams.
Similar Questions
The de Broglie wavelength is given by
View SolutionIf particles are moving with same velocity , then maximum de - Broglie wavelength will be for
View SolutionAn α-particle and a proton are fired through the same magnetic field which is perpendicular to their velocity vectors. The α-partcles and the proton move such that radius of curvature of their paths is same. Find the ratio of their de Broglie wavelengths.
View SolutionConsider the four gases hydrogen, oxygen, nitrogen and helium at the same temperature. Arrange them in the increasing order of the de Broglie wavelengths of their molecules.
View SolutionA deuteron and an α - partical have same kinetic energy. Find the ratio of their de-Broglie wavelengths.
View SolutionWhen the velocity of an electron increases, its de Broglie wavelength
View SolutionIf an alpha particle and a deuteron move with velocity v and 2v respectively, the ratio of their de-Broglie wave length will be ……….
View Solutionde-Broglie wavelength applies only to
View SolutionAn electron and a proton have same kinetic energy Ratio of their respective de-Broglie wavelength is about
View SolutionA material particle with a rest mass mo is moving with a velocity of light c. Then, the wavelength of the de Broglie wave associated with it is
View Solution