Benzene diazonium chloride (A) decoposes into chlorobenzene (B) and N2 (g) in first order reaction. Volumes of N2 collected after 5 min and at the complete decomposition of A are 10 mL. and 50mL respectively. The are constant for the reaction is
0.446min−1
0.0446min−1
0.223min−1
0.112min(−1)
The correct Answer is:B
To find the rate constant for the decomposition of benzene diazonium chloride into chlorobenzene and nitrogen gas, we will follow these steps:
Step 1: Understand the Reaction
The reaction can be summarized as:
C6H5N2Cl→C6H5Cl+N2
This is a first-order reaction.
Step 2: Gather Initial and Final Volumes
From the problem:
- Initial volume of benzene diazonium chloride (A) = 50 mL (at t=0)
- Volume of N2 collected after 5 minutes = 10 mL
- Volume of N2 at complete decomposition = 50 mL
Step 3: Calculate Remaining Volume of A after 5 Minutes
After 5 minutes, the volume of benzene diazonium chloride remaining can be calculated as:
Volume of A remaining=Initial volume−Volume of N2 collected
Volume of A remaining=50mL−10mL=40mL
Step 4: Use the First-Order Rate Equation
For a first-order reaction, the rate constant k can be calculated using the formula:
k=2.303tlog(A0At)
where:
- A0 = initial volume of A = 50 mL
- At = volume of A at time t = 40 mL
- t = time = 5 minutes
Step 5: Substitute Values into the Equation
Substituting the values into the equation:
k=2.3035log(5040)
Step 6: Calculate the Logarithm
Calculating the logarithm:
log(5040)=log(1.25)
Using logarithm values:
log(1.25)≈0.0969
Step 7: Calculate the Rate Constant
Now substituting back into the equation:
k=2.3035×0.0969
k≈2.303×0.09695
k≈0.2235
k≈0.0446min−1
Final Answer
The rate constant k for the reaction is approximately 0.0446min−1.
---
The reaction can be summarized as:
C6H5N2Cl→C6H5Cl+N2
This is a first-order reaction.
From the problem:
- Initial volume of benzene diazonium chloride (A) = 50 mL (at t=0)
- Volume of N2 collected after 5 minutes = 10 mL
- Volume of N2 at complete decomposition = 50 mL
After 5 minutes, the volume of benzene diazonium chloride remaining can be calculated as:
Volume of A remaining=Initial volume−Volume of N2 collected
Volume of A remaining=50mL−10mL=40mL
For a first-order reaction, the rate constant k can be calculated using the formula:
k=2.303tlog(A0At)
where:
- A0 = initial volume of A = 50 mL
- At = volume of A at time t = 40 mL
- t = time = 5 minutes
Substituting the values into the equation:
k=2.3035log(5040)
Calculating the logarithm:
log(5040)=log(1.25)
Using logarithm values:
log(1.25)≈0.0969
Now substituting back into the equation:
k=2.3035×0.0969
k≈2.303×0.09695
k≈0.2235
k≈0.0446min−1
The rate constant k for the reaction is approximately 0.0446min−1.
Similar Questions
A first order reaction is 20% complete in 10 min. What is the rate constant?
View SolutionDecomposition of NH4NO2(aq into N2(g) and 2H2O(l) is first order reaction.
View SolutionA first order reaction is 20% complete in 10min. Calculate (a) the specific rate constant of the reaction and (b) the time taken for the reaction to reach 75% completion.
View SolutionA first order reaction is 50% complete in 30 min at 27∘C. Calculate the rate constant of the reaction at 27∘C.
View SolutionThe half -lie period for the decomposition of AB2(g) at 100mm pressure is 10min and at 200mm pressure is 5 min, the order of reaction is
View SolutionFor the first order reaction. N2O5(g)→N2O4(g)+12O2(g), the volume of O2 produced is 15 mL and 40 mL after 8 minutes and at the end of the reaction respectively. The rate constant is equal to
View Solution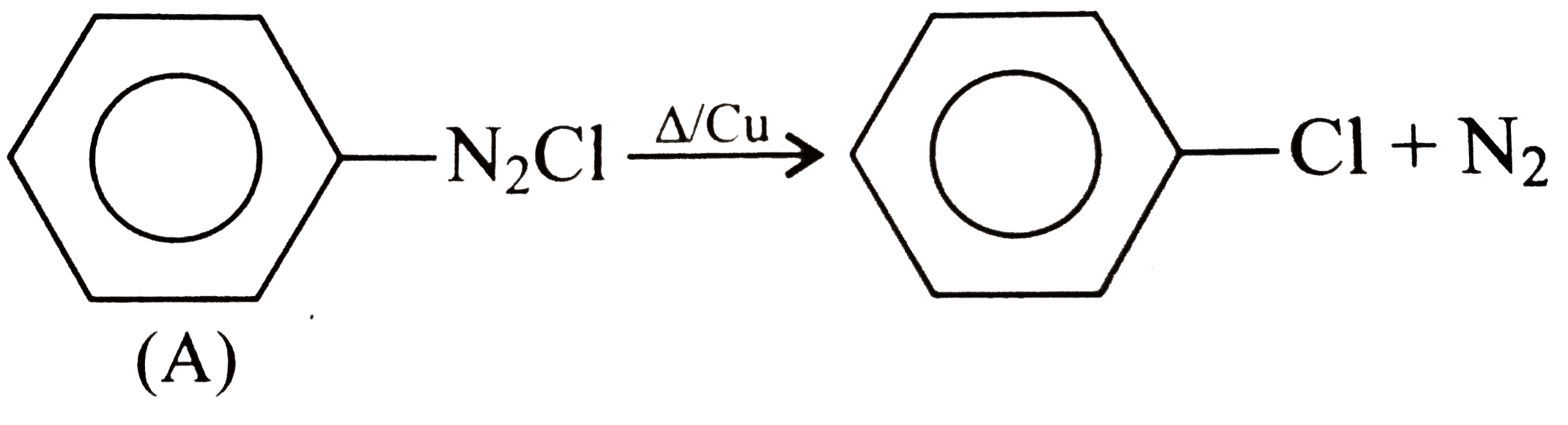
Half life id independent of the concentration of A. After 10min volume of N2 gas is 10L and after complete reaction is 50L. Hence, the rate constant is
View SolutionFor the first order reaction A(g)→2B(g)+C(g), the initial pressure is PA=90mHg, the pressure after 10 minutes is found to be 180mmHg. The rate constant of the reaction is
View SolutionTime required to decompose SO2Cl2 to half of its intial amount is 60min. If the decomposition is a first order reaction, calculate the rate constant of the reaction.
View SolutionThe half life of decompoistion of N2O5 is a first order reaction represented by N2O5→N2O4+1/2O2
After 15min the volume of O2 profuced is 9ml and at the end of the reaction 35ml. The rate constant is equal to
View Solution
