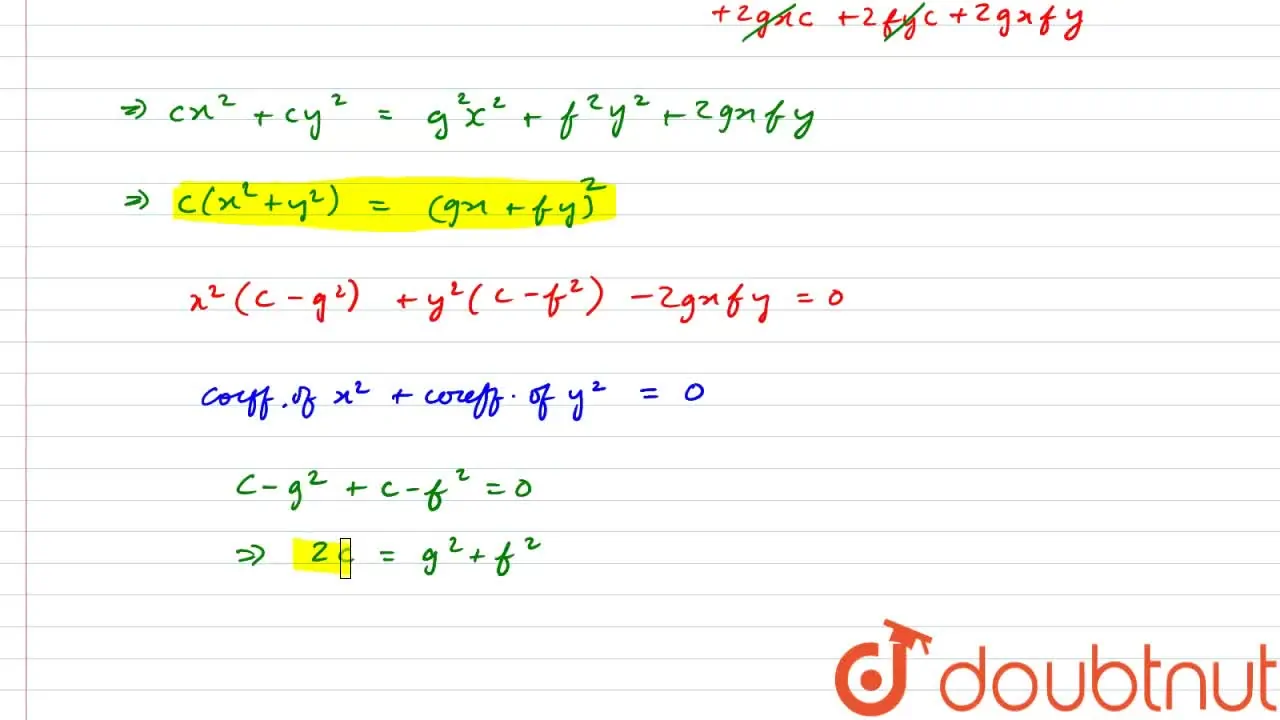
Find the pair of tangents from the origin
to the circle x2+y2+2gx+2fy+c=0
and hence deduce a condition for these
tangents to be perpendicular.
The correct Answer is:2c
Step by step video & image solution for Find the pair of tangents from the origin to the circle x^(2) + y^(2) + 2gx + 2fy + c = 0 and hence deduce a condition for these tangents to be perpendicular. by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Similar Questions
The pair of tangents from origin to the circle x2+y2+4x+2y+3=0 is
View SolutionFind the pair of tangents from origin to x2+y2+4x+2y+3=0
View SolutionFind the equation of the two tangents from the point (0,1) to the circle x2+y2−2x+4y=0
View SolutionThe equation of tangents drawn from the origin to the circlex2+y2−2rx−2hy+h2=0
View SolutionThe angle between the pair of tangents from the point (1,12) to the circle x2+y2+4x+2y−4=0 is
View SolutionFind the angle between the two tangents from the origin to the circle (x−7)2+(y+1)2=25
View SolutionFind the angle between the tangents
drawn from (3,2) to the circle
x2+y2−6x+4y−2=0View SolutionFind the angle between the pair of
tangents drawn from (1,3) to the circle
x2+y2−2x+4y−11=0View SolutionFind the centre and radius of the circle ax2+ay2+2gx+2fy+c=0 where a≠0.
View SolutionA pair of tangents are drawn from the origin to the circle x2+y2+20(x+y)+20=0 . Then find its equations.
View Solution